All Courses
Get the App Signup / Login
Sign in Open App
Class 7 Exam  >  Class 7 Tests  >  Mathematics (Maths) Class 7  >  Olympiad Test: Comparing Quantities - Class 7 MCQ

Olympiad Test: Comparing Quantities - Class 7 MCQ


Test Description

20 Questions MCQ Test Mathematics (Maths) Class 7 - Olympiad Test: Comparing Quantities

Olympiad Test: Comparing Quantities for Class 7 2024 is part of Mathematics (Maths) Class 7 preparation. The Olympiad Test: Comparing Quantities questions and answers have been prepared according to the Class 7 exam syllabus.The Olympiad Test: Comparing Quantities MCQs are made for Class 7 2024 Exam. Find important definitions, questions, notes, meanings, examples, exercises, MCQs and online tests for Olympiad Test: Comparing Quantities below.
Solutions of Olympiad Test: Comparing Quantities questions in English are available as part of our Mathematics (Maths) Class 7 for Class 7 & Olympiad Test: Comparing Quantities solutions in Hindi for Mathematics (Maths) Class 7 course. Download more important topics, notes, lectures and mock test series for Class 7 Exam by signing up for free. Attempt Olympiad Test: Comparing Quantities | 20 questions in 30 minutes | Mock test for Class 7 preparation | Free important questions MCQ to study Mathematics (Maths) Class 7 for Class 7 Exam | Download free PDF with solutions
Olympiad Test: Comparing Quantities - Question 1
Save

In a computer lab, there are 3 computers for every 6 students. How many computers will be needed for 24 students?

Detailed Solution for Olympiad Test: Comparing Quantities - Question 1

3 computers used by 6 students 

1 computer = 6/3 student 

1 computer is used by 2 students 

therefore computers needed are half of number of students
24/2 = 12 computers 

Olympiad Test: Comparing Quantities - Question 2
Save

Out of 32 students, 8 are absent. What percent of the students are present?

Detailed Solution for Olympiad Test: Comparing Quantities - Question 2

No. of students that are present
=32-8=24
Percentage of absent students =24/32*100=75%

1 Crore+ students have signed up on EduRev. Have you? Download the App
Olympiad Test: Comparing Quantities - Question 3
Save

There are 25 radios, 16 of them are out of order. What percent of radios are out of order?

Detailed Solution for Olympiad Test: Comparing Quantities - Question 3

No. of radios out of order=16
Percentage of radios out of order=16/25*100=64%

Olympiad Test: Comparing Quantities - Question 4
Save

A shop has 500 parts, out of which 5 are defective. What percent are not defective?
Detailed Solution for Olympiad Test: Comparing Quantities - Question 4

%defective = 5/500 x 100 = 1%

so %not defective = 100 - 1 =99%

Olympiad Test: Comparing Quantities - Question 5
Save

There are 120 voters, 90 of them voted yes. What percent voted yes?
Detailed Solution for Olympiad Test: Comparing Quantities - Question 5

90/120 x 100 %
3/4 x 100 %
75 %

Olympiad Test: Comparing Quantities - Question 6
Save

A survey of 40 children showed that 25% liked playing football. How many children not liked playing football?
Detailed Solution for Olympiad Test: Comparing Quantities - Question 6

Total number of children=40
25% like football
Children who don’t like playing football=100-25=75%
So no. of children who don’t like football=75% of 40
= 75/100 * 40 = 30 children

Olympiad Test: Comparing Quantities - Question 7
Save

8% children of a class of 25 like getting wet in the rain. How many children do not like getting wet in the rain.
Detailed Solution for Olympiad Test: Comparing Quantities - Question 7

Number of children that like getting wet in rain = 8% of 25
= 8/100 × 25
= 8/4
= 2 children.
Number of children that don't like getting wet in rain
= 25 - 2
= 23 children
Therefore, there are 23 such students that don't like getting wet in rain.
 

Olympiad Test: Comparing Quantities - Question 8
Save

Rahul bought a sweater and saved Rs 20 when a discount of 25% was given. What was the price of the sweater before the discount?
Detailed Solution for Olympiad Test: Comparing Quantities - Question 8

discount=25%
25% of x =20
25/100*x=20
25x=2000
x=2000/25=Rs. 80

Olympiad Test: Comparing Quantities - Question 9
Save

Out of 15,000 voters in a constituency, 60% voted. Find the number of voters who did not vote.
Olympiad Test: Comparing Quantities - Question 10
Save

Meeta saves Rs 400 from her salary. If this is 10% of her salary. What is her salary?
Detailed Solution for Olympiad Test: Comparing Quantities - Question 10

400 is the 10% of the salary
10% of x = 400
10/100*x = 400
x=400*10 = Rs. 4000

Olympiad Test: Comparing Quantities - Question 11
Save

A local cricket team played 20 matches in one season. It won 25% of them. How many matches did they lose?
Detailed Solution for Olympiad Test: Comparing Quantities - Question 11

Percentage of lost matches=75%
No. of matches lost=75% of 20
=75/100*20
=15 matches

Olympiad Test: Comparing Quantities - Question 12
Save

A school team won 6 games this year against 4 games won last year. What is the percent increase?
Olympiad Test: Comparing Quantities - Question 13
Save

The number of illiterate persons in a country decreased from 150 lakhs to 100 lakhs in 10 years. What is the percentage of decrease?
Detailed Solution for Olympiad Test: Comparing Quantities - Question 13

Percentage of decrease = change in illiterate people/150*100 =(150-100)*100/150=50*100/150=100/3%

Olympiad Test: Comparing Quantities - Question 14
Save

Cost of an item is Rs 50. It was sold with a profit of 12%. Find the selling price.
Olympiad Test: Comparing Quantities - Question 15
Save

How much will an item cost if 10% discount is given on the marked price Rs 100?
Detailed Solution for Olympiad Test: Comparing Quantities - Question 15

Olympiad Test: Comparing Quantities - Question 16
Save

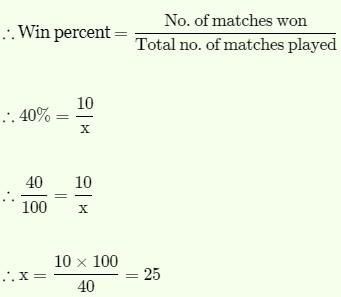
A football team won 10 matches out of the total number of matches they played. If their win percentage was 40, then how many matches did they play in all?
Detailed Solution for Olympiad Test: Comparing Quantities - Question 16

Olympiad Test: Comparing Quantities - Question 17
Save

If Chameli had Rs 600 left after spending 75% of her money, how much did she have in the beginning?
Detailed Solution for Olympiad Test: Comparing Quantities - Question 17

After spending 75% of money , chameli has Rs. 600.
So 600=25% of the money
25% of x=600
25/100*x=600
x=600*4=Rs. 2400

Olympiad Test: Comparing Quantities - Question 18
Save

The price of a scooter was Rs 34,000 last year. It has increased by 20% this year. What is the price now?
Detailed Solution for Olympiad Test: Comparing Quantities - Question 18

Price of the scooter last year=34000
Increase=20%
Amount of increase=20% of 34000=20/100*34000=6800
New price=34000+6800=Rs. 40800

Olympiad Test: Comparing Quantities - Question 19
Save

The price of a scooter was Rs 34,000 last year. It has decreased by 5% this year. What is the price now?
Detailed Solution for Olympiad Test: Comparing Quantities - Question 19

Olympiad Test: Comparing Quantities - Question 20
Save

Mohit bought a CD for Rs. 750 and sold it Rs. 875. Find his gain or loss percent.
76 videos|345 docs|39 tests
Join Course
Information about Olympiad Test: Comparing Quantities Page
In this test you can find the Exam questions for Olympiad Test: Comparing Quantities solved & explained in the simplest way possible. Besides giving Questions and answers for Olympiad Test: Comparing Quantities, EduRev gives you an ample number of Online tests for practice

Top Courses for Class 7

76 videos|345 docs|39 tests
Join Course
Download as PDF

Top Courses for Class 7

Important Questions for Olympiad Test: Comparing Quantities

Find all the important questions for Olympiad Test: Comparing Quantities at EduRev.Get fully prepared for Olympiad Test: Comparing Quantities with EduRev's comprehensive question bank and test resources. Our platform offers a diverse range of question papers covering various topics within the Olympiad Test: Comparing Quantities syllabus. Whether you need to review specific subjects or assess your overall readiness, EduRev has you covered. The questions are designed to challenge you and help you gain confidence in tackling the actual exam. Maximize your chances of success by utilizing EduRev's extensive collection of Olympiad Test: Comparing Quantities resources.

Olympiad Test: Comparing Quantities MCQs with Answers

Prepare for the Olympiad Test: Comparing Quantities within the Class 7 exam with comprehensive MCQs and answers at EduRev. Our platform offers a wide range of practice papers, question papers, and mock tests to familiarize you with the exam pattern and syllabus. Access the best books, study materials, and notes curated by toppers to enhance your preparation. Stay updated with the exam date and receive expert preparation tips and paper analysis. Visit EduRev's official website today and access a wealth of videos and coaching resources to excel in your exam.

Online Tests for Olympiad Test: Comparing Quantities Mathematics (Maths) Class 7

Practice with a wide array of question papers that follow the exam pattern and syllabus. Our platform offers a user-friendly interface, allowing you to track your progress and identify areas for improvement. Access detailed solutions and explanations for each test to enhance your understanding of concepts. With EduRev's Online Tests, you can build confidence, boost your performance, and ace Olympiad Test: Comparing Quantities with ease. Join thousands of successful students who have benefited from our trusted online resources.
© EduRev
Education Revolution
Follow Us
Signup to see your scores go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup