Test: Evens And Odds- 2 - GMAT MCQ
10 Questions MCQ Test Quantitative for GMAT - Test: Evens And Odds- 2
If p and q are integers and p + q + p is odd, which of the following must be odd?
If a , b, and c are integers and ab2 / c is a positive even integer, which of the following must be true?
I. ab is even
II. ab > 0
III. c is even
I. ab is even
II. ab > 0
III. c is even
If k and y are integers, and 10k + y is odd, which of the following must be true?
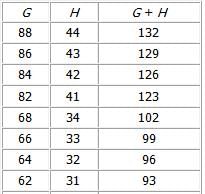
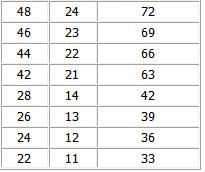
Each digit in the two-digit number G is halved to form a new two-digit number H. Which of the following
could be the sum of G and H?
If a is an even integer and b is an odd integer, which of the following cannot be an even integer?
If x and y are prime integers and x < y, which of the following cannot be true?
If q, r, and s are consecutive even integers and q < r < s, which of the following CANNOT be the value of s2 – r2 – q2?
For all positive integers m, (m) = 3m when m is odd and (m) = ½ m when m is even, which of the following is equivalent to (9)*(6)?
If a is an even integer and b is an odd integer, which of the following cannot be an even integer?
If x and y are prime integers and x < y, which of the following cannot be true?
|
127 videos|154 docs|111 tests
|