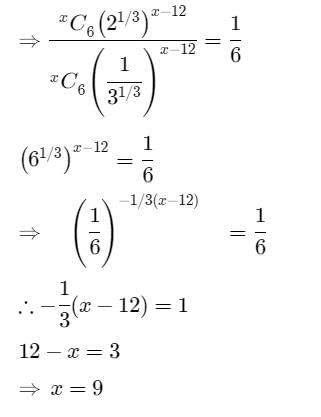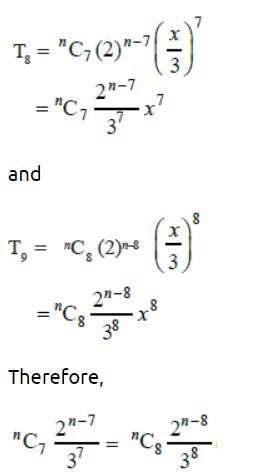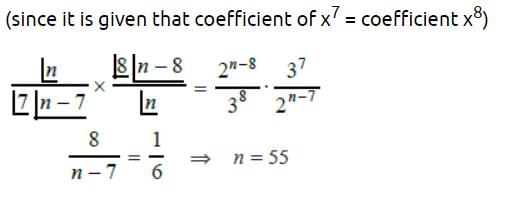JEE Advanced Level Test: Binomial Numbers- 1 - JEE MCQ
20 Questions MCQ Test Chapter-wise Tests for JEE Main & Advanced - JEE Advanced Level Test: Binomial Numbers- 1
If 'a' be the sum of the odd terms & 'b' the sum of the even terms in the expansion of (1+x)n, then (1–x2)n equals
Given that the term of the expansion (x1/3 – x-1/2)15 which does not contain x is 5m where m ∈ N, then m equals
In the binomial (21/3 + 3-1/3)n, if the ratio of the seventh term from the beginning of the expansion to the seventh term from its end is 1/6, then n equals
If the coefficients of x7 & x8 in the expansion of are equal, then the value of n is
The expression is a polynomial in x of degree
Number of rational terms in the expansion of is
The sum of the binomial coefficients of is equal to 256. The constant term in the expansion is
The sum of the co-efficients in the expansion of (1 – 2x + 5x2)n is 'a' and the sum of the co-efficients in the expansion of (1 + x)2n is b. Then
The number of terms in the expansion of (x + y)n is
The greatest terms of the expansion (2x + 5y)13 when x = 10, y = 2 is
The binomial expansion of , n ∈ N contains a term independent of x
Let where n ∈ N and p ∈ N and 0 < f < 1 then the value, f2 – f + pf – p is
If (1 + x + x2)25 = a0 + a1x + a2x2 + .........+a50. x50 then a0 + a2 + a4 + ...........+ a50is
The co-efficient of x4 in the expansion of (1 – x + 2x2)12 is
Coefficient of at in the expansion of (a+p)m-1 + (a+p)m-2 (a+q) + (a+p)m-3 (a+q)2 + ....(a+q)m-1 where a > q and p > q is
Number of terms free from radical sign in the expansion of (1 + 31/3 + 71/7)10 is
In the expansion of (1 + x)n (1 + y)n (1 + z)n, the sum of the co-efficients of the terms of degree 'r' is
|
446 docs|930 tests
|