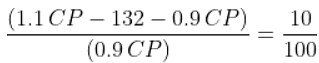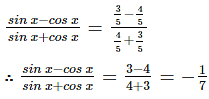SSC CGL (Tier II) Practice Test - 9 - SSC CGL MCQ
30 Questions MCQ Test SSC CGL Tier II Mock Test Series 2024 - SSC CGL (Tier II) Practice Test - 9
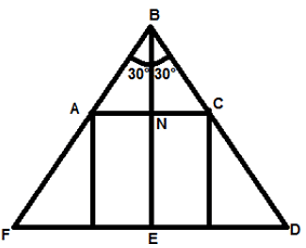
An equilateral triangle of side 12 cm is drawn. What is the area (in cm2) of the largest square which can be drawn inside it?
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
Directions: Study the graph carefully and answer the following question.
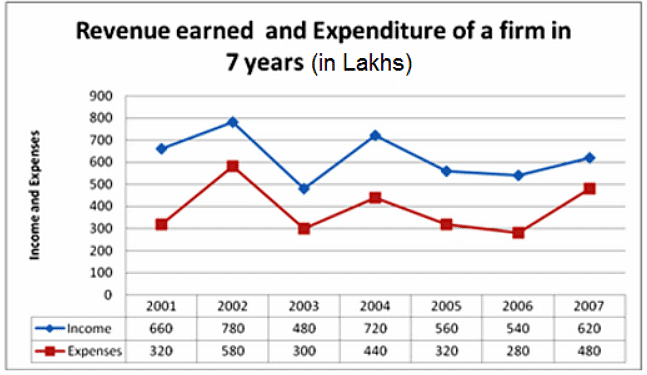
Q. In 2008, If the firm faces extra expense of the value Rs. 50 lakh, and earns Rs. 45 lakh more, then the approximate % change in the savings of the firm during the given years is

A flag of height 4 metres is standing on the top of a building. The angle of elevation of the top of the flag from a point X is 45° and the angle of elevation of the top of building from X is 30°. Point X is on the ground level. What is the height (in metres) of the building?
Each interior angle of a regular polygon is 120° greater than each exterior angle. How many sides are there in the polygon?
Arjun invested Rs. 2400 and Bhaskar invested Rs. 3600 in a startup. After the completion of four successful months of the startup, Keshav also invested Rs. X in this startup. After the completion of eight successful months of the startup, Bhaskar withdrew Rs. 600. When annual profit was calculated, it came out to be Rs. 22,500 and Keshav's share in this profit was Rs. 8000. What is the value of X?
Which of the following values of θ satisfies the given equation?
![]() = 4, where θ ∈ (0, π)
= 4, where θ ∈ (0, π)
Directions: In the following number series, one number is wrong. Find out the wrong number.
5, 8, 15, 30, 60, 124
A group of 12 men can complete a piece of work in 9 days. After 3 days, 6 men joined to replace 2 men. How many days would be required to complete the remaining work?
The digit in units place in the product 771 × 659 × 365 is
A photocopy machine copies 1,500 workbooks in 8 hours, while another machine takes 12 hours to do the same job. What is the total number of hours that both the machines working together will take to complete the 1,500 workbooks?
Ramesh travels by bus from city A to city B at an average speed of 44 km/hr. Suresh travels by taxi from city A to city B at an average speed of 77 km/hr and takes 3 hours less than the time taken by Ramesh. What is the distance (in km) between the two cities?
Directions: In the following number series one number is wrong. Find out the wrong number.
160, 161, 170, 195, 244, 324, 446
A person covered one-fourth of a distance at the speed of 2.5 kmph. He covered the remaining distance at the speed of 5 kmph. If he took half-an-hour for the entire journey, what distance did he travel?
A man sells an article at a gain of 10%. If he had bought it at 10% less and sold it for Rs. 132 less, he would have still gained 10%. The cost price of the article is
A person goes from A to B with speed 40 km/hr & return from B to A with speed 30 km/hr. Whole journey takes 14 hr, then find the distance between A & B in Km.
Rajib, Barun, and Anil engaged in work together. it was agreed that Rajib would invest Rs 4875 for 8 months, Barun Rs 8400 for 5 months, and Anil Rs 15000 for 2 months. Rajib wants to be the working member for which, he was to receive 5% of the profits. The total profit earned was Rs 7400. Calculate the share of Anil in the profit.
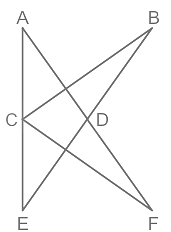
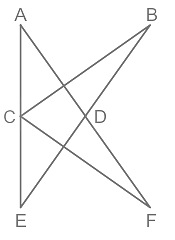
In the figure given below, ∠CBE = ∠AFC, AF = 12 cm, CB = 6 cm, BE = 12 cm and CF = 6 cm. If AC = 2 cm, then find the ratio of CE : AE.
The total cost of fencing the right-angled triangular park is Rs. 102,600. If the length of the smallest side is 20 m and the length of the second longest side is 28 m longer than the smallest side, then find the rate at which fencing is done per m. (in Rs per m)
A student of a school has to score 25% of marks to get throughout. If he gets 50 marks and fails by 25 marks, then find the maximum marks set for the examination?
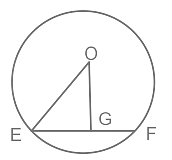
The perpendicular from the center O of the circle bisects the chord EF. If EF = 30 cm and the radius of the circle is 17 cm, then find the length of the perpendicular OG.
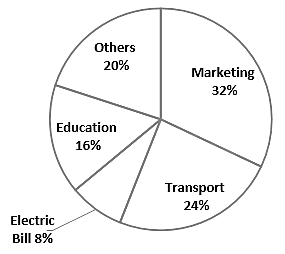
The pie chart represents an expenditure of a family in a month. If the difference between the expenditure on marketing and Transportation is Rs 2400. Then find the expenditure on transport, Electric Bill, and others together.
If 2b = a + c and y2 = zx then find (xb - c) (yc - a) (za - b)
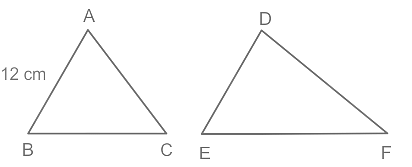
The perimeters of Δ ABC and Δ DEF are 36 cm and 54 cm respectively. AB = 12 cm. If both are similar triangles, find side DE.
If the compound interest earned for the 2nd year is Rs. 240 and the rate of interest applied is 20% per annum, then find the principal invested.
There are 35 mobile phones in a box, out of which the average weight of 12 mobile phones is 50 grams and the average weight of 11 mobile phones is 40 grams and the average weight of the remaining mobile phones is 45 grams, then find the average weight of all mobile phones. (approx.)
Rupam's age is 5/12 times Manju's age, and the HCF of the age of Rupam and Manju is 4. Find the difference between Manju's age and Rupam's age.
A certain amount of milk is added to the 150 liters of 3 : 7 milk-water solution. Find the quantity of the milk present in the final mixture, if the ratio of the milk and water in the final solution is 5 : 7?
X and Y can do a piece of work in 45 days and 40 days respectively. They begin to work together, but X leaves after n days and then Y completes the remaining work in 23 days. What is n equal to ?
|
66 docs|139 tests
|
|
66 docs|139 tests
|


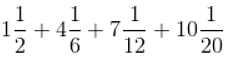 ... up to 20 terms?
... up to 20 terms?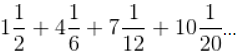 up to 20 terms
up to 20 terms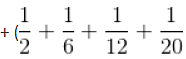 ...) up to 20 terms
...) up to 20 terms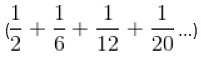

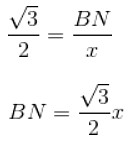
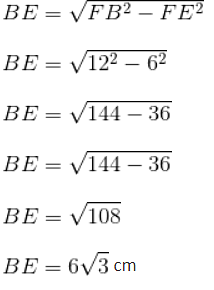
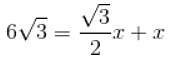
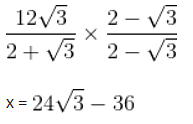
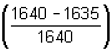 x 100 = 0.304878% = 0.31% Loss
x 100 = 0.304878% = 0.31% Loss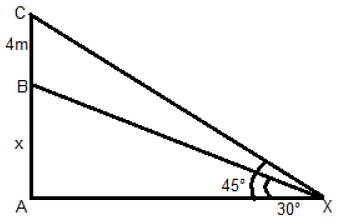
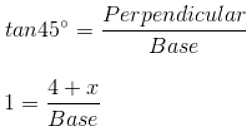
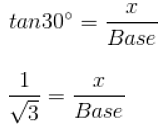
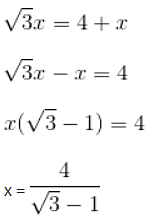
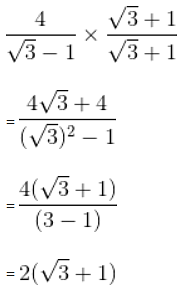
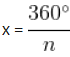
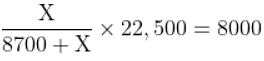
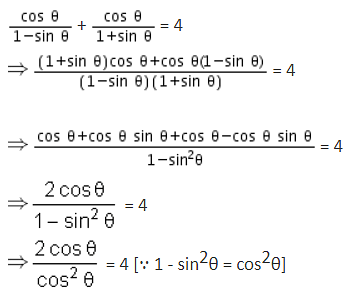
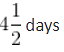
 workbooks in 1 hour.
workbooks in 1 hour.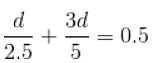
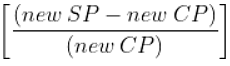 × 100 = profit %
× 100 = profit %