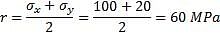Test: Principal Stress & Strain - 1 - Mechanical Engineering MCQ

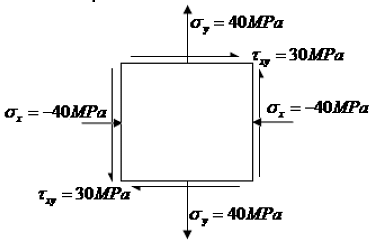
In a bi-axial stress problem, the stresses in x and y directions are (σ x = 200 MPa and σy =100 MPa. The maximum principal stress in MPa, is:

The normal stresses at a point are σx = 10 MPa and, σy = 2 MPa; the shear stress at this point is 4MPa. The maximum principal stress at this point is:

The state of stress at a point under plane stress condition is

σ_xx = 40 MPa , σ_yy = 100 MPa and τ_xy = 40 MPa .

The radius of the Mohr‟s circle representing the given state of stress in MPa is

When a component is subjected to axial stress the normal stress σ_n is maximum, if cos θ is _______ . (σ_n=σ_xCos²θ)

1. maximum
2. minimum
3. always one
4. always zero

If the two principal strains at a point are 1000 × 10^-6 and -600 × 10^-6, then the maximum shear strain is:

In the case of bi-axial state of normal stresses, the normal stress on 45° plane is equal to

In a two-dimensional problem, the state of pure shear at a point is characterized by

For the state of stress of pure shear τ the strain energy stored per unit volume in the elastic, homogeneous isotropic material having elastic constants E and ν will be:

Assertion (A): Circular shafts made of brittle material fail along a helicoidally surface inclined at 45° to the axis (artery point) when subjected to twisting moment.

Reason (R): The state of pure shear caused by torsion of the shaft is equivalent to one of tension at 45° to the shaft axis and equal compression in the perpendicular direction.

The state of plane stress in a plate of 100 mm thickness is given as σ_xx = 100 N/mm², σ_yy = 200 N/mm², Young's modulus = 300 N/mm², Poisson's ratio = 0.3. The stress developed in the direction of thickness is:

Consider the following statements:

State of stress in two dimensions at a point in a loaded component can be completely specified by indicating the normal and shear stresses on

1. A plane containing the point

2. Any two planes passing through the point

3. Two mutually perpendicular planes passing through the point

Of these statements

In a strained material one of the principal stresses is twice the other. The maximum shear stress in the same case is t_max . Then, what is the value of the maximum principle stress?

The principal stresses σ₁, σ₂ and σ₃ at a point respectively are 80 MPa, 30 MPa and –40 MPa. The maximum shear stress is:

Plane stress at a point in a body is defined by principal stresses 3σ and σ. The ratio of the normal stress to the maximum shear stresses on the plane of maximum shear stress is:

For the state of plane stress.
Shown the maximum and minimum principal stresses are:

Maximum shear stress in a Mohr's Circle

Two-dimensional state of stress at a point in a plane stressed element is represented by a Mohr circle of zero radius. Then both principal stresses