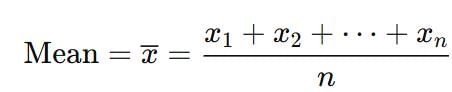What is the definition of an average?

The average, or mean, is calculated by adding all the values in a set and then dividing by the number of values.

 For Example, 

For the numbers 4, 8, and 10, 

The average is 
(4 + 8 + 10) / 3 

= 7.33.

How do you calculate the average of a set of numbers?

To calculate the average, sum all the numbers in the set and then divide the sum by the total count of numbers. 

For Example, 

The average of 2, 3, 5 is 

(2 + 3 + 5) / 3 

= 10 / 3 

= 3.33

If the average of five test scores is 80 and one score is 100, what is the average of the other four scores?

Let the sum of the five scores be S. Since the average is 80, 

S = 80 x 5 = 400. 

If one score is 100, the sum of the other four scores is 400 - 100 = 300. The average of the other four scores is 300 / 4 = 75

What happens to the average if a new number is added to a set?

If the new number is higher than the current average, the overall average will increase; 
if it is lower, the overall average will decrease.

A class has an average score of 90. If a new student joins and raises the average to 92, what is the score of the new student assuming there were 10 students initially?

Let the total score of the 10 students be T. 

Since the average is 90, 

T = 90 x 10 = 900

After the new student joins, 

The average becomes 92 for 11 students, 

So the new total score is

92 x 11 = 1012 

The new student's score is 1012 - 900 = 112.

What is the formula for calculating the average?

The formula for calculating the average (mean) is: 

For Example, 

For the values 5, 10, and 15, the average is 

= (5 + 10 + 15) / 3 

= 10.

If the average of a set of numbers is known, how can you find the total sum of the numbers?

If the average (A) of a set of n numbers is known, the total sum (S) can be calculated using the formula: 

S = A x n. 

For Example, 

If the average is 20 and there are 5 numbers, the Total Sum = 20 X 5 = 100.

A set of five numbers has an average of 60. If one more number is added, and the new average becomes 64, what is the value of the new number?

Let the sum of the original five numbers be S. 

Since the average is 60, 

S = 60 x 5 = 300. 

The new total sum for six numbers is 64 x 6 = 384. The new number is 

384 - 300 = 84.

In a group of 8 friends, the average age is 25. If one friend leaves and the average age becomes 26, what is the age of the friend who left?

Let the total age of the 8 friends be T. Since the average age is 25, 

T = 25 X 8 = 200. 

After one friend leaves, the total age for 7 friends is 26 x 7= 182. 

The age of the friend who left is 

200 - 182 = 18.

The average score of a student in 6 subjects is 75. If the student scores 90 in the seventh subject, what is the new average?

The total score for 6 subjects is 

75 x 6 = 450. 

With the seventh subject, the total score becomes 450 + 90 = 540. 

The new average is 

540 / 7 = 77.14

If the average of three numbers is 30 and two of the numbers are 20 and 40, what is the third number?

Let the three numbers be x, 20, and 40. 

The average is 

(x + 20 + 40) / 3 = 30. 

This simplifies to 

x + 60 = 90, 

so x = 90 - 60 = 30.