Class 12 Exam > Class 12 Questions > The focal length of convex lens is 10 cm. If ...
Start Learning for Free
The focal length of convex lens is 10 cm. If it is placed into a liquid of refractive index 1.75 find focal length ?
Verified Answer
The focal length of convex lens is 10 cm. If it is placed into a liqui...
The main issue with this question is that neither original environment’s refractive index n0, nor the lens’s material’s refractive index nlens are given. While we could assume n0 to be air, nlens has a very serious impact on the end result.
From the Lensmaker’s formula I could derive the formula for the ratio of focal lengths incorporating minimum of variables. Deriving it I’ll leave as an exercise for the reader.
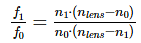
From this formula, knowing that f0=0.2m and n1=1.25, then assuming n lens=1.5 (approx. typical window glass) and n0=1 (air) we could calculate that the resulting f1 would be equal to 50 cm:
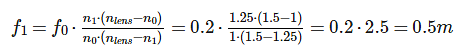
 This question is part of UPSC exam. View all Class 12 courses
This question is part of UPSC exam. View all Class 12 courses
Most Upvoted Answer
The focal length of convex lens is 10 cm. If it is placed into a liqui...
Focal Length of Convex Lens in Air
The focal length of a convex lens is a measure of its ability to converge or diverge light. In air, the focal length is given by the formula:
1/f = (n - 1) * (1/R1 - 1/R2)
Where:
- f is the focal length in air
- n is the refractive index of the lens material
- R1 is the radius of curvature of the first surface of the lens
- R2 is the radius of curvature of the second surface of the lens
In this case, the focal length of the convex lens in air is given as 10 cm.
Finding the Refractive Index of the Liquid
To find the focal length of the lens when it is placed into a liquid with a refractive index of 1.75, we can rearrange the formula and solve for f:
f = (n - 1) * (1/R1 - 1/R2)
Since the lens is placed into a liquid, we need to consider the refractive index of the liquid. Let's assume that the radius of curvature of both surfaces of the lens remains the same. Substitute the given values into the formula:
f = (1.75 - 1) * (1/R1 - 1/R2)
Now, let's calculate the new focal length.
Calculating the New Focal Length
Using the given refractive index of the liquid (n = 1.75), we can calculate the new focal length of the lens.
f = (1.75 - 1) * (1/R1 - 1/R2)
Since we don't have specific values for the radii of curvature of the lens surfaces, we cannot calculate the exact value of the new focal length. However, we can determine the relationship between the original focal length and the new focal length.
Relationship between Focal Lengths
The relationship between the focal lengths in air (f) and in the liquid (f') can be expressed as:
f' = nf
Where:
- f' is the new focal length of the lens in the liquid
- n is the refractive index of the liquid
- f is the original focal length of the lens in air
Substituting the given values into the equation, we have:
f' = 1.75 * 10 cm
Therefore, the new focal length of the convex lens when placed in the liquid with a refractive index of 1.75 is 17.5 cm.
The focal length of a convex lens is a measure of its ability to converge or diverge light. In air, the focal length is given by the formula:
1/f = (n - 1) * (1/R1 - 1/R2)
Where:
- f is the focal length in air
- n is the refractive index of the lens material
- R1 is the radius of curvature of the first surface of the lens
- R2 is the radius of curvature of the second surface of the lens
In this case, the focal length of the convex lens in air is given as 10 cm.
Finding the Refractive Index of the Liquid
To find the focal length of the lens when it is placed into a liquid with a refractive index of 1.75, we can rearrange the formula and solve for f:
f = (n - 1) * (1/R1 - 1/R2)
Since the lens is placed into a liquid, we need to consider the refractive index of the liquid. Let's assume that the radius of curvature of both surfaces of the lens remains the same. Substitute the given values into the formula:
f = (1.75 - 1) * (1/R1 - 1/R2)
Now, let's calculate the new focal length.
Calculating the New Focal Length
Using the given refractive index of the liquid (n = 1.75), we can calculate the new focal length of the lens.
f = (1.75 - 1) * (1/R1 - 1/R2)
Since we don't have specific values for the radii of curvature of the lens surfaces, we cannot calculate the exact value of the new focal length. However, we can determine the relationship between the original focal length and the new focal length.
Relationship between Focal Lengths
The relationship between the focal lengths in air (f) and in the liquid (f') can be expressed as:
f' = nf
Where:
- f' is the new focal length of the lens in the liquid
- n is the refractive index of the liquid
- f is the original focal length of the lens in air
Substituting the given values into the equation, we have:
f' = 1.75 * 10 cm
Therefore, the new focal length of the convex lens when placed in the liquid with a refractive index of 1.75 is 17.5 cm.

|
Explore Courses for Class 12 exam
|

|
Similar Class 12 Doubts
The focal length of convex lens is 10 cm. If it is placed into a liquid of refractive index 1.75 find focal length ?
Question Description
The focal length of convex lens is 10 cm. If it is placed into a liquid of refractive index 1.75 find focal length ? for Class 12 2024 is part of Class 12 preparation. The Question and answers have been prepared according to the Class 12 exam syllabus. Information about The focal length of convex lens is 10 cm. If it is placed into a liquid of refractive index 1.75 find focal length ? covers all topics & solutions for Class 12 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for The focal length of convex lens is 10 cm. If it is placed into a liquid of refractive index 1.75 find focal length ?.
The focal length of convex lens is 10 cm. If it is placed into a liquid of refractive index 1.75 find focal length ? for Class 12 2024 is part of Class 12 preparation. The Question and answers have been prepared according to the Class 12 exam syllabus. Information about The focal length of convex lens is 10 cm. If it is placed into a liquid of refractive index 1.75 find focal length ? covers all topics & solutions for Class 12 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for The focal length of convex lens is 10 cm. If it is placed into a liquid of refractive index 1.75 find focal length ?.
Solutions for The focal length of convex lens is 10 cm. If it is placed into a liquid of refractive index 1.75 find focal length ? in English & in Hindi are available as part of our courses for Class 12.
Download more important topics, notes, lectures and mock test series for Class 12 Exam by signing up for free.
Here you can find the meaning of The focal length of convex lens is 10 cm. If it is placed into a liquid of refractive index 1.75 find focal length ? defined & explained in the simplest way possible. Besides giving the explanation of
The focal length of convex lens is 10 cm. If it is placed into a liquid of refractive index 1.75 find focal length ?, a detailed solution for The focal length of convex lens is 10 cm. If it is placed into a liquid of refractive index 1.75 find focal length ? has been provided alongside types of The focal length of convex lens is 10 cm. If it is placed into a liquid of refractive index 1.75 find focal length ? theory, EduRev gives you an
ample number of questions to practice The focal length of convex lens is 10 cm. If it is placed into a liquid of refractive index 1.75 find focal length ? tests, examples and also practice Class 12 tests.

|
Explore Courses for Class 12 exam
|

|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.



















