B Com Exam > B Com Questions > There are two series with 5000 lottery ticket...
Start Learning for Free
There are two series with 5000 lottery tickets in each .There is only one price of rupees 3000 and tickets are sold at rupees 2 each .A person buys two tickets . What will be his expected gain if he buys :- a) one ticket of each series, B) tickets of same series?
Most Upvoted Answer
There are two series with 5000 lottery tickets in each .There is only ...
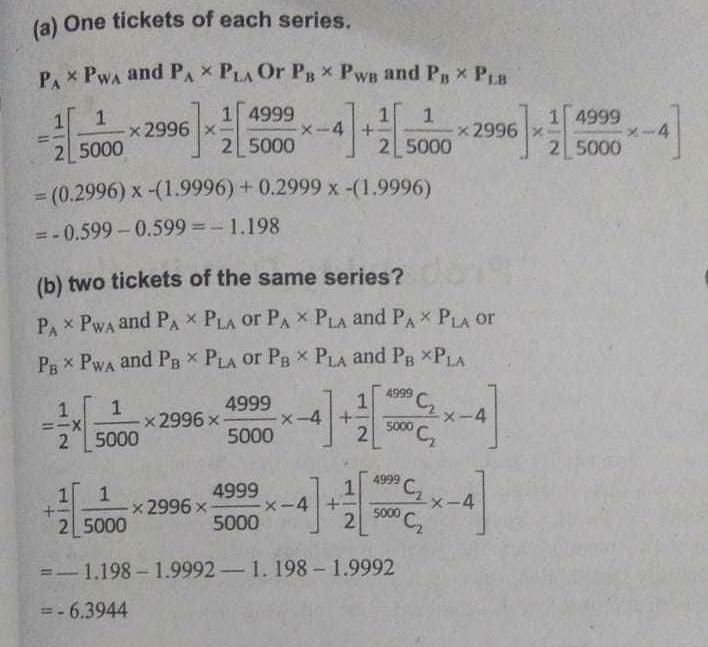
Community Answer
There are two series with 5000 lottery tickets in each .There is only ...
Scenario
There are two series with 5000 lottery tickets in each. There is only one prize of rupees 3000, and tickets are sold at rupees 2 each. A person buys two tickets. What will be his expected gain if he buys:
- One ticket of each series
- Tickets of the same series
Expected Gain Calculation
One Ticket of Each Series
There are a total of 10,000 tickets (5000 in each series). The probability of winning the prize is 1/10,000. Therefore, the expected gain can be calculated as:
Expected gain = (Probability of winning x Prize money) - Cost of tickets
Expected gain = (1/10,000 x 3000) - (2+2) = -0.6 rupees
Therefore, the expected gain of buying one ticket of each series is negative. It means that, on average, the person will lose 0.6 rupees for every two tickets bought.
Tickets of the Same Series
If the person buys two tickets of the same series, the probability of winning the prize increases to 2/5000 or 1/2500.
Expected gain = (Probability of winning x Prize money) - Cost of tickets
Expected gain = (1/2500 x 3000) - (2+2) = 0.2 rupees
Therefore, the expected gain of buying two tickets of the same series is positive. It means that, on average, the person will gain 0.2 rupees for every two tickets bought.
Conclusion
Based on the above calculations, it is recommended that the person should buy two tickets of the same series to maximize their expected gain. However, it is important to note that lottery is a game of chance, and there is no guarantee of winning or losing.

|
Explore Courses for B Com exam
|

|
Similar B Com Doubts
There are two series with 5000 lottery tickets in each .There is only one price of rupees 3000 and tickets are sold at rupees 2 each .A person buys two tickets . What will be his expected gain if he buys :- a) one ticket of each series, B) tickets of same series?
Question Description
There are two series with 5000 lottery tickets in each .There is only one price of rupees 3000 and tickets are sold at rupees 2 each .A person buys two tickets . What will be his expected gain if he buys :- a) one ticket of each series, B) tickets of same series? for B Com 2025 is part of B Com preparation. The Question and answers have been prepared according to the B Com exam syllabus. Information about There are two series with 5000 lottery tickets in each .There is only one price of rupees 3000 and tickets are sold at rupees 2 each .A person buys two tickets . What will be his expected gain if he buys :- a) one ticket of each series, B) tickets of same series? covers all topics & solutions for B Com 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for There are two series with 5000 lottery tickets in each .There is only one price of rupees 3000 and tickets are sold at rupees 2 each .A person buys two tickets . What will be his expected gain if he buys :- a) one ticket of each series, B) tickets of same series?.
There are two series with 5000 lottery tickets in each .There is only one price of rupees 3000 and tickets are sold at rupees 2 each .A person buys two tickets . What will be his expected gain if he buys :- a) one ticket of each series, B) tickets of same series? for B Com 2025 is part of B Com preparation. The Question and answers have been prepared according to the B Com exam syllabus. Information about There are two series with 5000 lottery tickets in each .There is only one price of rupees 3000 and tickets are sold at rupees 2 each .A person buys two tickets . What will be his expected gain if he buys :- a) one ticket of each series, B) tickets of same series? covers all topics & solutions for B Com 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for There are two series with 5000 lottery tickets in each .There is only one price of rupees 3000 and tickets are sold at rupees 2 each .A person buys two tickets . What will be his expected gain if he buys :- a) one ticket of each series, B) tickets of same series?.
Solutions for There are two series with 5000 lottery tickets in each .There is only one price of rupees 3000 and tickets are sold at rupees 2 each .A person buys two tickets . What will be his expected gain if he buys :- a) one ticket of each series, B) tickets of same series? in English & in Hindi are available as part of our courses for B Com.
Download more important topics, notes, lectures and mock test series for B Com Exam by signing up for free.
Here you can find the meaning of There are two series with 5000 lottery tickets in each .There is only one price of rupees 3000 and tickets are sold at rupees 2 each .A person buys two tickets . What will be his expected gain if he buys :- a) one ticket of each series, B) tickets of same series? defined & explained in the simplest way possible. Besides giving the explanation of
There are two series with 5000 lottery tickets in each .There is only one price of rupees 3000 and tickets are sold at rupees 2 each .A person buys two tickets . What will be his expected gain if he buys :- a) one ticket of each series, B) tickets of same series?, a detailed solution for There are two series with 5000 lottery tickets in each .There is only one price of rupees 3000 and tickets are sold at rupees 2 each .A person buys two tickets . What will be his expected gain if he buys :- a) one ticket of each series, B) tickets of same series? has been provided alongside types of There are two series with 5000 lottery tickets in each .There is only one price of rupees 3000 and tickets are sold at rupees 2 each .A person buys two tickets . What will be his expected gain if he buys :- a) one ticket of each series, B) tickets of same series? theory, EduRev gives you an
ample number of questions to practice There are two series with 5000 lottery tickets in each .There is only one price of rupees 3000 and tickets are sold at rupees 2 each .A person buys two tickets . What will be his expected gain if he buys :- a) one ticket of each series, B) tickets of same series? tests, examples and also practice B Com tests.

|
Explore Courses for B Com exam
|

|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.



















