Class 12 Exam > Class 12 Questions > The intensity variation in the two interferen...
Start Learning for Free
The intensity variation in the two interference pattern obtained with the help of 2 coherent sources is 5% of the average intensity. Find the ratio of intensities of two sources?
Most Upvoted Answer
The intensity variation in the two interference pattern obtained with ...
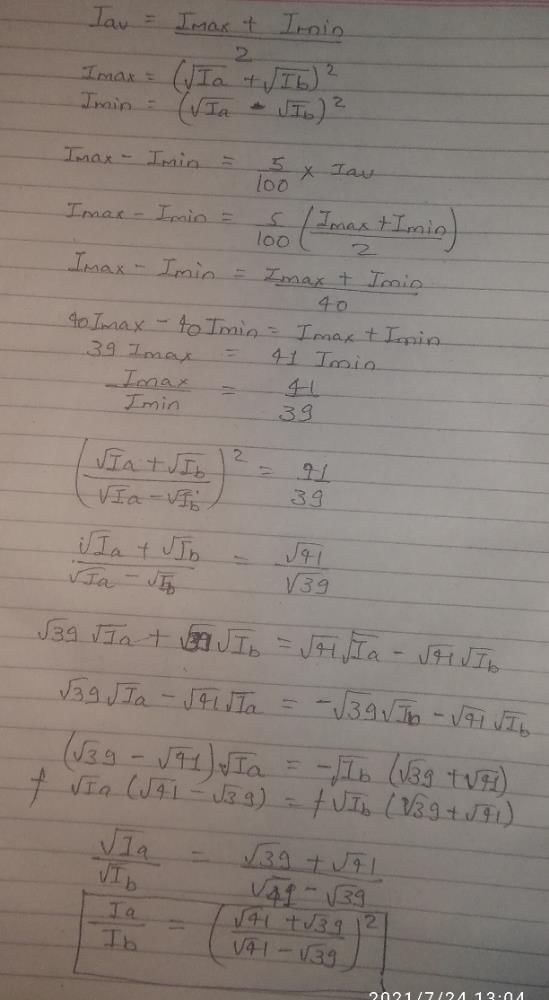
Community Answer
The intensity variation in the two interference pattern obtained with ...
Introduction
When two coherent sources of light interfere, they produce an interference pattern characterized by bright and dark regions. The intensity of the light in these regions can vary, and it is possible to calculate the ratio of intensities of the two sources based on the variation in intensity.
Interference Pattern and Intensity Variation
In an interference pattern, the bright regions are caused by constructive interference, where the crests of the two waves align, resulting in increased intensity. The dark regions, on the other hand, are caused by destructive interference, where the crests of one wave align with the troughs of the other, resulting in decreased intensity.
The intensity variation in the interference pattern is defined as the difference between the maximum and minimum intensities divided by the average intensity:
Intensity Variation = (Imax - Imin) / Iavg
Given that the intensity variation is 5% of the average intensity, we can write:
0.05 = (Imax - Imin) / Iavg
Calculating the Ratio of Intensities
Let's assume the intensities of the two sources are I1 and I2, where I1 is the intensity of the first source and I2 is the intensity of the second source. We need to find the ratio of intensities, which can be expressed as:
Ratio = I1 / I2
To proceed further, we need to make some assumptions. Let's assume that the maximum intensity occurs when the waves from both sources are in phase, resulting in constructive interference. In this case, Imax can be expressed as:
Imax = I1 + I2
Similarly, let's assume that the minimum intensity occurs when the waves from both sources are completely out of phase, resulting in destructive interference. In this case, Imin can be expressed as:
Imin = I1 - I2
Substituting these values into the intensity variation equation, we have:
0.05 = (I1 + I2 - I1 + I2) / (I1 + I2)
Simplifying the equation, we get:
0.05 = 2I2 / (I1 + I2)
Now, we can rearrange the equation to solve for the ratio of intensities:
Ratio = I1 / I2 = (2 / 0.05) - 1
Ratio = 40 - 1 = 39
Conclusion
The ratio of intensities of the two coherent sources is 39. This means that the intensity of the first source is 39 times greater than the intensity of the second source. The intensity variation in the interference pattern can provide valuable information about the relative intensities of the sources involved in the interference phenomenon. Understanding this ratio can help in various applications, such as determining the power distribution between different sources or analyzing the interference patterns in optical systems.
When two coherent sources of light interfere, they produce an interference pattern characterized by bright and dark regions. The intensity of the light in these regions can vary, and it is possible to calculate the ratio of intensities of the two sources based on the variation in intensity.
Interference Pattern and Intensity Variation
In an interference pattern, the bright regions are caused by constructive interference, where the crests of the two waves align, resulting in increased intensity. The dark regions, on the other hand, are caused by destructive interference, where the crests of one wave align with the troughs of the other, resulting in decreased intensity.
The intensity variation in the interference pattern is defined as the difference between the maximum and minimum intensities divided by the average intensity:
Intensity Variation = (Imax - Imin) / Iavg
Given that the intensity variation is 5% of the average intensity, we can write:
0.05 = (Imax - Imin) / Iavg
Calculating the Ratio of Intensities
Let's assume the intensities of the two sources are I1 and I2, where I1 is the intensity of the first source and I2 is the intensity of the second source. We need to find the ratio of intensities, which can be expressed as:
Ratio = I1 / I2
To proceed further, we need to make some assumptions. Let's assume that the maximum intensity occurs when the waves from both sources are in phase, resulting in constructive interference. In this case, Imax can be expressed as:
Imax = I1 + I2
Similarly, let's assume that the minimum intensity occurs when the waves from both sources are completely out of phase, resulting in destructive interference. In this case, Imin can be expressed as:
Imin = I1 - I2
Substituting these values into the intensity variation equation, we have:
0.05 = (I1 + I2 - I1 + I2) / (I1 + I2)
Simplifying the equation, we get:
0.05 = 2I2 / (I1 + I2)
Now, we can rearrange the equation to solve for the ratio of intensities:
Ratio = I1 / I2 = (2 / 0.05) - 1
Ratio = 40 - 1 = 39
Conclusion
The ratio of intensities of the two coherent sources is 39. This means that the intensity of the first source is 39 times greater than the intensity of the second source. The intensity variation in the interference pattern can provide valuable information about the relative intensities of the sources involved in the interference phenomenon. Understanding this ratio can help in various applications, such as determining the power distribution between different sources or analyzing the interference patterns in optical systems.

|
Explore Courses for Class 12 exam
|

|
Similar Class 12 Doubts
The intensity variation in the two interference pattern obtained with the help of 2 coherent sources is 5% of the average intensity. Find the ratio of intensities of two sources?
Question Description
The intensity variation in the two interference pattern obtained with the help of 2 coherent sources is 5% of the average intensity. Find the ratio of intensities of two sources? for Class 12 2024 is part of Class 12 preparation. The Question and answers have been prepared according to the Class 12 exam syllabus. Information about The intensity variation in the two interference pattern obtained with the help of 2 coherent sources is 5% of the average intensity. Find the ratio of intensities of two sources? covers all topics & solutions for Class 12 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for The intensity variation in the two interference pattern obtained with the help of 2 coherent sources is 5% of the average intensity. Find the ratio of intensities of two sources?.
The intensity variation in the two interference pattern obtained with the help of 2 coherent sources is 5% of the average intensity. Find the ratio of intensities of two sources? for Class 12 2024 is part of Class 12 preparation. The Question and answers have been prepared according to the Class 12 exam syllabus. Information about The intensity variation in the two interference pattern obtained with the help of 2 coherent sources is 5% of the average intensity. Find the ratio of intensities of two sources? covers all topics & solutions for Class 12 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for The intensity variation in the two interference pattern obtained with the help of 2 coherent sources is 5% of the average intensity. Find the ratio of intensities of two sources?.
Solutions for The intensity variation in the two interference pattern obtained with the help of 2 coherent sources is 5% of the average intensity. Find the ratio of intensities of two sources? in English & in Hindi are available as part of our courses for Class 12.
Download more important topics, notes, lectures and mock test series for Class 12 Exam by signing up for free.
Here you can find the meaning of The intensity variation in the two interference pattern obtained with the help of 2 coherent sources is 5% of the average intensity. Find the ratio of intensities of two sources? defined & explained in the simplest way possible. Besides giving the explanation of
The intensity variation in the two interference pattern obtained with the help of 2 coherent sources is 5% of the average intensity. Find the ratio of intensities of two sources?, a detailed solution for The intensity variation in the two interference pattern obtained with the help of 2 coherent sources is 5% of the average intensity. Find the ratio of intensities of two sources? has been provided alongside types of The intensity variation in the two interference pattern obtained with the help of 2 coherent sources is 5% of the average intensity. Find the ratio of intensities of two sources? theory, EduRev gives you an
ample number of questions to practice The intensity variation in the two interference pattern obtained with the help of 2 coherent sources is 5% of the average intensity. Find the ratio of intensities of two sources? tests, examples and also practice Class 12 tests.

|
Explore Courses for Class 12 exam
|

|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.



















