IIT JAM Exam > IIT JAM Questions > A uniform rod of mass m and length l is free ...
Start Learning for Free
A uniform rod of mass m and length l is free to rotate about a fixed axis through its end and perpendicular to its length . Find the period of small oscillations of the rod.?
Verified Answer
A uniform rod of mass m and length l is free to rotate about a fixed a...
Ans.
Method to Solve :
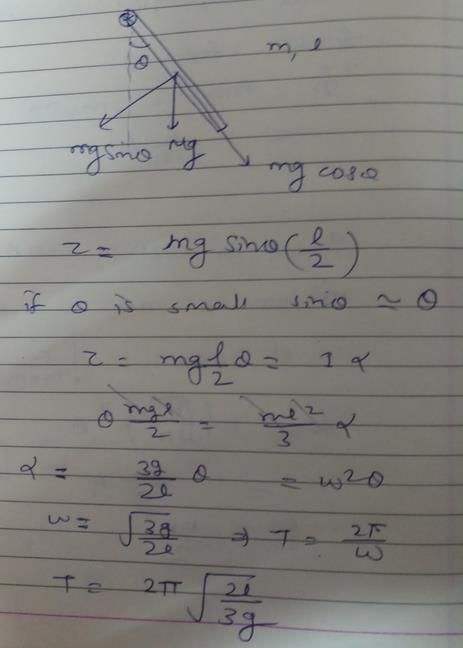
 This question is part of UPSC exam. View all IIT JAM courses
This question is part of UPSC exam. View all IIT JAM courses
Most Upvoted Answer
A uniform rod of mass m and length l is free to rotate about a fixed a...
Introduction:
To find the period of small oscillations of a uniform rod rotating freely about a fixed axis through its end and perpendicular to its length, we can use the principles of rotational motion and harmonic motion.
Key Concepts:
1. Moment of Inertia of a Uniform Rod: The moment of inertia of a uniform rod rotating about an axis through its end and perpendicular to its length is given by the formula I = (1/3) * m * l^2, where m is the mass of the rod and l is the length of the rod.
2. Torque and Angular Acceleration: When an external torque is applied to the rod, it produces angular acceleration according to the equation τ = I * α, where τ is the torque, I is the moment of inertia, and α is the angular acceleration.
3. Simple Harmonic Motion: For small oscillations, the motion of the rod can be approximated as simple harmonic motion, where the restoring torque is proportional to the angular displacement. The equation for simple harmonic motion is τ = -k * θ, where τ is the restoring torque, k is the angular spring constant, and θ is the angular displacement.
Calculating the Period:
To find the period of small oscillations, we need to calculate the angular spring constant and relate it to the period.
1. Calculating the Angular Spring Constant:
From the equation τ = -k * θ, we can equate it with τ = I * α and solve for k.
-τ = I * α
-k * θ = I * (d^2θ/dt^2)
-k * θ = (1/3) * m * l^2 * (d^2θ/dt^2)
Comparing the coefficients, we get k = (1/3) * m * l^2.
2. Relating the Angular Spring Constant to Period:
For simple harmonic motion, the angular frequency (ω) is related to the angular spring constant (k) by the equation ω = √(k/I).
The period (T) of the small oscillations can be calculated using the equation T = 2π/ω.
Substituting the value of ω and rearranging the equation, we get T = 2π * √(I/k).
T = 2π * √(3l^2/3ml^2)
T = 2π * √(1/m)
T = 2π * √(1/g) [Using g = 9.8 m/s^2]
Conclusion:
The period of small oscillations of a uniform rod rotating freely about a fixed axis through its end and perpendicular to its length is given by T = 2π * √(1/g), where g is the acceleration due to gravity. This period is independent of the length and mass of the rod.
To find the period of small oscillations of a uniform rod rotating freely about a fixed axis through its end and perpendicular to its length, we can use the principles of rotational motion and harmonic motion.
Key Concepts:
1. Moment of Inertia of a Uniform Rod: The moment of inertia of a uniform rod rotating about an axis through its end and perpendicular to its length is given by the formula I = (1/3) * m * l^2, where m is the mass of the rod and l is the length of the rod.
2. Torque and Angular Acceleration: When an external torque is applied to the rod, it produces angular acceleration according to the equation τ = I * α, where τ is the torque, I is the moment of inertia, and α is the angular acceleration.
3. Simple Harmonic Motion: For small oscillations, the motion of the rod can be approximated as simple harmonic motion, where the restoring torque is proportional to the angular displacement. The equation for simple harmonic motion is τ = -k * θ, where τ is the restoring torque, k is the angular spring constant, and θ is the angular displacement.
Calculating the Period:
To find the period of small oscillations, we need to calculate the angular spring constant and relate it to the period.
1. Calculating the Angular Spring Constant:
From the equation τ = -k * θ, we can equate it with τ = I * α and solve for k.
-τ = I * α
-k * θ = I * (d^2θ/dt^2)
-k * θ = (1/3) * m * l^2 * (d^2θ/dt^2)
Comparing the coefficients, we get k = (1/3) * m * l^2.
2. Relating the Angular Spring Constant to Period:
For simple harmonic motion, the angular frequency (ω) is related to the angular spring constant (k) by the equation ω = √(k/I).
The period (T) of the small oscillations can be calculated using the equation T = 2π/ω.
Substituting the value of ω and rearranging the equation, we get T = 2π * √(I/k).
T = 2π * √(3l^2/3ml^2)
T = 2π * √(1/m)
T = 2π * √(1/g) [Using g = 9.8 m/s^2]
Conclusion:
The period of small oscillations of a uniform rod rotating freely about a fixed axis through its end and perpendicular to its length is given by T = 2π * √(1/g), where g is the acceleration due to gravity. This period is independent of the length and mass of the rod.

|
Explore Courses for IIT JAM exam
|

|
Similar IIT JAM Doubts
A uniform rod of mass m and length l is free to rotate about a fixed axis through its end and perpendicular to its length . Find the period of small oscillations of the rod.?
Question Description
A uniform rod of mass m and length l is free to rotate about a fixed axis through its end and perpendicular to its length . Find the period of small oscillations of the rod.? for IIT JAM 2024 is part of IIT JAM preparation. The Question and answers have been prepared according to the IIT JAM exam syllabus. Information about A uniform rod of mass m and length l is free to rotate about a fixed axis through its end and perpendicular to its length . Find the period of small oscillations of the rod.? covers all topics & solutions for IIT JAM 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A uniform rod of mass m and length l is free to rotate about a fixed axis through its end and perpendicular to its length . Find the period of small oscillations of the rod.?.
A uniform rod of mass m and length l is free to rotate about a fixed axis through its end and perpendicular to its length . Find the period of small oscillations of the rod.? for IIT JAM 2024 is part of IIT JAM preparation. The Question and answers have been prepared according to the IIT JAM exam syllabus. Information about A uniform rod of mass m and length l is free to rotate about a fixed axis through its end and perpendicular to its length . Find the period of small oscillations of the rod.? covers all topics & solutions for IIT JAM 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A uniform rod of mass m and length l is free to rotate about a fixed axis through its end and perpendicular to its length . Find the period of small oscillations of the rod.?.
Solutions for A uniform rod of mass m and length l is free to rotate about a fixed axis through its end and perpendicular to its length . Find the period of small oscillations of the rod.? in English & in Hindi are available as part of our courses for IIT JAM.
Download more important topics, notes, lectures and mock test series for IIT JAM Exam by signing up for free.
Here you can find the meaning of A uniform rod of mass m and length l is free to rotate about a fixed axis through its end and perpendicular to its length . Find the period of small oscillations of the rod.? defined & explained in the simplest way possible. Besides giving the explanation of
A uniform rod of mass m and length l is free to rotate about a fixed axis through its end and perpendicular to its length . Find the period of small oscillations of the rod.?, a detailed solution for A uniform rod of mass m and length l is free to rotate about a fixed axis through its end and perpendicular to its length . Find the period of small oscillations of the rod.? has been provided alongside types of A uniform rod of mass m and length l is free to rotate about a fixed axis through its end and perpendicular to its length . Find the period of small oscillations of the rod.? theory, EduRev gives you an
ample number of questions to practice A uniform rod of mass m and length l is free to rotate about a fixed axis through its end and perpendicular to its length . Find the period of small oscillations of the rod.? tests, examples and also practice IIT JAM tests.

|
Explore Courses for IIT JAM exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.



















