JEE Exam > JEE Questions > A bullet fired into a fixed target loses half...
Start Learning for Free
A bullet fired into a fixed target loses half of its velocity after penetrating 3 cm. How much further it will penetrate before coming to rest assuming that it faces constant resistance to motion ?
- a)2.0 cm
- b)3.0 cm
- c)1.0 cm
- d)1.5 cm
Correct answer is option 'C'. Can you explain this answer?
Verified Answer
A bullet fired into a fixed target loses half of its velocity after pe...
Let K be the initial kinetic energy and F be the resistive force. Then according to work-energy theorem,
W = ΔK
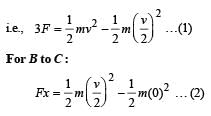
Dividing eqns. (1) and (2) we get x/3 = 1/3
or x = 1 cm
Most Upvoted Answer
A bullet fired into a fixed target loses half of its velocity after pe...
Given information:
- A bullet fired into a fixed target loses half of its velocity after penetrating 3 cm.
- The bullet faces constant resistance to motion.
To determine:
- How much further the bullet will penetrate before coming to rest.
Solution:
Let's assume the initial velocity of the bullet is V.
Step 1: Calculate the velocity after penetrating 3 cm
Since the bullet loses half of its velocity after penetrating 3 cm, the velocity after penetrating 3 cm can be calculated using the formula:
V_after = V_initial / 2
Step 2: Calculate the time taken to penetrate 3 cm
We can use the equation of motion to calculate the time taken to penetrate 3 cm. The equation is:
s = ut + (1/2)at^2
where:
- s is the distance traveled (3 cm or 0.03 m)
- u is the initial velocity (V)
- a is the acceleration (constant resistance to motion, which can be denoted as -k, where k is a positive constant)
- t is the time taken to travel the distance
Plugging in the given values, the equation becomes:
0.03 = V * t + (1/2)(-k)t^2
Step 3: Calculate the time taken to come to rest
When the bullet comes to rest, its final velocity is 0. Therefore, we can use the equation of motion to calculate the time taken to come to rest. The equation is:
v = u + at
where:
- v is the final velocity (0)
- u is the initial velocity (V_after)
- a is the acceleration (constant resistance to motion, which can be denoted as -k)
- t is the time taken to come to rest
Plugging in the given values, the equation becomes:
0 = V_after + (-k)t_rest
Step 4: Calculate the distance traveled after penetrating 3 cm
To find the distance traveled after penetrating 3 cm, we can use the equation of motion:
s = ut + (1/2)at^2
where:
- s is the distance traveled after penetrating 3 cm (let's denote it as S)
- u is the initial velocity after penetrating 3 cm (V_after)
- a is the acceleration (constant resistance to motion, which can be denoted as -k)
- t is the time taken to come to rest (t_rest)
Plugging in the given values, the equation becomes:
S = V_after * t_rest + (1/2)(-k)t_rest^2
Step 5: Substitute the values and calculate the distance
We have two equations from Step 2 and Step 4, which can be solved simultaneously to find the values of t and S.
From Step 2: 0.03 = V * t + (1/2)(-k)t^2
From Step 4: S = V_after * t_rest + (1/2)(-k)t_rest^2
Substituting V_after = V_initial / 2 and rearranging the equations, we get:
0.03 = V * t + (-k/2)t^2
S = (V_initial / 2) * t_rest +
- A bullet fired into a fixed target loses half of its velocity after penetrating 3 cm.
- The bullet faces constant resistance to motion.
To determine:
- How much further the bullet will penetrate before coming to rest.
Solution:
Let's assume the initial velocity of the bullet is V.
Step 1: Calculate the velocity after penetrating 3 cm
Since the bullet loses half of its velocity after penetrating 3 cm, the velocity after penetrating 3 cm can be calculated using the formula:
V_after = V_initial / 2
Step 2: Calculate the time taken to penetrate 3 cm
We can use the equation of motion to calculate the time taken to penetrate 3 cm. The equation is:
s = ut + (1/2)at^2
where:
- s is the distance traveled (3 cm or 0.03 m)
- u is the initial velocity (V)
- a is the acceleration (constant resistance to motion, which can be denoted as -k, where k is a positive constant)
- t is the time taken to travel the distance
Plugging in the given values, the equation becomes:
0.03 = V * t + (1/2)(-k)t^2
Step 3: Calculate the time taken to come to rest
When the bullet comes to rest, its final velocity is 0. Therefore, we can use the equation of motion to calculate the time taken to come to rest. The equation is:
v = u + at
where:
- v is the final velocity (0)
- u is the initial velocity (V_after)
- a is the acceleration (constant resistance to motion, which can be denoted as -k)
- t is the time taken to come to rest
Plugging in the given values, the equation becomes:
0 = V_after + (-k)t_rest
Step 4: Calculate the distance traveled after penetrating 3 cm
To find the distance traveled after penetrating 3 cm, we can use the equation of motion:
s = ut + (1/2)at^2
where:
- s is the distance traveled after penetrating 3 cm (let's denote it as S)
- u is the initial velocity after penetrating 3 cm (V_after)
- a is the acceleration (constant resistance to motion, which can be denoted as -k)
- t is the time taken to come to rest (t_rest)
Plugging in the given values, the equation becomes:
S = V_after * t_rest + (1/2)(-k)t_rest^2
Step 5: Substitute the values and calculate the distance
We have two equations from Step 2 and Step 4, which can be solved simultaneously to find the values of t and S.
From Step 2: 0.03 = V * t + (1/2)(-k)t^2
From Step 4: S = V_after * t_rest + (1/2)(-k)t_rest^2
Substituting V_after = V_initial / 2 and rearranging the equations, we get:
0.03 = V * t + (-k/2)t^2
S = (V_initial / 2) * t_rest +

|
Explore Courses for JEE exam
|

|
Similar JEE Doubts
A bullet fired into a fixed target loses half of its velocity after penetrating 3 cm. How much further it will penetrate before coming to rest assuming that it faces constant resistance to motion ?a)2.0 cmb)3.0 cmc)1.0 cmd)1.5 cmCorrect answer is option 'C'. Can you explain this answer?
Question Description
A bullet fired into a fixed target loses half of its velocity after penetrating 3 cm. How much further it will penetrate before coming to rest assuming that it faces constant resistance to motion ?a)2.0 cmb)3.0 cmc)1.0 cmd)1.5 cmCorrect answer is option 'C'. Can you explain this answer? for JEE 2025 is part of JEE preparation. The Question and answers have been prepared according to the JEE exam syllabus. Information about A bullet fired into a fixed target loses half of its velocity after penetrating 3 cm. How much further it will penetrate before coming to rest assuming that it faces constant resistance to motion ?a)2.0 cmb)3.0 cmc)1.0 cmd)1.5 cmCorrect answer is option 'C'. Can you explain this answer? covers all topics & solutions for JEE 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A bullet fired into a fixed target loses half of its velocity after penetrating 3 cm. How much further it will penetrate before coming to rest assuming that it faces constant resistance to motion ?a)2.0 cmb)3.0 cmc)1.0 cmd)1.5 cmCorrect answer is option 'C'. Can you explain this answer?.
A bullet fired into a fixed target loses half of its velocity after penetrating 3 cm. How much further it will penetrate before coming to rest assuming that it faces constant resistance to motion ?a)2.0 cmb)3.0 cmc)1.0 cmd)1.5 cmCorrect answer is option 'C'. Can you explain this answer? for JEE 2025 is part of JEE preparation. The Question and answers have been prepared according to the JEE exam syllabus. Information about A bullet fired into a fixed target loses half of its velocity after penetrating 3 cm. How much further it will penetrate before coming to rest assuming that it faces constant resistance to motion ?a)2.0 cmb)3.0 cmc)1.0 cmd)1.5 cmCorrect answer is option 'C'. Can you explain this answer? covers all topics & solutions for JEE 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A bullet fired into a fixed target loses half of its velocity after penetrating 3 cm. How much further it will penetrate before coming to rest assuming that it faces constant resistance to motion ?a)2.0 cmb)3.0 cmc)1.0 cmd)1.5 cmCorrect answer is option 'C'. Can you explain this answer?.
Solutions for A bullet fired into a fixed target loses half of its velocity after penetrating 3 cm. How much further it will penetrate before coming to rest assuming that it faces constant resistance to motion ?a)2.0 cmb)3.0 cmc)1.0 cmd)1.5 cmCorrect answer is option 'C'. Can you explain this answer? in English & in Hindi are available as part of our courses for JEE.
Download more important topics, notes, lectures and mock test series for JEE Exam by signing up for free.
Here you can find the meaning of A bullet fired into a fixed target loses half of its velocity after penetrating 3 cm. How much further it will penetrate before coming to rest assuming that it faces constant resistance to motion ?a)2.0 cmb)3.0 cmc)1.0 cmd)1.5 cmCorrect answer is option 'C'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
A bullet fired into a fixed target loses half of its velocity after penetrating 3 cm. How much further it will penetrate before coming to rest assuming that it faces constant resistance to motion ?a)2.0 cmb)3.0 cmc)1.0 cmd)1.5 cmCorrect answer is option 'C'. Can you explain this answer?, a detailed solution for A bullet fired into a fixed target loses half of its velocity after penetrating 3 cm. How much further it will penetrate before coming to rest assuming that it faces constant resistance to motion ?a)2.0 cmb)3.0 cmc)1.0 cmd)1.5 cmCorrect answer is option 'C'. Can you explain this answer? has been provided alongside types of A bullet fired into a fixed target loses half of its velocity after penetrating 3 cm. How much further it will penetrate before coming to rest assuming that it faces constant resistance to motion ?a)2.0 cmb)3.0 cmc)1.0 cmd)1.5 cmCorrect answer is option 'C'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice A bullet fired into a fixed target loses half of its velocity after penetrating 3 cm. How much further it will penetrate before coming to rest assuming that it faces constant resistance to motion ?a)2.0 cmb)3.0 cmc)1.0 cmd)1.5 cmCorrect answer is option 'C'. Can you explain this answer? tests, examples and also practice JEE tests.

|
Explore Courses for JEE exam
|

|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
























