Class 12 Exam > Class 12 Questions > A parallel beam light emerges from the opposi...
Start Learning for Free
A parallel beam light emerges from the opposite surface of the sphere when point source of light at the surface of the sphere the refractive index of the sphere is?
Verified Answer
A parallel beam light emerges from the opposite surface of the sphere ...
Given: A point source of light at the surface of a sphere causes a parallel beam of light to emerge from the opposite surface of the sphere.
To find the refractive index of the material of the sphere
Solution:
The distance of the source from the opposite surface of the sphere, u=−2R
Now,
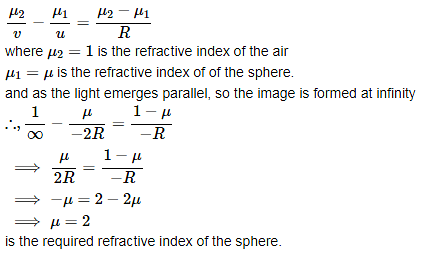
 This question is part of UPSC exam. View all Class 12 courses
This question is part of UPSC exam. View all Class 12 courses
Most Upvoted Answer
A parallel beam light emerges from the opposite surface of the sphere ...
Introduction:
When a point source of light is placed at the surface of a sphere, and a parallel beam of light emerges from the opposite surface, we can determine the refractive index of the sphere. The refractive index is a measure of how much a material slows down the speed of light as it passes through.
Explanation:
1. Principle of refraction:
According to the principle of refraction, when light passes from one medium to another, it changes its direction due to the change in speed. This change in direction is known as refraction.
2. Refraction at spherical surfaces:
When light passes through a spherical surface, it undergoes refraction. The amount of refraction depends on the refractive indices of the two media involved.
3. Parallel beam of light:
A parallel beam of light means that the light rays are all parallel to each other. In order for a parallel beam of light to emerge from the opposite surface of the sphere, the incident rays must be refracted in such a way that they become parallel.
4. Conditions for a parallel beam:
In order for a parallel beam to emerge, the refractive index of the medium surrounding the point source of light must be lower than the refractive index of the sphere.
5. Explanation:
When the point source of light is at the surface of the sphere, the incident rays will refract as they enter the sphere. The refracted rays will converge towards the center of the sphere and then diverge as they exit the sphere.
If the refractive index of the sphere is higher than the surrounding medium, the rays will converge towards the center of the sphere and then continue to converge even after exiting the sphere. In this case, a parallel beam of light will not emerge.
However, if the refractive index of the sphere is lower than the surrounding medium, the rays will converge towards the center of the sphere and then diverge after exiting the sphere. In this case, a parallel beam of light will emerge.
6. Determining the refractive index:
To determine the refractive index of the sphere, we can compare the angles of incidence and refraction using Snell's law. Snell's law states that the ratio of the sine of the angle of incidence to the sine of the angle of refraction is equal to the ratio of the refractive indices of the two media.
By measuring the angles of incidence and refraction, we can calculate the refractive index of the sphere using Snell's law.
Conclusion:
When a parallel beam of light emerges from the opposite surface of a sphere with a point source of light at its surface, it indicates that the refractive index of the sphere is lower than the refractive index of the surrounding medium. By measuring the angles of incidence and refraction, we can determine the refractive index of the sphere using Snell's law.
When a point source of light is placed at the surface of a sphere, and a parallel beam of light emerges from the opposite surface, we can determine the refractive index of the sphere. The refractive index is a measure of how much a material slows down the speed of light as it passes through.
Explanation:
1. Principle of refraction:
According to the principle of refraction, when light passes from one medium to another, it changes its direction due to the change in speed. This change in direction is known as refraction.
2. Refraction at spherical surfaces:
When light passes through a spherical surface, it undergoes refraction. The amount of refraction depends on the refractive indices of the two media involved.
3. Parallel beam of light:
A parallel beam of light means that the light rays are all parallel to each other. In order for a parallel beam of light to emerge from the opposite surface of the sphere, the incident rays must be refracted in such a way that they become parallel.
4. Conditions for a parallel beam:
In order for a parallel beam to emerge, the refractive index of the medium surrounding the point source of light must be lower than the refractive index of the sphere.
5. Explanation:
When the point source of light is at the surface of the sphere, the incident rays will refract as they enter the sphere. The refracted rays will converge towards the center of the sphere and then diverge as they exit the sphere.
If the refractive index of the sphere is higher than the surrounding medium, the rays will converge towards the center of the sphere and then continue to converge even after exiting the sphere. In this case, a parallel beam of light will not emerge.
However, if the refractive index of the sphere is lower than the surrounding medium, the rays will converge towards the center of the sphere and then diverge after exiting the sphere. In this case, a parallel beam of light will emerge.
6. Determining the refractive index:
To determine the refractive index of the sphere, we can compare the angles of incidence and refraction using Snell's law. Snell's law states that the ratio of the sine of the angle of incidence to the sine of the angle of refraction is equal to the ratio of the refractive indices of the two media.
By measuring the angles of incidence and refraction, we can calculate the refractive index of the sphere using Snell's law.
Conclusion:
When a parallel beam of light emerges from the opposite surface of a sphere with a point source of light at its surface, it indicates that the refractive index of the sphere is lower than the refractive index of the surrounding medium. By measuring the angles of incidence and refraction, we can determine the refractive index of the sphere using Snell's law.

|
Explore Courses for Class 12 exam
|

|
Similar Class 12 Doubts
A parallel beam light emerges from the opposite surface of the sphere when point source of light at the surface of the sphere the refractive index of the sphere is?
Question Description
A parallel beam light emerges from the opposite surface of the sphere when point source of light at the surface of the sphere the refractive index of the sphere is? for Class 12 2024 is part of Class 12 preparation. The Question and answers have been prepared according to the Class 12 exam syllabus. Information about A parallel beam light emerges from the opposite surface of the sphere when point source of light at the surface of the sphere the refractive index of the sphere is? covers all topics & solutions for Class 12 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A parallel beam light emerges from the opposite surface of the sphere when point source of light at the surface of the sphere the refractive index of the sphere is?.
A parallel beam light emerges from the opposite surface of the sphere when point source of light at the surface of the sphere the refractive index of the sphere is? for Class 12 2024 is part of Class 12 preparation. The Question and answers have been prepared according to the Class 12 exam syllabus. Information about A parallel beam light emerges from the opposite surface of the sphere when point source of light at the surface of the sphere the refractive index of the sphere is? covers all topics & solutions for Class 12 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A parallel beam light emerges from the opposite surface of the sphere when point source of light at the surface of the sphere the refractive index of the sphere is?.
Solutions for A parallel beam light emerges from the opposite surface of the sphere when point source of light at the surface of the sphere the refractive index of the sphere is? in English & in Hindi are available as part of our courses for Class 12.
Download more important topics, notes, lectures and mock test series for Class 12 Exam by signing up for free.
Here you can find the meaning of A parallel beam light emerges from the opposite surface of the sphere when point source of light at the surface of the sphere the refractive index of the sphere is? defined & explained in the simplest way possible. Besides giving the explanation of
A parallel beam light emerges from the opposite surface of the sphere when point source of light at the surface of the sphere the refractive index of the sphere is?, a detailed solution for A parallel beam light emerges from the opposite surface of the sphere when point source of light at the surface of the sphere the refractive index of the sphere is? has been provided alongside types of A parallel beam light emerges from the opposite surface of the sphere when point source of light at the surface of the sphere the refractive index of the sphere is? theory, EduRev gives you an
ample number of questions to practice A parallel beam light emerges from the opposite surface of the sphere when point source of light at the surface of the sphere the refractive index of the sphere is? tests, examples and also practice Class 12 tests.

|
Explore Courses for Class 12 exam
|

|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.



















