IIT JAM Exam > IIT JAM Questions > A particle of unit mass is moving in one dime...
Start Learning for Free
A particle of unit mass is moving in one dimension potential V(x)=x²-x⁴. the minimum mechanical energy above which the motion of the particle cannot be bounded for any given initial condition is
Verified Answer
A particle of unit mass is moving in one dimension potential V(x)=x²-x...
Solution :
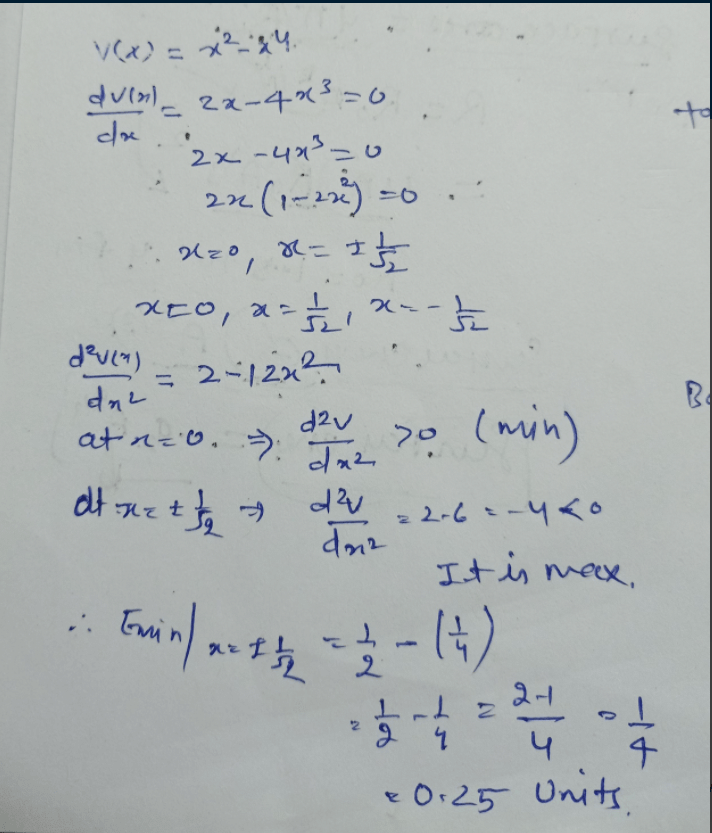
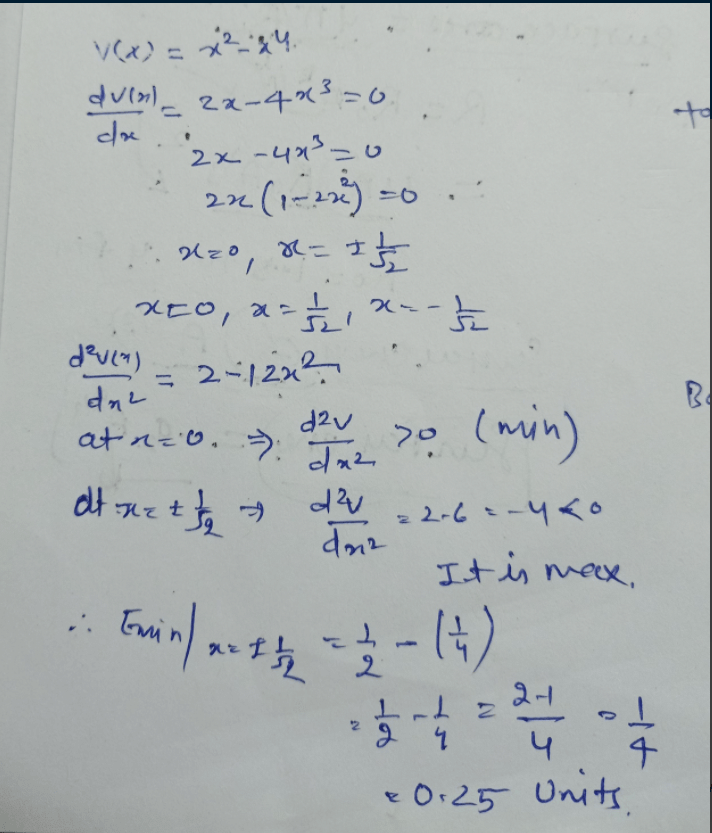
 This question is part of UPSC exam. View all IIT JAM courses
This question is part of UPSC exam. View all IIT JAM courses
Most Upvoted Answer
A particle of unit mass is moving in one dimension potential V(x)=x²-x...
Minimum Mechanical Energy for Unbounded Motion
The minimum mechanical energy above which the motion of the particle cannot be bounded for any given initial condition in the potential V(x)=x²-x⁴ can be determined by analyzing the behavior of the potential function and considering the conditions for unbounded motion.
Understanding the Potential Function
The potential function V(x)=x²-x⁴ represents a one-dimensional potential energy landscape. It is a downward-opening parabola with a maximum value at the origin (x=0) and two local minima at x=-1 and x=1. The potential function approaches positive infinity as x approaches negative or positive infinity.
Determining the Boundaries of Motion
To determine the boundaries of motion, we need to consider the turning points of the potential function. These turning points occur where the derivative of the potential function is equal to zero.
Taking the derivative of the potential function with respect to x, we get:
V'(x) = 2x - 4x³
Setting V'(x) equal to zero and solving for x, we find the turning points:
2x - 4x³ = 0
2x(1 - 2x²) = 0
x = 0 or x = ±1/√2
Therefore, the turning points are located at x = 0, x = 1/√2, and x = -1/√2.
Analyzing the Energy Levels
To understand the behavior of the particle for different energy levels, we need to consider the total mechanical energy of the system. The total mechanical energy (E) is given by the sum of the kinetic energy (K) and the potential energy (V). Since the mass of the particle is unity, the kinetic energy is given by K = p²/2m = p²/2.
For bounded motion, the total mechanical energy of the particle must be less than or equal to the maximum value of the potential energy. In this case, the maximum value of the potential energy occurs at the origin, V(0) = 0.
Minimum Mechanical Energy for Unbounded Motion
For unbounded motion, the total mechanical energy of the particle must be greater than the maximum value of the potential energy. Since the potential energy approaches positive infinity as x approaches negative or positive infinity, there is no upper limit on the potential energy.
Therefore, the minimum mechanical energy above which the motion of the particle cannot be bounded for any given initial condition is infinity.
Conclusion
In the potential V(x)=x²-x⁴, the minimum mechanical energy above which the motion of the particle cannot be bounded for any given initial condition is infinity. This is because the potential energy approaches positive infinity as x approaches negative or positive infinity, making it impossible to confine the particle within any finite energy level.
The minimum mechanical energy above which the motion of the particle cannot be bounded for any given initial condition in the potential V(x)=x²-x⁴ can be determined by analyzing the behavior of the potential function and considering the conditions for unbounded motion.
Understanding the Potential Function
The potential function V(x)=x²-x⁴ represents a one-dimensional potential energy landscape. It is a downward-opening parabola with a maximum value at the origin (x=0) and two local minima at x=-1 and x=1. The potential function approaches positive infinity as x approaches negative or positive infinity.
Determining the Boundaries of Motion
To determine the boundaries of motion, we need to consider the turning points of the potential function. These turning points occur where the derivative of the potential function is equal to zero.
Taking the derivative of the potential function with respect to x, we get:
V'(x) = 2x - 4x³
Setting V'(x) equal to zero and solving for x, we find the turning points:
2x - 4x³ = 0
2x(1 - 2x²) = 0
x = 0 or x = ±1/√2
Therefore, the turning points are located at x = 0, x = 1/√2, and x = -1/√2.
Analyzing the Energy Levels
To understand the behavior of the particle for different energy levels, we need to consider the total mechanical energy of the system. The total mechanical energy (E) is given by the sum of the kinetic energy (K) and the potential energy (V). Since the mass of the particle is unity, the kinetic energy is given by K = p²/2m = p²/2.
For bounded motion, the total mechanical energy of the particle must be less than or equal to the maximum value of the potential energy. In this case, the maximum value of the potential energy occurs at the origin, V(0) = 0.
Minimum Mechanical Energy for Unbounded Motion
For unbounded motion, the total mechanical energy of the particle must be greater than the maximum value of the potential energy. Since the potential energy approaches positive infinity as x approaches negative or positive infinity, there is no upper limit on the potential energy.
Therefore, the minimum mechanical energy above which the motion of the particle cannot be bounded for any given initial condition is infinity.
Conclusion
In the potential V(x)=x²-x⁴, the minimum mechanical energy above which the motion of the particle cannot be bounded for any given initial condition is infinity. This is because the potential energy approaches positive infinity as x approaches negative or positive infinity, making it impossible to confine the particle within any finite energy level.

|
Explore Courses for IIT JAM exam
|

|
Similar IIT JAM Doubts
A particle of unit mass is moving in one dimension potential V(x)=x²-x⁴. the minimum mechanical energy above which the motion of the particle cannot be bounded for any given initial condition is
Question Description
A particle of unit mass is moving in one dimension potential V(x)=x²-x⁴. the minimum mechanical energy above which the motion of the particle cannot be bounded for any given initial condition is for IIT JAM 2024 is part of IIT JAM preparation. The Question and answers have been prepared according to the IIT JAM exam syllabus. Information about A particle of unit mass is moving in one dimension potential V(x)=x²-x⁴. the minimum mechanical energy above which the motion of the particle cannot be bounded for any given initial condition is covers all topics & solutions for IIT JAM 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A particle of unit mass is moving in one dimension potential V(x)=x²-x⁴. the minimum mechanical energy above which the motion of the particle cannot be bounded for any given initial condition is.
A particle of unit mass is moving in one dimension potential V(x)=x²-x⁴. the minimum mechanical energy above which the motion of the particle cannot be bounded for any given initial condition is for IIT JAM 2024 is part of IIT JAM preparation. The Question and answers have been prepared according to the IIT JAM exam syllabus. Information about A particle of unit mass is moving in one dimension potential V(x)=x²-x⁴. the minimum mechanical energy above which the motion of the particle cannot be bounded for any given initial condition is covers all topics & solutions for IIT JAM 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A particle of unit mass is moving in one dimension potential V(x)=x²-x⁴. the minimum mechanical energy above which the motion of the particle cannot be bounded for any given initial condition is.
Solutions for A particle of unit mass is moving in one dimension potential V(x)=x²-x⁴. the minimum mechanical energy above which the motion of the particle cannot be bounded for any given initial condition is in English & in Hindi are available as part of our courses for IIT JAM.
Download more important topics, notes, lectures and mock test series for IIT JAM Exam by signing up for free.
Here you can find the meaning of A particle of unit mass is moving in one dimension potential V(x)=x²-x⁴. the minimum mechanical energy above which the motion of the particle cannot be bounded for any given initial condition is defined & explained in the simplest way possible. Besides giving the explanation of
A particle of unit mass is moving in one dimension potential V(x)=x²-x⁴. the minimum mechanical energy above which the motion of the particle cannot be bounded for any given initial condition is, a detailed solution for A particle of unit mass is moving in one dimension potential V(x)=x²-x⁴. the minimum mechanical energy above which the motion of the particle cannot be bounded for any given initial condition is has been provided alongside types of A particle of unit mass is moving in one dimension potential V(x)=x²-x⁴. the minimum mechanical energy above which the motion of the particle cannot be bounded for any given initial condition is theory, EduRev gives you an
ample number of questions to practice A particle of unit mass is moving in one dimension potential V(x)=x²-x⁴. the minimum mechanical energy above which the motion of the particle cannot be bounded for any given initial condition is tests, examples and also practice IIT JAM tests.

|
Explore Courses for IIT JAM exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.



















