Civil Engineering (CE) Exam > Civil Engineering (CE) Questions > A doubly reinforced concrete beam has effecti...
Start Learning for Free
A doubly reinforced concrete beam has effective cover d’ to the centre of compression reinforcement. ‘xu’ is the depth of neutral axis, and d is the effective depth to the centre of tension reinforcement. What is the maximum strain in concrete at the level of compression reinforcement?
- a)0.0035 (1 – d’/d)
- b)0.0035 (1 – d’/xu)
- c)0.002 (1 – d’/xu)
- d)0.002 (1 – d’/d)
Correct answer is option 'B'. Can you explain this answer?
Verified Answer
A doubly reinforced concrete beam has effective cover d’ to the ...
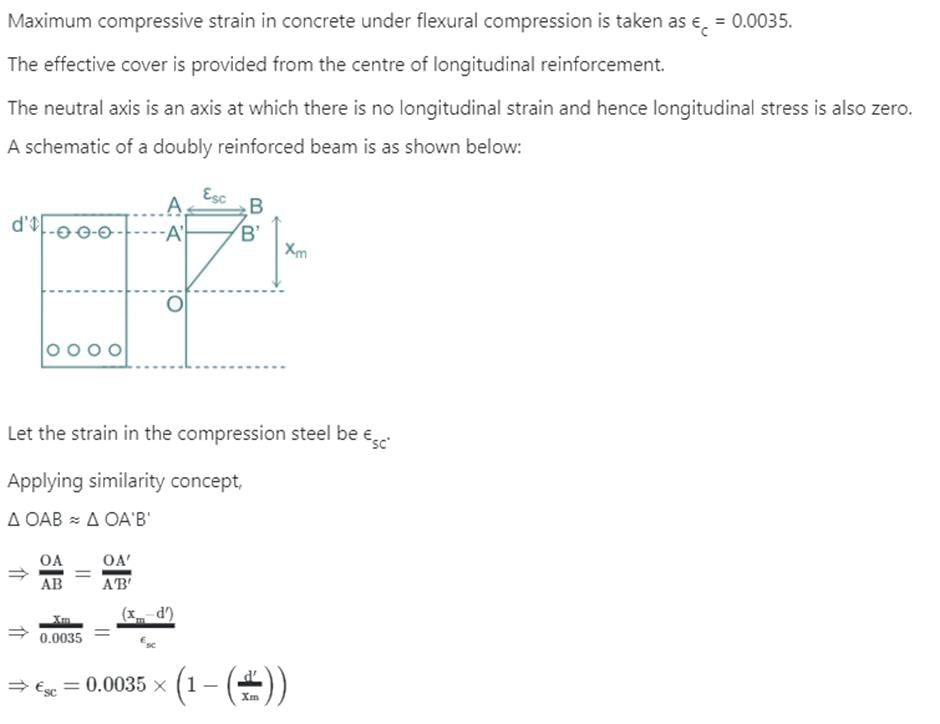
Most Upvoted Answer
A doubly reinforced concrete beam has effective cover d’ to the ...
Epth of 50 mm on both the compression and tension sides. The beam has a width of 250 mm, an overall depth of 500 mm, and is reinforced with two sets of bars. The first set consists of 4 bars of 20 mm diameter on the tension side, and the second set consists of 4 bars of 20 mm diameter on the compression side. The concrete has a compressive strength of 25 MPa, and the yield strength of the steel is 500 MPa.
To design the beam, the following steps can be followed:
1. Determine the effective depth of the beam:
Effective depth = overall depth - effective cover depth
Effective depth = 500 mm - 50 mm - 50 mm = 400 mm
2. Calculate the moment capacity of the beam using the balanced reinforcement method:
Assuming balanced reinforcement, the tensile and compressive forces in the steel are equal at the ultimate limit state.
As = Ast = Asc
where As = area of steel required
Ast = area of steel on tension side
Asc = area of steel on compression side
Assuming a neutral axis depth of d, the moment capacity of the beam can be calculated as:
M = 0.68 fck bd^2 + Ast (fy/γs) (d - d')
where fck = concrete compressive strength
fy = steel yield strength
γs = partial safety factor for steel
Taking γs = 1.15, the moment capacity can be calculated as:
M = 0.68 x 25 x 250 x 400^2 + 4 x π/4 x 20^2 x 500 x (500 - 50 - 20) x 500/1.15
M = 138,400 kNm
3. Check deflection:
The maximum allowable deflection can be taken as L/250, where L is the span of the beam. Assuming a span of 6 meters, the maximum allowable deflection is:
δmax = 6000/250 = 24 mm
Using standard formulas for deflection, the actual deflection can be calculated as:
δ = (5wL^4)/(384EI)
where w = self-weight of the beam
E = modulus of elasticity of concrete
I = moment of inertia of the beam
Assuming a self-weight of the beam of 25 kN/m and a modulus of elasticity of 28,000 MPa, the moment of inertia can be calculated as:
I = (1/12)bh^3 + Ast (d - d')^2 + Asc (d - d')^2
I = (1/12) x 250 x 400^3 + 4 x π/4 x 20^2 x (400 - 50 - 20)^2 + 4 x π/4 x 20^2 x (50 + 20)^2
I = 72.9 x 10^6 mm^4
Substituting the values into the deflection formula, the actual deflection is:
δ = (5 x 25 x 6^4)/(384 x 28000 x 72.9 x 10^6) = 4.3 mm
Since the actual deflection is less than the maximum allowable deflection, the beam
To design the beam, the following steps can be followed:
1. Determine the effective depth of the beam:
Effective depth = overall depth - effective cover depth
Effective depth = 500 mm - 50 mm - 50 mm = 400 mm
2. Calculate the moment capacity of the beam using the balanced reinforcement method:
Assuming balanced reinforcement, the tensile and compressive forces in the steel are equal at the ultimate limit state.
As = Ast = Asc
where As = area of steel required
Ast = area of steel on tension side
Asc = area of steel on compression side
Assuming a neutral axis depth of d, the moment capacity of the beam can be calculated as:
M = 0.68 fck bd^2 + Ast (fy/γs) (d - d')
where fck = concrete compressive strength
fy = steel yield strength
γs = partial safety factor for steel
Taking γs = 1.15, the moment capacity can be calculated as:
M = 0.68 x 25 x 250 x 400^2 + 4 x π/4 x 20^2 x 500 x (500 - 50 - 20) x 500/1.15
M = 138,400 kNm
3. Check deflection:
The maximum allowable deflection can be taken as L/250, where L is the span of the beam. Assuming a span of 6 meters, the maximum allowable deflection is:
δmax = 6000/250 = 24 mm
Using standard formulas for deflection, the actual deflection can be calculated as:
δ = (5wL^4)/(384EI)
where w = self-weight of the beam
E = modulus of elasticity of concrete
I = moment of inertia of the beam
Assuming a self-weight of the beam of 25 kN/m and a modulus of elasticity of 28,000 MPa, the moment of inertia can be calculated as:
I = (1/12)bh^3 + Ast (d - d')^2 + Asc (d - d')^2
I = (1/12) x 250 x 400^3 + 4 x π/4 x 20^2 x (400 - 50 - 20)^2 + 4 x π/4 x 20^2 x (50 + 20)^2
I = 72.9 x 10^6 mm^4
Substituting the values into the deflection formula, the actual deflection is:
δ = (5 x 25 x 6^4)/(384 x 28000 x 72.9 x 10^6) = 4.3 mm
Since the actual deflection is less than the maximum allowable deflection, the beam
Free Test
FREE
| Start Free Test |
Community Answer
A doubly reinforced concrete beam has effective cover d’ to the ...
Can anyone explain this?
|
Explore Courses for Civil Engineering (CE) exam
|
|
Similar Civil Engineering (CE) Doubts
Top Courses for Civil Engineering (CE)View all
A doubly reinforced concrete beam has effective cover d’ to the centre of compression reinforcement. ‘xu’ is the depth of neutral axis, and d is the effective depth to the centre of tension reinforcement. What is the maximum strain in concrete at the level of compression reinforcement?a)0.0035 (1 – d’/d)b)0.0035 (1 – d’/xu)c)0.002 (1 – d’/xu)d)0.002 (1 – d’/d)Correct answer is option 'B'. Can you explain this answer?
Question Description
A doubly reinforced concrete beam has effective cover d’ to the centre of compression reinforcement. ‘xu’ is the depth of neutral axis, and d is the effective depth to the centre of tension reinforcement. What is the maximum strain in concrete at the level of compression reinforcement?a)0.0035 (1 – d’/d)b)0.0035 (1 – d’/xu)c)0.002 (1 – d’/xu)d)0.002 (1 – d’/d)Correct answer is option 'B'. Can you explain this answer? for Civil Engineering (CE) 2025 is part of Civil Engineering (CE) preparation. The Question and answers have been prepared according to the Civil Engineering (CE) exam syllabus. Information about A doubly reinforced concrete beam has effective cover d’ to the centre of compression reinforcement. ‘xu’ is the depth of neutral axis, and d is the effective depth to the centre of tension reinforcement. What is the maximum strain in concrete at the level of compression reinforcement?a)0.0035 (1 – d’/d)b)0.0035 (1 – d’/xu)c)0.002 (1 – d’/xu)d)0.002 (1 – d’/d)Correct answer is option 'B'. Can you explain this answer? covers all topics & solutions for Civil Engineering (CE) 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A doubly reinforced concrete beam has effective cover d’ to the centre of compression reinforcement. ‘xu’ is the depth of neutral axis, and d is the effective depth to the centre of tension reinforcement. What is the maximum strain in concrete at the level of compression reinforcement?a)0.0035 (1 – d’/d)b)0.0035 (1 – d’/xu)c)0.002 (1 – d’/xu)d)0.002 (1 – d’/d)Correct answer is option 'B'. Can you explain this answer?.
A doubly reinforced concrete beam has effective cover d’ to the centre of compression reinforcement. ‘xu’ is the depth of neutral axis, and d is the effective depth to the centre of tension reinforcement. What is the maximum strain in concrete at the level of compression reinforcement?a)0.0035 (1 – d’/d)b)0.0035 (1 – d’/xu)c)0.002 (1 – d’/xu)d)0.002 (1 – d’/d)Correct answer is option 'B'. Can you explain this answer? for Civil Engineering (CE) 2025 is part of Civil Engineering (CE) preparation. The Question and answers have been prepared according to the Civil Engineering (CE) exam syllabus. Information about A doubly reinforced concrete beam has effective cover d’ to the centre of compression reinforcement. ‘xu’ is the depth of neutral axis, and d is the effective depth to the centre of tension reinforcement. What is the maximum strain in concrete at the level of compression reinforcement?a)0.0035 (1 – d’/d)b)0.0035 (1 – d’/xu)c)0.002 (1 – d’/xu)d)0.002 (1 – d’/d)Correct answer is option 'B'. Can you explain this answer? covers all topics & solutions for Civil Engineering (CE) 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A doubly reinforced concrete beam has effective cover d’ to the centre of compression reinforcement. ‘xu’ is the depth of neutral axis, and d is the effective depth to the centre of tension reinforcement. What is the maximum strain in concrete at the level of compression reinforcement?a)0.0035 (1 – d’/d)b)0.0035 (1 – d’/xu)c)0.002 (1 – d’/xu)d)0.002 (1 – d’/d)Correct answer is option 'B'. Can you explain this answer?.
Solutions for A doubly reinforced concrete beam has effective cover d’ to the centre of compression reinforcement. ‘xu’ is the depth of neutral axis, and d is the effective depth to the centre of tension reinforcement. What is the maximum strain in concrete at the level of compression reinforcement?a)0.0035 (1 – d’/d)b)0.0035 (1 – d’/xu)c)0.002 (1 – d’/xu)d)0.002 (1 – d’/d)Correct answer is option 'B'. Can you explain this answer? in English & in Hindi are available as part of our courses for Civil Engineering (CE).
Download more important topics, notes, lectures and mock test series for Civil Engineering (CE) Exam by signing up for free.
Here you can find the meaning of A doubly reinforced concrete beam has effective cover d’ to the centre of compression reinforcement. ‘xu’ is the depth of neutral axis, and d is the effective depth to the centre of tension reinforcement. What is the maximum strain in concrete at the level of compression reinforcement?a)0.0035 (1 – d’/d)b)0.0035 (1 – d’/xu)c)0.002 (1 – d’/xu)d)0.002 (1 – d’/d)Correct answer is option 'B'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
A doubly reinforced concrete beam has effective cover d’ to the centre of compression reinforcement. ‘xu’ is the depth of neutral axis, and d is the effective depth to the centre of tension reinforcement. What is the maximum strain in concrete at the level of compression reinforcement?a)0.0035 (1 – d’/d)b)0.0035 (1 – d’/xu)c)0.002 (1 – d’/xu)d)0.002 (1 – d’/d)Correct answer is option 'B'. Can you explain this answer?, a detailed solution for A doubly reinforced concrete beam has effective cover d’ to the centre of compression reinforcement. ‘xu’ is the depth of neutral axis, and d is the effective depth to the centre of tension reinforcement. What is the maximum strain in concrete at the level of compression reinforcement?a)0.0035 (1 – d’/d)b)0.0035 (1 – d’/xu)c)0.002 (1 – d’/xu)d)0.002 (1 – d’/d)Correct answer is option 'B'. Can you explain this answer? has been provided alongside types of A doubly reinforced concrete beam has effective cover d’ to the centre of compression reinforcement. ‘xu’ is the depth of neutral axis, and d is the effective depth to the centre of tension reinforcement. What is the maximum strain in concrete at the level of compression reinforcement?a)0.0035 (1 – d’/d)b)0.0035 (1 – d’/xu)c)0.002 (1 – d’/xu)d)0.002 (1 – d’/d)Correct answer is option 'B'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice A doubly reinforced concrete beam has effective cover d’ to the centre of compression reinforcement. ‘xu’ is the depth of neutral axis, and d is the effective depth to the centre of tension reinforcement. What is the maximum strain in concrete at the level of compression reinforcement?a)0.0035 (1 – d’/d)b)0.0035 (1 – d’/xu)c)0.002 (1 – d’/xu)d)0.002 (1 – d’/d)Correct answer is option 'B'. Can you explain this answer? tests, examples and also practice Civil Engineering (CE) tests.
|
|
Explore Courses for Civil Engineering (CE) exam
|
|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
|
© EduRev
|
Education Revolution
|
|
Signup to see your scores
go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup