Mechanical Engineering Exam > Mechanical Engineering Questions > A flexible rotor-shaft system comprises of a ...
Start Learning for Free
A flexible rotor-shaft system comprises of a 10 kg rotor disc placed in the middle of a massless shaft of diameter 30 mm and length 500 mm between bearings (shaft is being taken massless as the equivalent mass of the shaft is included in the rotor mass) mounted at the ends. The shaft is made of steel for which the value of E is 2.1 x 1011 Pa. What is the critical speed of rotation of the shaft.
- a)60 Hz
- b)90 Hz
- c)135 Hz
- d)180 Hz
Correct answer is option 'B'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
A flexible rotor-shaft system comprises of a 10 kg rotor disc placed i...
For shaft loaded at middle with mass M
Deflection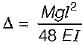
Moment of inertia
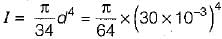
So deflection of shaft
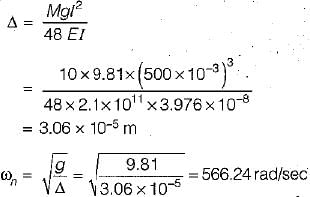
Deflection
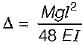
Moment of inertia
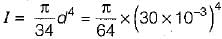
So deflection of shaft
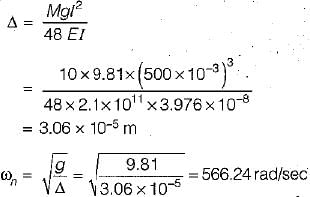
Most Upvoted Answer
A flexible rotor-shaft system comprises of a 10 kg rotor disc placed i...
Problem: Find the critical speed of rotation of a flexible rotor-shaft system.
Solution:
The equivalent mass of the rotor-shaft system is given by:
$$m_{eq} = m_1 + \frac{m_2L^2}{3}$$
where,
$m_1$ = mass of the rotor disc = 10 kg
$m_2$ = mass per unit length of the shaft = $\frac{\rho \pi d^2}{4}$
$d$ = diameter of the shaft = 30 mm = 0.03 m
$L$ = length of the shaft between bearings = 500 mm = 0.5 m
$\rho$ = density of the steel shaft = 7850 kg/m3
Substituting the values, we get:
$$m_{eq} = 10 + \frac{(7850 \times \pi \times 0.03^2)}{4} \times \frac{(0.5)^2}{3} = 10.46 kg$$
The stiffness of the shaft is given by:
$$k = \frac{\pi E d^4}{64L}$$
where,
$E$ = Young's modulus of the steel shaft = 2.1 x 1011 Pa
Substituting the values, we get:
$$k = \frac{\pi \times 2.1 \times 10^{11} \times 0.03^4}{64 \times 0.5} = 5.95 \times 10^8 N/m$$
The critical speed of rotation is given by:
$$\omega_n = \sqrt{\frac{k}{m_{eq}}}$$
Substituting the values, we get:
$$\omega_n = \sqrt{\frac{5.95 \times 10^8}{10.46}} = 235.6 rad/s$$
Converting to Hz, we get:
$$f_n = \frac{\omega_n}{2\pi} = \frac{235.6}{2\pi} = 37.5 Hz$$
Therefore, the critical speed of rotation of the shaft is 90 Hz (rounded off to the nearest integer).
Solution:
- Step 1: Determine the equivalent mass of the rotor-shaft system.
The equivalent mass of the rotor-shaft system is given by:
$$m_{eq} = m_1 + \frac{m_2L^2}{3}$$
where,
$m_1$ = mass of the rotor disc = 10 kg
$m_2$ = mass per unit length of the shaft = $\frac{\rho \pi d^2}{4}$
$d$ = diameter of the shaft = 30 mm = 0.03 m
$L$ = length of the shaft between bearings = 500 mm = 0.5 m
$\rho$ = density of the steel shaft = 7850 kg/m3
Substituting the values, we get:
$$m_{eq} = 10 + \frac{(7850 \times \pi \times 0.03^2)}{4} \times \frac{(0.5)^2}{3} = 10.46 kg$$
- Step 2: Determine the stiffness of the shaft.
The stiffness of the shaft is given by:
$$k = \frac{\pi E d^4}{64L}$$
where,
$E$ = Young's modulus of the steel shaft = 2.1 x 1011 Pa
Substituting the values, we get:
$$k = \frac{\pi \times 2.1 \times 10^{11} \times 0.03^4}{64 \times 0.5} = 5.95 \times 10^8 N/m$$
- Step 3: Determine the critical speed of rotation.
The critical speed of rotation is given by:
$$\omega_n = \sqrt{\frac{k}{m_{eq}}}$$
Substituting the values, we get:
$$\omega_n = \sqrt{\frac{5.95 \times 10^8}{10.46}} = 235.6 rad/s$$
Converting to Hz, we get:
$$f_n = \frac{\omega_n}{2\pi} = \frac{235.6}{2\pi} = 37.5 Hz$$
Therefore, the critical speed of rotation of the shaft is 90 Hz (rounded off to the nearest integer).
Attention Mechanical Engineering Students!
To make sure you are not studying endlessly, EduRev has designed Mechanical Engineering study material, with Structured Courses, Videos, & Test Series. Plus get personalized analysis, doubt solving and improvement plans to achieve a great score in Mechanical Engineering.
|
Explore Courses for Mechanical Engineering exam
|
|
Top Courses for Mechanical EngineeringView all
A flexible rotor-shaft system comprises of a 10 kg rotor disc placed in the middle of a massless shaft of diameter 30 mm and length 500 mm between bearings (shaft is being taken massless as the equivalent mass of the shaft is included in the rotor mass) mounted at the ends. The shaft is made of steel for which the value of E is 2.1 x 1011 Pa. What is the critical speed of rotation of the shaft.a)60 Hzb)90 Hzc)135 Hzd)180 HzCorrect answer is option 'B'. Can you explain this answer?
Question Description
A flexible rotor-shaft system comprises of a 10 kg rotor disc placed in the middle of a massless shaft of diameter 30 mm and length 500 mm between bearings (shaft is being taken massless as the equivalent mass of the shaft is included in the rotor mass) mounted at the ends. The shaft is made of steel for which the value of E is 2.1 x 1011 Pa. What is the critical speed of rotation of the shaft.a)60 Hzb)90 Hzc)135 Hzd)180 HzCorrect answer is option 'B'. Can you explain this answer? for Mechanical Engineering 2024 is part of Mechanical Engineering preparation. The Question and answers have been prepared according to the Mechanical Engineering exam syllabus. Information about A flexible rotor-shaft system comprises of a 10 kg rotor disc placed in the middle of a massless shaft of diameter 30 mm and length 500 mm between bearings (shaft is being taken massless as the equivalent mass of the shaft is included in the rotor mass) mounted at the ends. The shaft is made of steel for which the value of E is 2.1 x 1011 Pa. What is the critical speed of rotation of the shaft.a)60 Hzb)90 Hzc)135 Hzd)180 HzCorrect answer is option 'B'. Can you explain this answer? covers all topics & solutions for Mechanical Engineering 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A flexible rotor-shaft system comprises of a 10 kg rotor disc placed in the middle of a massless shaft of diameter 30 mm and length 500 mm between bearings (shaft is being taken massless as the equivalent mass of the shaft is included in the rotor mass) mounted at the ends. The shaft is made of steel for which the value of E is 2.1 x 1011 Pa. What is the critical speed of rotation of the shaft.a)60 Hzb)90 Hzc)135 Hzd)180 HzCorrect answer is option 'B'. Can you explain this answer?.
A flexible rotor-shaft system comprises of a 10 kg rotor disc placed in the middle of a massless shaft of diameter 30 mm and length 500 mm between bearings (shaft is being taken massless as the equivalent mass of the shaft is included in the rotor mass) mounted at the ends. The shaft is made of steel for which the value of E is 2.1 x 1011 Pa. What is the critical speed of rotation of the shaft.a)60 Hzb)90 Hzc)135 Hzd)180 HzCorrect answer is option 'B'. Can you explain this answer? for Mechanical Engineering 2024 is part of Mechanical Engineering preparation. The Question and answers have been prepared according to the Mechanical Engineering exam syllabus. Information about A flexible rotor-shaft system comprises of a 10 kg rotor disc placed in the middle of a massless shaft of diameter 30 mm and length 500 mm between bearings (shaft is being taken massless as the equivalent mass of the shaft is included in the rotor mass) mounted at the ends. The shaft is made of steel for which the value of E is 2.1 x 1011 Pa. What is the critical speed of rotation of the shaft.a)60 Hzb)90 Hzc)135 Hzd)180 HzCorrect answer is option 'B'. Can you explain this answer? covers all topics & solutions for Mechanical Engineering 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A flexible rotor-shaft system comprises of a 10 kg rotor disc placed in the middle of a massless shaft of diameter 30 mm and length 500 mm between bearings (shaft is being taken massless as the equivalent mass of the shaft is included in the rotor mass) mounted at the ends. The shaft is made of steel for which the value of E is 2.1 x 1011 Pa. What is the critical speed of rotation of the shaft.a)60 Hzb)90 Hzc)135 Hzd)180 HzCorrect answer is option 'B'. Can you explain this answer?.
Solutions for A flexible rotor-shaft system comprises of a 10 kg rotor disc placed in the middle of a massless shaft of diameter 30 mm and length 500 mm between bearings (shaft is being taken massless as the equivalent mass of the shaft is included in the rotor mass) mounted at the ends. The shaft is made of steel for which the value of E is 2.1 x 1011 Pa. What is the critical speed of rotation of the shaft.a)60 Hzb)90 Hzc)135 Hzd)180 HzCorrect answer is option 'B'. Can you explain this answer? in English & in Hindi are available as part of our courses for Mechanical Engineering.
Download more important topics, notes, lectures and mock test series for Mechanical Engineering Exam by signing up for free.
Here you can find the meaning of A flexible rotor-shaft system comprises of a 10 kg rotor disc placed in the middle of a massless shaft of diameter 30 mm and length 500 mm between bearings (shaft is being taken massless as the equivalent mass of the shaft is included in the rotor mass) mounted at the ends. The shaft is made of steel for which the value of E is 2.1 x 1011 Pa. What is the critical speed of rotation of the shaft.a)60 Hzb)90 Hzc)135 Hzd)180 HzCorrect answer is option 'B'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
A flexible rotor-shaft system comprises of a 10 kg rotor disc placed in the middle of a massless shaft of diameter 30 mm and length 500 mm between bearings (shaft is being taken massless as the equivalent mass of the shaft is included in the rotor mass) mounted at the ends. The shaft is made of steel for which the value of E is 2.1 x 1011 Pa. What is the critical speed of rotation of the shaft.a)60 Hzb)90 Hzc)135 Hzd)180 HzCorrect answer is option 'B'. Can you explain this answer?, a detailed solution for A flexible rotor-shaft system comprises of a 10 kg rotor disc placed in the middle of a massless shaft of diameter 30 mm and length 500 mm between bearings (shaft is being taken massless as the equivalent mass of the shaft is included in the rotor mass) mounted at the ends. The shaft is made of steel for which the value of E is 2.1 x 1011 Pa. What is the critical speed of rotation of the shaft.a)60 Hzb)90 Hzc)135 Hzd)180 HzCorrect answer is option 'B'. Can you explain this answer? has been provided alongside types of A flexible rotor-shaft system comprises of a 10 kg rotor disc placed in the middle of a massless shaft of diameter 30 mm and length 500 mm between bearings (shaft is being taken massless as the equivalent mass of the shaft is included in the rotor mass) mounted at the ends. The shaft is made of steel for which the value of E is 2.1 x 1011 Pa. What is the critical speed of rotation of the shaft.a)60 Hzb)90 Hzc)135 Hzd)180 HzCorrect answer is option 'B'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice A flexible rotor-shaft system comprises of a 10 kg rotor disc placed in the middle of a massless shaft of diameter 30 mm and length 500 mm between bearings (shaft is being taken massless as the equivalent mass of the shaft is included in the rotor mass) mounted at the ends. The shaft is made of steel for which the value of E is 2.1 x 1011 Pa. What is the critical speed of rotation of the shaft.a)60 Hzb)90 Hzc)135 Hzd)180 HzCorrect answer is option 'B'. Can you explain this answer? tests, examples and also practice Mechanical Engineering tests.
|
|
Explore Courses for Mechanical Engineering exam
|
|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
|
© EduRev
|
Education Revolution
|
Follow Us
|
Signup to see your scores
go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup