IIT JAM Exam > IIT JAM Questions > Four moles of a radioactive material decays i...
Start Learning for Free
Four moles of a radioactive material decays into 0.5 moles in 90 seconds. Then the disintegration constant is equal to ______ x 10-2s-1 (upto two decimal places)
Correct answer is between '2.30,2.32'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
Four moles of a radioactive material decays into 0.5 moles in 90 secon...
Initial number of nuclei = 4 moles x NA , where NA is Avogadro nuniber.
Final number of nuclei = 0.5 moles x NA
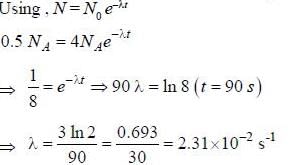
Final number of nuclei = 0.5 moles x NA
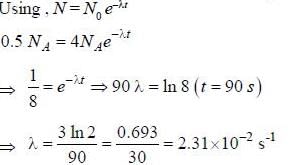
Most Upvoted Answer
Four moles of a radioactive material decays into 0.5 moles in 90 secon...
The disintegration constant, also known as the decay constant, is a measure of how quickly a radioactive material decays. It represents the probability of decay per unit time. To calculate the disintegration constant, we need to use the formula:
N = N0 * e^(-λt)
Where:
N = Final number of moles
N0 = Initial number of moles
λ = Disintegration constant
t = Time
Given that four moles of a radioactive material decays into 0.5 moles in 90 seconds, we can use this information to calculate the disintegration constant.
Step 1: Convert the number of moles into fractions of moles
Since the disintegration constant represents a probability, we need to convert the number of moles into fractions of moles. In this case, we have:
N0 = 4 moles
N = 0.5 moles
Step 2: Rearrange the formula to solve for the disintegration constant
Rearranging the formula, we have:
λ = -ln(N/N0) / t
Step 3: Substitute the given values into the formula
Substituting the given values into the formula, we have:
λ = -ln(0.5/4) / 90
Step 4: Calculate the disintegration constant
Using a calculator, we can evaluate the natural logarithm and divide it by 90 to find the disintegration constant. The correct answer is between 2.30 and 2.32 x 10^-2 s^-1.
In conclusion, the disintegration constant is calculated by using the formula N = N0 * e^(-λt), where N and N0 represent the final and initial number of moles, λ is the disintegration constant, and t is the time. By rearranging the formula and substituting the given values, we can calculate the disintegration constant. In this case, four moles of a radioactive material decaying into 0.5 moles in 90 seconds gives a disintegration constant between 2.30 and 2.32 x 10^-2 s^-1.
N = N0 * e^(-λt)
Where:
N = Final number of moles
N0 = Initial number of moles
λ = Disintegration constant
t = Time
Given that four moles of a radioactive material decays into 0.5 moles in 90 seconds, we can use this information to calculate the disintegration constant.
Step 1: Convert the number of moles into fractions of moles
Since the disintegration constant represents a probability, we need to convert the number of moles into fractions of moles. In this case, we have:
N0 = 4 moles
N = 0.5 moles
Step 2: Rearrange the formula to solve for the disintegration constant
Rearranging the formula, we have:
λ = -ln(N/N0) / t
Step 3: Substitute the given values into the formula
Substituting the given values into the formula, we have:
λ = -ln(0.5/4) / 90
Step 4: Calculate the disintegration constant
Using a calculator, we can evaluate the natural logarithm and divide it by 90 to find the disintegration constant. The correct answer is between 2.30 and 2.32 x 10^-2 s^-1.
In conclusion, the disintegration constant is calculated by using the formula N = N0 * e^(-λt), where N and N0 represent the final and initial number of moles, λ is the disintegration constant, and t is the time. By rearranging the formula and substituting the given values, we can calculate the disintegration constant. In this case, four moles of a radioactive material decaying into 0.5 moles in 90 seconds gives a disintegration constant between 2.30 and 2.32 x 10^-2 s^-1.

|
Explore Courses for IIT JAM exam
|

|
Similar IIT JAM Doubts
Four moles of a radioactive material decays into 0.5 moles in 90 seconds. Then the disintegration constant is equal to ______x 10-2s-1 (upto two decimal places)Correct answer is between '2.30,2.32'. Can you explain this answer?
Question Description
Four moles of a radioactive material decays into 0.5 moles in 90 seconds. Then the disintegration constant is equal to ______x 10-2s-1 (upto two decimal places)Correct answer is between '2.30,2.32'. Can you explain this answer? for IIT JAM 2024 is part of IIT JAM preparation. The Question and answers have been prepared according to the IIT JAM exam syllabus. Information about Four moles of a radioactive material decays into 0.5 moles in 90 seconds. Then the disintegration constant is equal to ______x 10-2s-1 (upto two decimal places)Correct answer is between '2.30,2.32'. Can you explain this answer? covers all topics & solutions for IIT JAM 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Four moles of a radioactive material decays into 0.5 moles in 90 seconds. Then the disintegration constant is equal to ______x 10-2s-1 (upto two decimal places)Correct answer is between '2.30,2.32'. Can you explain this answer?.
Four moles of a radioactive material decays into 0.5 moles in 90 seconds. Then the disintegration constant is equal to ______x 10-2s-1 (upto two decimal places)Correct answer is between '2.30,2.32'. Can you explain this answer? for IIT JAM 2024 is part of IIT JAM preparation. The Question and answers have been prepared according to the IIT JAM exam syllabus. Information about Four moles of a radioactive material decays into 0.5 moles in 90 seconds. Then the disintegration constant is equal to ______x 10-2s-1 (upto two decimal places)Correct answer is between '2.30,2.32'. Can you explain this answer? covers all topics & solutions for IIT JAM 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Four moles of a radioactive material decays into 0.5 moles in 90 seconds. Then the disintegration constant is equal to ______x 10-2s-1 (upto two decimal places)Correct answer is between '2.30,2.32'. Can you explain this answer?.
Solutions for Four moles of a radioactive material decays into 0.5 moles in 90 seconds. Then the disintegration constant is equal to ______x 10-2s-1 (upto two decimal places)Correct answer is between '2.30,2.32'. Can you explain this answer? in English & in Hindi are available as part of our courses for IIT JAM.
Download more important topics, notes, lectures and mock test series for IIT JAM Exam by signing up for free.
Here you can find the meaning of Four moles of a radioactive material decays into 0.5 moles in 90 seconds. Then the disintegration constant is equal to ______x 10-2s-1 (upto two decimal places)Correct answer is between '2.30,2.32'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
Four moles of a radioactive material decays into 0.5 moles in 90 seconds. Then the disintegration constant is equal to ______x 10-2s-1 (upto two decimal places)Correct answer is between '2.30,2.32'. Can you explain this answer?, a detailed solution for Four moles of a radioactive material decays into 0.5 moles in 90 seconds. Then the disintegration constant is equal to ______x 10-2s-1 (upto two decimal places)Correct answer is between '2.30,2.32'. Can you explain this answer? has been provided alongside types of Four moles of a radioactive material decays into 0.5 moles in 90 seconds. Then the disintegration constant is equal to ______x 10-2s-1 (upto two decimal places)Correct answer is between '2.30,2.32'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice Four moles of a radioactive material decays into 0.5 moles in 90 seconds. Then the disintegration constant is equal to ______x 10-2s-1 (upto two decimal places)Correct answer is between '2.30,2.32'. Can you explain this answer? tests, examples and also practice IIT JAM tests.

|
Explore Courses for IIT JAM exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.


















