IIT JAM Exam > IIT JAM Questions > A current of 0.5 A is passed through molten A...
Start Learning for Free
A current of 0.5 A is passed through molten AlCl3 for 40 min the mass of aluminium deposited at the cathode is _____ g (Al = 27)
Correct answer is between '0.110,0.115'. Can you explain this answer?
Verified Answer
A current of 0.5 A is passed through molten AlCl3for 40 min the mass o...
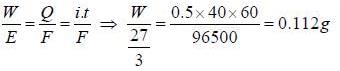
Most Upvoted Answer
A current of 0.5 A is passed through molten AlCl3for 40 min the mass o...
To calculate the mass of aluminum deposited at the cathode during electrolysis, we can use Faraday's laws of electrolysis. Here’s a detailed breakdown:
1. Understanding the Reaction
- In the electrolysis of molten AlCl3, aluminum ions (Al³⁺) gain electrons to form aluminum metal:
\[ \text{Al}^{3+} + 3e^- \rightarrow \text{Al} \]
2. Determine Charge Passed
- To find out how much charge is passed, use the formula:
\[ Q = I \times t \]
where:
- \( I = 0.5 \, A \) (current)
- \( t = 40 \, \text{min} = 40 \times 60 = 2400 \, \text{s} \)
- Calculating the charge:
\[ Q = 0.5 \, A \times 2400 \, s = 1200 \, C \]
3. Calculate Moles of Electrons
- Using Faraday's constant (\( F \approx 96500 \, C/mol \)), calculate the moles of electrons:
\[ n_{e^-} = \frac{Q}{F} = \frac{1200}{96500} \approx 0.01242 \, mol \]
4. Relate Moles of Aluminum to Moles of Electrons
- From the reaction, 3 moles of electrons are required to deposit 1 mole of aluminum:
\[ n_{Al} = \frac{n_{e^-}}{3} = \frac{0.01242}{3} \approx 0.00414 \, mol \]
5. Calculate Mass of Aluminum
- Finally, using the molar mass of aluminum (27 g/mol):
\[ \text{mass} = n_{Al} \times \text{molar mass} = 0.00414 \times 27 \approx 0.11178 \, g \]
Thus, the mass of aluminum deposited at the cathode is approximately 0.112 g, which falls within the specified range of 0.110 to 0.115 g.
1. Understanding the Reaction
- In the electrolysis of molten AlCl3, aluminum ions (Al³⁺) gain electrons to form aluminum metal:
\[ \text{Al}^{3+} + 3e^- \rightarrow \text{Al} \]
2. Determine Charge Passed
- To find out how much charge is passed, use the formula:
\[ Q = I \times t \]
where:
- \( I = 0.5 \, A \) (current)
- \( t = 40 \, \text{min} = 40 \times 60 = 2400 \, \text{s} \)
- Calculating the charge:
\[ Q = 0.5 \, A \times 2400 \, s = 1200 \, C \]
3. Calculate Moles of Electrons
- Using Faraday's constant (\( F \approx 96500 \, C/mol \)), calculate the moles of electrons:
\[ n_{e^-} = \frac{Q}{F} = \frac{1200}{96500} \approx 0.01242 \, mol \]
4. Relate Moles of Aluminum to Moles of Electrons
- From the reaction, 3 moles of electrons are required to deposit 1 mole of aluminum:
\[ n_{Al} = \frac{n_{e^-}}{3} = \frac{0.01242}{3} \approx 0.00414 \, mol \]
5. Calculate Mass of Aluminum
- Finally, using the molar mass of aluminum (27 g/mol):
\[ \text{mass} = n_{Al} \times \text{molar mass} = 0.00414 \times 27 \approx 0.11178 \, g \]
Thus, the mass of aluminum deposited at the cathode is approximately 0.112 g, which falls within the specified range of 0.110 to 0.115 g.

|
Explore Courses for IIT JAM exam
|

|
Question Description
A current of 0.5 A is passed through molten AlCl3for 40 min the mass of aluminium deposited at the cathode is _____g (Al = 27)Correct answer is between '0.110,0.115'. Can you explain this answer? for IIT JAM 2025 is part of IIT JAM preparation. The Question and answers have been prepared according to the IIT JAM exam syllabus. Information about A current of 0.5 A is passed through molten AlCl3for 40 min the mass of aluminium deposited at the cathode is _____g (Al = 27)Correct answer is between '0.110,0.115'. Can you explain this answer? covers all topics & solutions for IIT JAM 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A current of 0.5 A is passed through molten AlCl3for 40 min the mass of aluminium deposited at the cathode is _____g (Al = 27)Correct answer is between '0.110,0.115'. Can you explain this answer?.
A current of 0.5 A is passed through molten AlCl3for 40 min the mass of aluminium deposited at the cathode is _____g (Al = 27)Correct answer is between '0.110,0.115'. Can you explain this answer? for IIT JAM 2025 is part of IIT JAM preparation. The Question and answers have been prepared according to the IIT JAM exam syllabus. Information about A current of 0.5 A is passed through molten AlCl3for 40 min the mass of aluminium deposited at the cathode is _____g (Al = 27)Correct answer is between '0.110,0.115'. Can you explain this answer? covers all topics & solutions for IIT JAM 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A current of 0.5 A is passed through molten AlCl3for 40 min the mass of aluminium deposited at the cathode is _____g (Al = 27)Correct answer is between '0.110,0.115'. Can you explain this answer?.
Solutions for A current of 0.5 A is passed through molten AlCl3for 40 min the mass of aluminium deposited at the cathode is _____g (Al = 27)Correct answer is between '0.110,0.115'. Can you explain this answer? in English & in Hindi are available as part of our courses for IIT JAM.
Download more important topics, notes, lectures and mock test series for IIT JAM Exam by signing up for free.
Here you can find the meaning of A current of 0.5 A is passed through molten AlCl3for 40 min the mass of aluminium deposited at the cathode is _____g (Al = 27)Correct answer is between '0.110,0.115'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
A current of 0.5 A is passed through molten AlCl3for 40 min the mass of aluminium deposited at the cathode is _____g (Al = 27)Correct answer is between '0.110,0.115'. Can you explain this answer?, a detailed solution for A current of 0.5 A is passed through molten AlCl3for 40 min the mass of aluminium deposited at the cathode is _____g (Al = 27)Correct answer is between '0.110,0.115'. Can you explain this answer? has been provided alongside types of A current of 0.5 A is passed through molten AlCl3for 40 min the mass of aluminium deposited at the cathode is _____g (Al = 27)Correct answer is between '0.110,0.115'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice A current of 0.5 A is passed through molten AlCl3for 40 min the mass of aluminium deposited at the cathode is _____g (Al = 27)Correct answer is between '0.110,0.115'. Can you explain this answer? tests, examples and also practice IIT JAM tests.

|
Explore Courses for IIT JAM exam
|

|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.























