Class 8 Exam > Class 8 Questions > find the square root of the following by the ...
Start Learning for Free
find the square root of the following by the long division method 9801
?Most Upvoted Answer
find the square root of the following by the long division method 9801...
Long Division Method for Finding Square Roots of Numbers
The long division method is a simple and systematic way of finding the square root of a number. Here are the steps to follow:
1. Start by grouping the digits of the given number in pairs, starting from the rightmost digit. If there are any remaining digits, add a zero to the left of the first digit to form a pair.
2. Find the largest perfect square that is less than or equal to the first pair of digits. Write the square root of this number as the first digit of the answer.
3. Subtract the product of the digit and the first digit of the answer from the first pair of digits. Bring down the next pair of digits and place them next to the remainder.
4. Double the first digit of the answer, and then find the largest digit that can be placed in the second position such that the product of this digit and twice the first digit is less than or equal to the new dividend.
5. Write this digit as the second digit of the answer. Subtract the product of this digit and twice the first digit from the new dividend, and bring down the next pair of digits.
6. Repeat steps 4 and 5 until all the digits have been brought down.
7. The final answer is the square root of the given number.
Finding the Square Root of 9801 Using the Long Division Method
1. Group the digits of the number in pairs: 98 and 01.
2. Find the largest perfect square that is less than or equal to 98, which is 81. Write the square root of 81 as the first digit of the answer, which is 9.
3. Subtract the product of 9 and 9 from 98, which gives a remainder of 17. Bring down the next pair of digits, which gives a new dividend of 170.
4. Double the first digit of the answer, which is 9, to get 18. Find the largest digit that can be placed in the second position such that the product of this digit and twice the first digit is less than or equal to 170. This digit is 8, so write it as the second digit of the answer.
5. Subtract the product of 8 and 18 from 170, which gives a remainder of 14. Bring down the next pair of digits, which gives a new dividend of 141.
6. Double the first two digits of the answer, which gives 96. Find the largest digit that can be placed in the third position such that the product of this digit and 96 is less than or equal to 141. This digit is 1, so write it as the third digit of the answer.
7. Subtract the product of 1 and 196 from 141, which gives a remainder of 45. Bring down the next pair of digits, which gives a new dividend of 450.
8. Double the first three digits of the answer, which gives 196. Find the largest digit that can be placed in the fourth position such that the product of this digit and 196 is less than or equal to 450. This digit is 2, so write it as the fourth digit of the answer.
9. Subtract the product of 2 and 196 from 450, which gives a remainder of 58. Bring down the next pair of digits, which gives a
The long division method is a simple and systematic way of finding the square root of a number. Here are the steps to follow:
1. Start by grouping the digits of the given number in pairs, starting from the rightmost digit. If there are any remaining digits, add a zero to the left of the first digit to form a pair.
2. Find the largest perfect square that is less than or equal to the first pair of digits. Write the square root of this number as the first digit of the answer.
3. Subtract the product of the digit and the first digit of the answer from the first pair of digits. Bring down the next pair of digits and place them next to the remainder.
4. Double the first digit of the answer, and then find the largest digit that can be placed in the second position such that the product of this digit and twice the first digit is less than or equal to the new dividend.
5. Write this digit as the second digit of the answer. Subtract the product of this digit and twice the first digit from the new dividend, and bring down the next pair of digits.
6. Repeat steps 4 and 5 until all the digits have been brought down.
7. The final answer is the square root of the given number.
Finding the Square Root of 9801 Using the Long Division Method
1. Group the digits of the number in pairs: 98 and 01.
2. Find the largest perfect square that is less than or equal to 98, which is 81. Write the square root of 81 as the first digit of the answer, which is 9.
3. Subtract the product of 9 and 9 from 98, which gives a remainder of 17. Bring down the next pair of digits, which gives a new dividend of 170.
4. Double the first digit of the answer, which is 9, to get 18. Find the largest digit that can be placed in the second position such that the product of this digit and twice the first digit is less than or equal to 170. This digit is 8, so write it as the second digit of the answer.
5. Subtract the product of 8 and 18 from 170, which gives a remainder of 14. Bring down the next pair of digits, which gives a new dividend of 141.
6. Double the first two digits of the answer, which gives 96. Find the largest digit that can be placed in the third position such that the product of this digit and 96 is less than or equal to 141. This digit is 1, so write it as the third digit of the answer.
7. Subtract the product of 1 and 196 from 141, which gives a remainder of 45. Bring down the next pair of digits, which gives a new dividend of 450.
8. Double the first three digits of the answer, which gives 196. Find the largest digit that can be placed in the fourth position such that the product of this digit and 196 is less than or equal to 450. This digit is 2, so write it as the fourth digit of the answer.
9. Subtract the product of 2 and 196 from 450, which gives a remainder of 58. Bring down the next pair of digits, which gives a
Community Answer
find the square root of the following by the long division method 9801...
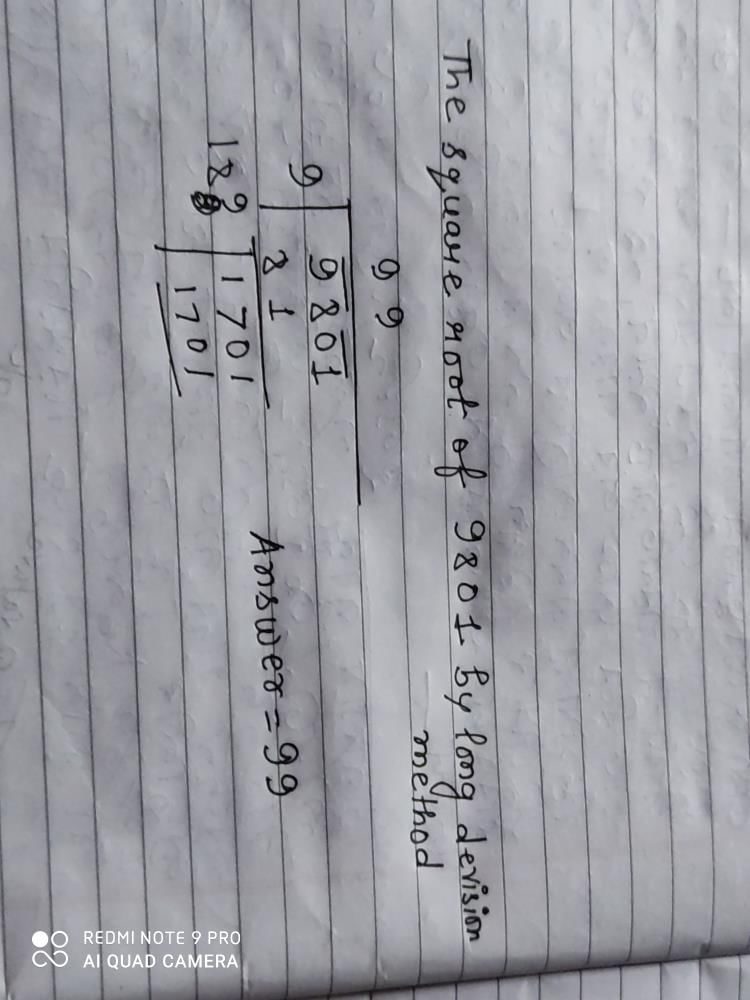
Attention Class 8 Students!
To make sure you are not studying endlessly, EduRev has designed Class 8 study material, with Structured Courses, Videos, & Test Series. Plus get personalized analysis, doubt solving and improvement plans to achieve a great score in Class 8.

|
Explore Courses for Class 8 exam
|

|
Similar Class 8 Doubts
find the square root of the following by the long division method 9801 Related: Points to Remember- Squares and Square Roots?
Question Description
find the square root of the following by the long division method 9801 Related: Points to Remember- Squares and Square Roots? for Class 8 2024 is part of Class 8 preparation. The Question and answers have been prepared according to the Class 8 exam syllabus. Information about find the square root of the following by the long division method 9801 Related: Points to Remember- Squares and Square Roots? covers all topics & solutions for Class 8 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for find the square root of the following by the long division method 9801 Related: Points to Remember- Squares and Square Roots?.
find the square root of the following by the long division method 9801 Related: Points to Remember- Squares and Square Roots? for Class 8 2024 is part of Class 8 preparation. The Question and answers have been prepared according to the Class 8 exam syllabus. Information about find the square root of the following by the long division method 9801 Related: Points to Remember- Squares and Square Roots? covers all topics & solutions for Class 8 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for find the square root of the following by the long division method 9801 Related: Points to Remember- Squares and Square Roots?.
Solutions for find the square root of the following by the long division method 9801 Related: Points to Remember- Squares and Square Roots? in English & in Hindi are available as part of our courses for Class 8.
Download more important topics, notes, lectures and mock test series for Class 8 Exam by signing up for free.
Here you can find the meaning of find the square root of the following by the long division method 9801 Related: Points to Remember- Squares and Square Roots? defined & explained in the simplest way possible. Besides giving the explanation of
find the square root of the following by the long division method 9801 Related: Points to Remember- Squares and Square Roots?, a detailed solution for find the square root of the following by the long division method 9801 Related: Points to Remember- Squares and Square Roots? has been provided alongside types of find the square root of the following by the long division method 9801 Related: Points to Remember- Squares and Square Roots? theory, EduRev gives you an
ample number of questions to practice find the square root of the following by the long division method 9801 Related: Points to Remember- Squares and Square Roots? tests, examples and also practice Class 8 tests.

|
Explore Courses for Class 8 exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.

























