Class 12 Exam > Class 12 Questions > The dimensions of permeability of free space ...
Start Learning for Free
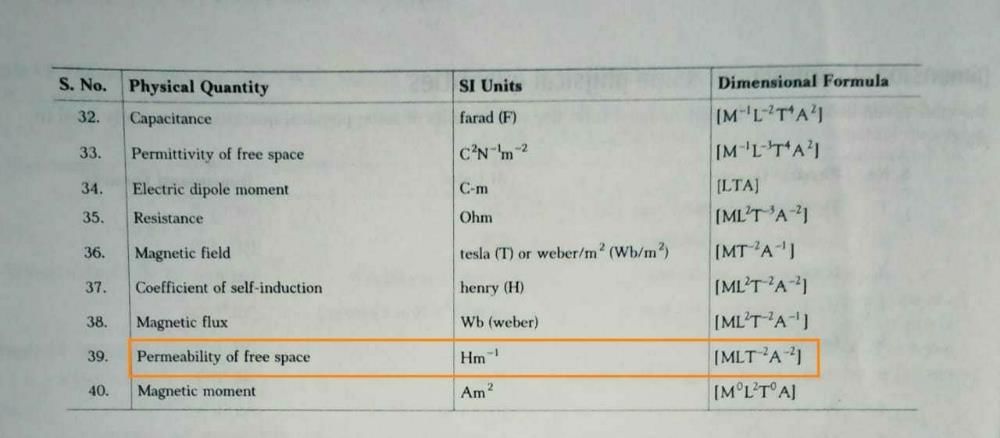
The dimensions of permeability of free space can be given by
- a)[MLT-2A-2]
- b)[MLA-2]
- c)[ML-3T2A2]
- d)[MLA-1]
Correct answer is option 'A'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
The dimensions of permeability of free space can be given bya)[MLT-2A-...
In SI units, permeability is measured in Henries per meter H/m or Hm−1.
Henry has the dimensions of [ML2T−2A−2].
Dimensions for magnetic permeability will be [ML2T−2A−2]/[L]=[MLT−2A−2]
Henry has the dimensions of [ML2T−2A−2].
Dimensions for magnetic permeability will be [ML2T−2A−2]/[L]=[MLT−2A−2]
Most Upvoted Answer
The dimensions of permeability of free space can be given bya)[MLT-2A-...
Free Test
FREE
| Start Free Test |
Community Answer
The dimensions of permeability of free space can be given bya)[MLT-2A-...
The dimensions of permeability of free space can be given by [MLT-2A-2]. Let's break down the answer and understand each component in detail.
[MLT-2A-2]
M - Represents the dimension of mass. In this case, the permeability of free space is not dependent on mass, so the dimension of mass is not present.
L - Represents the dimension of length. The permeability of free space is related to the distance or length scale. It indicates the ability of a material to allow the passage of magnetic field lines. In this case, the dimension of length is present.
T - Represents the dimension of time. The permeability of free space is not dependent on time, so the dimension of time is not present.
A - Represents the dimension of electric current. The permeability of free space is related to the ability of a material to allow the passage of electric current. In this case, the dimension of electric current is present.
-2 - Represents the exponent of the dimension. In this case, it indicates that the dimension is raised to the power of -2.
Explanation:
The permeability of free space, denoted by the symbol μ₀, is a fundamental constant in physics. It is a measure of the ability of a material to allow the passage of magnetic field lines. It is related to the magnetic properties of a material and is an important parameter in electromagnetic theory.
The dimensions of permeability can be determined by analyzing its units. In the International System of Units (SI), the unit of permeability is tesla meter per ampere (T·m/A). By breaking down the units, we can determine the dimensions.
The unit of magnetic field strength is tesla (T), which is equivalent to kg·s⁻²·A⁻¹ (kilogram per second squared per ampere). The unit of length is meter (m), the unit of time is second (s), and the unit of electric current is ampere (A).
By analyzing the units, we can conclude that the dimensions of permeability are:
- Mass (M): Not present
- Length (L): Present
- Time (T): Not present
- Electric current (A): Present
The dimensions are raised to the power of -2, indicating that the permeability is inversely proportional to the square of the dimensions. Therefore, the dimensions of permeability of free space can be given by [MLT-2A-2].
In conclusion, the dimensions of permeability of free space are given by [MLT-2A-2], as it includes the dimensions of length and electric current raised to the power of -2.
[MLT-2A-2]
M - Represents the dimension of mass. In this case, the permeability of free space is not dependent on mass, so the dimension of mass is not present.
L - Represents the dimension of length. The permeability of free space is related to the distance or length scale. It indicates the ability of a material to allow the passage of magnetic field lines. In this case, the dimension of length is present.
T - Represents the dimension of time. The permeability of free space is not dependent on time, so the dimension of time is not present.
A - Represents the dimension of electric current. The permeability of free space is related to the ability of a material to allow the passage of electric current. In this case, the dimension of electric current is present.
-2 - Represents the exponent of the dimension. In this case, it indicates that the dimension is raised to the power of -2.
Explanation:
The permeability of free space, denoted by the symbol μ₀, is a fundamental constant in physics. It is a measure of the ability of a material to allow the passage of magnetic field lines. It is related to the magnetic properties of a material and is an important parameter in electromagnetic theory.
The dimensions of permeability can be determined by analyzing its units. In the International System of Units (SI), the unit of permeability is tesla meter per ampere (T·m/A). By breaking down the units, we can determine the dimensions.
The unit of magnetic field strength is tesla (T), which is equivalent to kg·s⁻²·A⁻¹ (kilogram per second squared per ampere). The unit of length is meter (m), the unit of time is second (s), and the unit of electric current is ampere (A).
By analyzing the units, we can conclude that the dimensions of permeability are:
- Mass (M): Not present
- Length (L): Present
- Time (T): Not present
- Electric current (A): Present
The dimensions are raised to the power of -2, indicating that the permeability is inversely proportional to the square of the dimensions. Therefore, the dimensions of permeability of free space can be given by [MLT-2A-2].
In conclusion, the dimensions of permeability of free space are given by [MLT-2A-2], as it includes the dimensions of length and electric current raised to the power of -2.

|
Explore Courses for Class 12 exam
|

|
Similar Class 12 Doubts
The dimensions of permeability of free space can be given bya)[MLT-2A-2]b)[MLA-2]c)[ML-3T2A2]d)[MLA-1]Correct answer is option 'A'. Can you explain this answer?
Question Description
The dimensions of permeability of free space can be given bya)[MLT-2A-2]b)[MLA-2]c)[ML-3T2A2]d)[MLA-1]Correct answer is option 'A'. Can you explain this answer? for Class 12 2024 is part of Class 12 preparation. The Question and answers have been prepared according to the Class 12 exam syllabus. Information about The dimensions of permeability of free space can be given bya)[MLT-2A-2]b)[MLA-2]c)[ML-3T2A2]d)[MLA-1]Correct answer is option 'A'. Can you explain this answer? covers all topics & solutions for Class 12 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for The dimensions of permeability of free space can be given bya)[MLT-2A-2]b)[MLA-2]c)[ML-3T2A2]d)[MLA-1]Correct answer is option 'A'. Can you explain this answer?.
The dimensions of permeability of free space can be given bya)[MLT-2A-2]b)[MLA-2]c)[ML-3T2A2]d)[MLA-1]Correct answer is option 'A'. Can you explain this answer? for Class 12 2024 is part of Class 12 preparation. The Question and answers have been prepared according to the Class 12 exam syllabus. Information about The dimensions of permeability of free space can be given bya)[MLT-2A-2]b)[MLA-2]c)[ML-3T2A2]d)[MLA-1]Correct answer is option 'A'. Can you explain this answer? covers all topics & solutions for Class 12 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for The dimensions of permeability of free space can be given bya)[MLT-2A-2]b)[MLA-2]c)[ML-3T2A2]d)[MLA-1]Correct answer is option 'A'. Can you explain this answer?.
Solutions for The dimensions of permeability of free space can be given bya)[MLT-2A-2]b)[MLA-2]c)[ML-3T2A2]d)[MLA-1]Correct answer is option 'A'. Can you explain this answer? in English & in Hindi are available as part of our courses for Class 12.
Download more important topics, notes, lectures and mock test series for Class 12 Exam by signing up for free.
Here you can find the meaning of The dimensions of permeability of free space can be given bya)[MLT-2A-2]b)[MLA-2]c)[ML-3T2A2]d)[MLA-1]Correct answer is option 'A'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
The dimensions of permeability of free space can be given bya)[MLT-2A-2]b)[MLA-2]c)[ML-3T2A2]d)[MLA-1]Correct answer is option 'A'. Can you explain this answer?, a detailed solution for The dimensions of permeability of free space can be given bya)[MLT-2A-2]b)[MLA-2]c)[ML-3T2A2]d)[MLA-1]Correct answer is option 'A'. Can you explain this answer? has been provided alongside types of The dimensions of permeability of free space can be given bya)[MLT-2A-2]b)[MLA-2]c)[ML-3T2A2]d)[MLA-1]Correct answer is option 'A'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice The dimensions of permeability of free space can be given bya)[MLT-2A-2]b)[MLA-2]c)[ML-3T2A2]d)[MLA-1]Correct answer is option 'A'. Can you explain this answer? tests, examples and also practice Class 12 tests.

|
Explore Courses for Class 12 exam
|

|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.


















