Class 11 Exam > Class 11 Questions > A cylindrical vessel of height 500 mm has an ...
Start Learning for Free
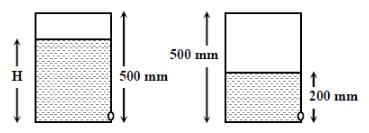
A cylindrical vessel of height 500 mm has an orifice (small hole) at its bottom. The orifice is initially closed and water is filled in it up to height H. Now the top is completely sealed with a cap and the orifice at the bottom is opened. Some water comes out from the orifice and the water level in the vessel becomes steady with height of water column being 200 mm. Find the fall in height (in mm) of water level due to opening of the orifice. [Take atmospheric pressure = 1.0 × 10 5 Nm-2 , density of water = 1000 kg m-3and g = 10 ms-2 . Neglect any effect of surface tension.] (Take temperature to be constant)
[JEE 2009]
Correct answer is '6'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
A cylindrical vessel of height 500 mm has an orifice (small hole) at i...
Pressure due to falling water level at 200mm is
P+ρgh=P0
or P=105−(1000)(10)(0.2)=98×103N/m2
now, P0V0=PV
or 105[A(0.5−H)]=98×103[A(0.5−0.2)]
where A= cross-sectional area of a vessel.
0.5−H=0.294
⇒H=0.206m=206mm
The fall in height (in mm) of water level =206−200=6
P+ρgh=P0
or P=105−(1000)(10)(0.2)=98×103N/m2
now, P0V0=PV
or 105[A(0.5−H)]=98×103[A(0.5−0.2)]
where A= cross-sectional area of a vessel.
0.5−H=0.294
⇒H=0.206m=206mm
The fall in height (in mm) of water level =206−200=6

Most Upvoted Answer
A cylindrical vessel of height 500 mm has an orifice (small hole) at i...
To solve this problem, we can use the principle of Pascal's law, which states that the pressure at any depth in a fluid is the same in all directions.
Initially, when the orifice is closed, the pressure at the bottom of the vessel is equal to the pressure at the top. This is because the weight of the water column above the orifice creates a pressure that is transmitted equally throughout the fluid.
When the orifice is opened, the pressure at the bottom of the vessel decreases due to the flow of water out of the orifice. This decrease in pressure causes the water level in the vessel to fall.
To find the fall in height of the water level, we can compare the pressure at the bottom of the vessel before and after the orifice is opened.
Before the orifice is opened, the pressure at the bottom of the vessel is equal to the pressure at the top, which is atmospheric pressure.
After the orifice is opened, the pressure at the bottom of the vessel is reduced due to the flow of water out of the orifice. This reduced pressure causes the water level to fall.
Using the equation for pressure in a fluid, we can write:
Pressure before = Pressure after
Atmospheric pressure = Pressure after
Since the height of the water column is directly proportional to the pressure at the bottom of the vessel, we can write:
Initial height / Final height = Atmospheric pressure / Pressure after
Substituting the given values, we have:
H / 200 = 1 / Pressure after
Solving for H, we find:
H = 200 / Pressure after
To determine the fall in height, we need to find the value of Pressure after. This can be calculated using the equation for pressure in a fluid:
Pressure after = Pressure before - pressure due to the height difference
Since the height difference is 300 mm (500 mm - 200 mm), we can write:
Pressure after = Atmospheric pressure - pressure due to the height difference
Substituting the given values, we have:
Pressure after = 1.0 - (density of water * acceleration due to gravity * height difference)
Since the density of water is approximately 1000 kg/m^3 and the acceleration due to gravity is approximately 9.8 m/s^2, we can convert the height difference to meters:
Height difference = 300 mm = 0.3 m
Substituting the values, we have:
Pressure after = 1.0 - (1000 kg/m^3 * 9.8 m/s^2 * 0.3 m)
Pressure after = 1.0 - 294 N/m^2
Pressure after = 706 N/m^2
Finally, substituting this value for Pressure after in the equation for H, we find:
H = 200 / 706
H ≈ 0.283 m
To convert this to mm, we multiply by 1000:
H ≈ 283 mm
Therefore, the fall in height of the water level due to opening the orifice is approximately 283 mm.
Initially, when the orifice is closed, the pressure at the bottom of the vessel is equal to the pressure at the top. This is because the weight of the water column above the orifice creates a pressure that is transmitted equally throughout the fluid.
When the orifice is opened, the pressure at the bottom of the vessel decreases due to the flow of water out of the orifice. This decrease in pressure causes the water level in the vessel to fall.
To find the fall in height of the water level, we can compare the pressure at the bottom of the vessel before and after the orifice is opened.
Before the orifice is opened, the pressure at the bottom of the vessel is equal to the pressure at the top, which is atmospheric pressure.
After the orifice is opened, the pressure at the bottom of the vessel is reduced due to the flow of water out of the orifice. This reduced pressure causes the water level to fall.
Using the equation for pressure in a fluid, we can write:
Pressure before = Pressure after
Atmospheric pressure = Pressure after
Since the height of the water column is directly proportional to the pressure at the bottom of the vessel, we can write:
Initial height / Final height = Atmospheric pressure / Pressure after
Substituting the given values, we have:
H / 200 = 1 / Pressure after
Solving for H, we find:
H = 200 / Pressure after
To determine the fall in height, we need to find the value of Pressure after. This can be calculated using the equation for pressure in a fluid:
Pressure after = Pressure before - pressure due to the height difference
Since the height difference is 300 mm (500 mm - 200 mm), we can write:
Pressure after = Atmospheric pressure - pressure due to the height difference
Substituting the given values, we have:
Pressure after = 1.0 - (density of water * acceleration due to gravity * height difference)
Since the density of water is approximately 1000 kg/m^3 and the acceleration due to gravity is approximately 9.8 m/s^2, we can convert the height difference to meters:
Height difference = 300 mm = 0.3 m
Substituting the values, we have:
Pressure after = 1.0 - (1000 kg/m^3 * 9.8 m/s^2 * 0.3 m)
Pressure after = 1.0 - 294 N/m^2
Pressure after = 706 N/m^2
Finally, substituting this value for Pressure after in the equation for H, we find:
H = 200 / 706
H ≈ 0.283 m
To convert this to mm, we multiply by 1000:
H ≈ 283 mm
Therefore, the fall in height of the water level due to opening the orifice is approximately 283 mm.
Attention Class 11 Students!
To make sure you are not studying endlessly, EduRev has designed Class 11 study material, with Structured Courses, Videos, & Test Series. Plus get personalized analysis, doubt solving and improvement plans to achieve a great score in Class 11.

|
Explore Courses for Class 11 exam
|

|
Similar Class 11 Doubts
A cylindrical vessel of height 500 mm has an orifice (small hole) at its bottom. The orifice is initially closed and water is filled in it up to height H. Now the top is completely sealed with a cap and the orifice at the bottom is opened. Some water comes out from the orifice and the water level in the vessel becomes steady with height of water column being 200 mm. Find the fall in height (in mm) of water level due to opening of the orifice. [Take atmospheric pressure = 1.0 × 10 5 Nm-2, density of water = 1000 kg m-3andg= 10 ms-2. Neglect any effect of surface tension.] (Take temperature to be constant)[JEE 2009]Correct answer is '6'. Can you explain this answer?
Question Description
A cylindrical vessel of height 500 mm has an orifice (small hole) at its bottom. The orifice is initially closed and water is filled in it up to height H. Now the top is completely sealed with a cap and the orifice at the bottom is opened. Some water comes out from the orifice and the water level in the vessel becomes steady with height of water column being 200 mm. Find the fall in height (in mm) of water level due to opening of the orifice. [Take atmospheric pressure = 1.0 × 10 5 Nm-2, density of water = 1000 kg m-3andg= 10 ms-2. Neglect any effect of surface tension.] (Take temperature to be constant)[JEE 2009]Correct answer is '6'. Can you explain this answer? for Class 11 2024 is part of Class 11 preparation. The Question and answers have been prepared according to the Class 11 exam syllabus. Information about A cylindrical vessel of height 500 mm has an orifice (small hole) at its bottom. The orifice is initially closed and water is filled in it up to height H. Now the top is completely sealed with a cap and the orifice at the bottom is opened. Some water comes out from the orifice and the water level in the vessel becomes steady with height of water column being 200 mm. Find the fall in height (in mm) of water level due to opening of the orifice. [Take atmospheric pressure = 1.0 × 10 5 Nm-2, density of water = 1000 kg m-3andg= 10 ms-2. Neglect any effect of surface tension.] (Take temperature to be constant)[JEE 2009]Correct answer is '6'. Can you explain this answer? covers all topics & solutions for Class 11 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A cylindrical vessel of height 500 mm has an orifice (small hole) at its bottom. The orifice is initially closed and water is filled in it up to height H. Now the top is completely sealed with a cap and the orifice at the bottom is opened. Some water comes out from the orifice and the water level in the vessel becomes steady with height of water column being 200 mm. Find the fall in height (in mm) of water level due to opening of the orifice. [Take atmospheric pressure = 1.0 × 10 5 Nm-2, density of water = 1000 kg m-3andg= 10 ms-2. Neglect any effect of surface tension.] (Take temperature to be constant)[JEE 2009]Correct answer is '6'. Can you explain this answer?.
A cylindrical vessel of height 500 mm has an orifice (small hole) at its bottom. The orifice is initially closed and water is filled in it up to height H. Now the top is completely sealed with a cap and the orifice at the bottom is opened. Some water comes out from the orifice and the water level in the vessel becomes steady with height of water column being 200 mm. Find the fall in height (in mm) of water level due to opening of the orifice. [Take atmospheric pressure = 1.0 × 10 5 Nm-2, density of water = 1000 kg m-3andg= 10 ms-2. Neglect any effect of surface tension.] (Take temperature to be constant)[JEE 2009]Correct answer is '6'. Can you explain this answer? for Class 11 2024 is part of Class 11 preparation. The Question and answers have been prepared according to the Class 11 exam syllabus. Information about A cylindrical vessel of height 500 mm has an orifice (small hole) at its bottom. The orifice is initially closed and water is filled in it up to height H. Now the top is completely sealed with a cap and the orifice at the bottom is opened. Some water comes out from the orifice and the water level in the vessel becomes steady with height of water column being 200 mm. Find the fall in height (in mm) of water level due to opening of the orifice. [Take atmospheric pressure = 1.0 × 10 5 Nm-2, density of water = 1000 kg m-3andg= 10 ms-2. Neglect any effect of surface tension.] (Take temperature to be constant)[JEE 2009]Correct answer is '6'. Can you explain this answer? covers all topics & solutions for Class 11 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A cylindrical vessel of height 500 mm has an orifice (small hole) at its bottom. The orifice is initially closed and water is filled in it up to height H. Now the top is completely sealed with a cap and the orifice at the bottom is opened. Some water comes out from the orifice and the water level in the vessel becomes steady with height of water column being 200 mm. Find the fall in height (in mm) of water level due to opening of the orifice. [Take atmospheric pressure = 1.0 × 10 5 Nm-2, density of water = 1000 kg m-3andg= 10 ms-2. Neglect any effect of surface tension.] (Take temperature to be constant)[JEE 2009]Correct answer is '6'. Can you explain this answer?.
Solutions for A cylindrical vessel of height 500 mm has an orifice (small hole) at its bottom. The orifice is initially closed and water is filled in it up to height H. Now the top is completely sealed with a cap and the orifice at the bottom is opened. Some water comes out from the orifice and the water level in the vessel becomes steady with height of water column being 200 mm. Find the fall in height (in mm) of water level due to opening of the orifice. [Take atmospheric pressure = 1.0 × 10 5 Nm-2, density of water = 1000 kg m-3andg= 10 ms-2. Neglect any effect of surface tension.] (Take temperature to be constant)[JEE 2009]Correct answer is '6'. Can you explain this answer? in English & in Hindi are available as part of our courses for Class 11.
Download more important topics, notes, lectures and mock test series for Class 11 Exam by signing up for free.
Here you can find the meaning of A cylindrical vessel of height 500 mm has an orifice (small hole) at its bottom. The orifice is initially closed and water is filled in it up to height H. Now the top is completely sealed with a cap and the orifice at the bottom is opened. Some water comes out from the orifice and the water level in the vessel becomes steady with height of water column being 200 mm. Find the fall in height (in mm) of water level due to opening of the orifice. [Take atmospheric pressure = 1.0 × 10 5 Nm-2, density of water = 1000 kg m-3andg= 10 ms-2. Neglect any effect of surface tension.] (Take temperature to be constant)[JEE 2009]Correct answer is '6'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
A cylindrical vessel of height 500 mm has an orifice (small hole) at its bottom. The orifice is initially closed and water is filled in it up to height H. Now the top is completely sealed with a cap and the orifice at the bottom is opened. Some water comes out from the orifice and the water level in the vessel becomes steady with height of water column being 200 mm. Find the fall in height (in mm) of water level due to opening of the orifice. [Take atmospheric pressure = 1.0 × 10 5 Nm-2, density of water = 1000 kg m-3andg= 10 ms-2. Neglect any effect of surface tension.] (Take temperature to be constant)[JEE 2009]Correct answer is '6'. Can you explain this answer?, a detailed solution for A cylindrical vessel of height 500 mm has an orifice (small hole) at its bottom. The orifice is initially closed and water is filled in it up to height H. Now the top is completely sealed with a cap and the orifice at the bottom is opened. Some water comes out from the orifice and the water level in the vessel becomes steady with height of water column being 200 mm. Find the fall in height (in mm) of water level due to opening of the orifice. [Take atmospheric pressure = 1.0 × 10 5 Nm-2, density of water = 1000 kg m-3andg= 10 ms-2. Neglect any effect of surface tension.] (Take temperature to be constant)[JEE 2009]Correct answer is '6'. Can you explain this answer? has been provided alongside types of A cylindrical vessel of height 500 mm has an orifice (small hole) at its bottom. The orifice is initially closed and water is filled in it up to height H. Now the top is completely sealed with a cap and the orifice at the bottom is opened. Some water comes out from the orifice and the water level in the vessel becomes steady with height of water column being 200 mm. Find the fall in height (in mm) of water level due to opening of the orifice. [Take atmospheric pressure = 1.0 × 10 5 Nm-2, density of water = 1000 kg m-3andg= 10 ms-2. Neglect any effect of surface tension.] (Take temperature to be constant)[JEE 2009]Correct answer is '6'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice A cylindrical vessel of height 500 mm has an orifice (small hole) at its bottom. The orifice is initially closed and water is filled in it up to height H. Now the top is completely sealed with a cap and the orifice at the bottom is opened. Some water comes out from the orifice and the water level in the vessel becomes steady with height of water column being 200 mm. Find the fall in height (in mm) of water level due to opening of the orifice. [Take atmospheric pressure = 1.0 × 10 5 Nm-2, density of water = 1000 kg m-3andg= 10 ms-2. Neglect any effect of surface tension.] (Take temperature to be constant)[JEE 2009]Correct answer is '6'. Can you explain this answer? tests, examples and also practice Class 11 tests.

|
Explore Courses for Class 11 exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
























