Class 11 Exam > Class 11 Questions > Derivation of schrodinger wave equation?
Start Learning for Free
Derivation of schrodinger wave equation?
Most Upvoted Answer
Derivation of schrodinger wave equation?
Schrodinger wave equation is a fundamental equation in quantum mechanics that describes the behavior of particles in a quantum system. It was first introduced by Erwin Schrodinger in 1925. The equation is based on the wave-particle duality of matter, which states that particles can exhibit both wave-like and particle-like behavior. The Schrodinger wave equation is used to calculate the wave function of a particle in a given quantum state.
Derivation of Schrodinger wave equation
The Schrodinger wave equation is derived from the classical wave equation. The classical wave equation describes the behavior of waves in a medium, such as water waves or sound waves. The wave equation relates the second derivative of the wave function with respect to time and space.
The classical wave equation is given by:
∂²u/∂t² = c²∂²u/∂x²
where u is the wave function, t is time, x is position, and c is the speed of the wave.
However, the classical wave equation cannot be applied to describe the behavior of particles in a quantum system. This is because particles in a quantum system exhibit wave-particle duality, which means that they can exhibit both wave-like and particle-like behavior.
To derive the Schrodinger wave equation, Schrodinger made use of de Broglie's hypothesis, which states that particles can also be described by a wave function. Based on this hypothesis, Schrodinger proposed that the wave function of a particle in a quantum system can be described by a complex function Ψ(x,t).
The Schrodinger wave equation can be derived by replacing the classical wave equation with the wave function Ψ(x,t) and making use of the de Broglie relation between the momentum and wavelength of a particle.
The Schrodinger wave equation is given by:
iℏ∂Ψ/∂t = HΨ
where i is the imaginary unit, ℏ is the reduced Planck constant, H is the Hamiltonian operator, and Ψ is the wave function of the particle.
The Hamiltonian operator represents the total energy of the particle and is given by:
H = -ℏ²/2m ∂²/∂x² + V(x)
where m is the mass of the particle and V(x) is the potential energy function.
Conclusion:
In conclusion, the Schrodinger wave equation is a fundamental equation in quantum mechanics that describes the behavior of particles in a quantum system. It is derived from the classical wave equation by replacing the wave function with the particle's wave function and making use of the de Broglie relation between the momentum and wavelength of the particle. The Schrodinger wave equation is a complex equation that is used to calculate the wave function of a particle in a given quantum state.
Derivation of Schrodinger wave equation
The Schrodinger wave equation is derived from the classical wave equation. The classical wave equation describes the behavior of waves in a medium, such as water waves or sound waves. The wave equation relates the second derivative of the wave function with respect to time and space.
The classical wave equation is given by:
∂²u/∂t² = c²∂²u/∂x²
where u is the wave function, t is time, x is position, and c is the speed of the wave.
However, the classical wave equation cannot be applied to describe the behavior of particles in a quantum system. This is because particles in a quantum system exhibit wave-particle duality, which means that they can exhibit both wave-like and particle-like behavior.
To derive the Schrodinger wave equation, Schrodinger made use of de Broglie's hypothesis, which states that particles can also be described by a wave function. Based on this hypothesis, Schrodinger proposed that the wave function of a particle in a quantum system can be described by a complex function Ψ(x,t).
The Schrodinger wave equation can be derived by replacing the classical wave equation with the wave function Ψ(x,t) and making use of the de Broglie relation between the momentum and wavelength of a particle.
The Schrodinger wave equation is given by:
iℏ∂Ψ/∂t = HΨ
where i is the imaginary unit, ℏ is the reduced Planck constant, H is the Hamiltonian operator, and Ψ is the wave function of the particle.
The Hamiltonian operator represents the total energy of the particle and is given by:
H = -ℏ²/2m ∂²/∂x² + V(x)
where m is the mass of the particle and V(x) is the potential energy function.
Conclusion:
In conclusion, the Schrodinger wave equation is a fundamental equation in quantum mechanics that describes the behavior of particles in a quantum system. It is derived from the classical wave equation by replacing the wave function with the particle's wave function and making use of the de Broglie relation between the momentum and wavelength of the particle. The Schrodinger wave equation is a complex equation that is used to calculate the wave function of a particle in a given quantum state.
Community Answer
Derivation of schrodinger wave equation?
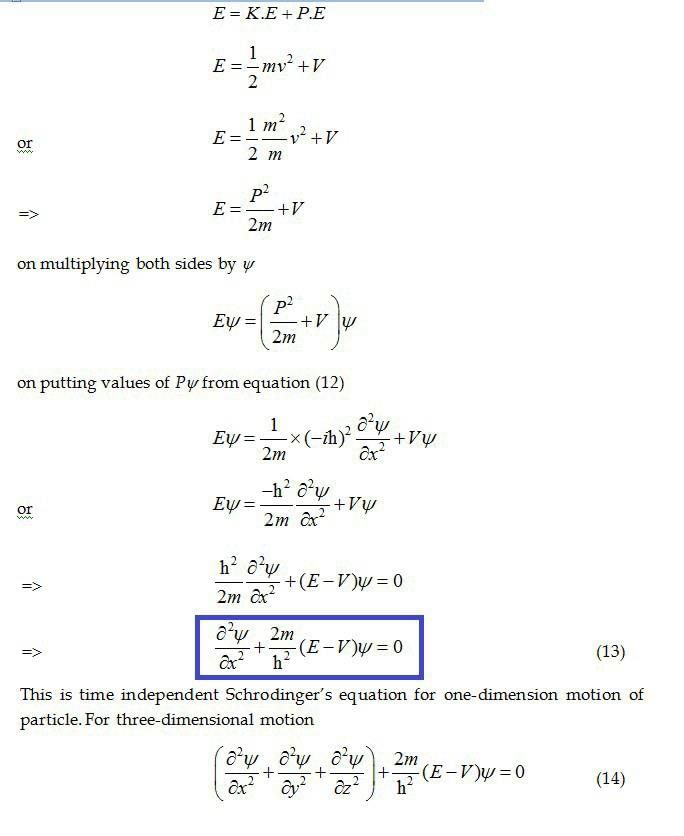

|
Explore Courses for Class 11 exam
|

|
Question Description
Derivation of schrodinger wave equation? for Class 11 2025 is part of Class 11 preparation. The Question and answers have been prepared according to the Class 11 exam syllabus. Information about Derivation of schrodinger wave equation? covers all topics & solutions for Class 11 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Derivation of schrodinger wave equation?.
Derivation of schrodinger wave equation? for Class 11 2025 is part of Class 11 preparation. The Question and answers have been prepared according to the Class 11 exam syllabus. Information about Derivation of schrodinger wave equation? covers all topics & solutions for Class 11 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Derivation of schrodinger wave equation?.
Solutions for Derivation of schrodinger wave equation? in English & in Hindi are available as part of our courses for Class 11.
Download more important topics, notes, lectures and mock test series for Class 11 Exam by signing up for free.
Here you can find the meaning of Derivation of schrodinger wave equation? defined & explained in the simplest way possible. Besides giving the explanation of
Derivation of schrodinger wave equation?, a detailed solution for Derivation of schrodinger wave equation? has been provided alongside types of Derivation of schrodinger wave equation? theory, EduRev gives you an
ample number of questions to practice Derivation of schrodinger wave equation? tests, examples and also practice Class 11 tests.

|
Explore Courses for Class 11 exam
|

|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.























