Class 9 Exam > Class 9 Questions > Two Parallelogram ABCD and EFCD on the same b...
Start Learning for Free
Two Parallelogram ABCD and EFCD on the same base dc and between the same parallels AF and DC are given
?Most Upvoted Answer
Two Parallelogram ABCD and EFCD on the same base dc and between the sa...
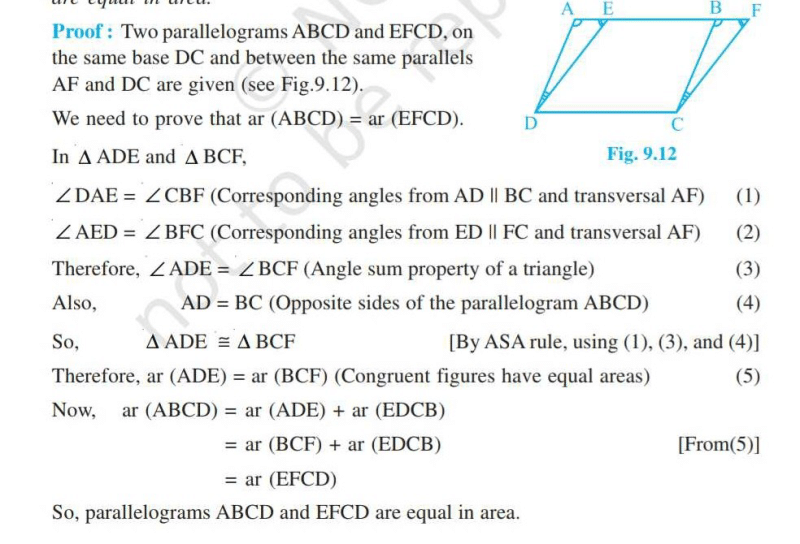
Community Answer
Two Parallelogram ABCD and EFCD on the same base dc and between the sa...
Understanding Areas of Parallelograms
The areas of parallelograms are determined by the base and height. For parallelograms ABCD and EFCD, which share the same base DC and are situated between the same parallels AF and DC, we can analyze their areas effectively.
Key Characteristics
- Equal Bases: Both parallelograms have the same base, DC. This means that the length of base DC is identical for both shapes.
- Same Height: The height is the perpendicular distance from the base to the opposite side. Since both parallelograms are between the same parallels (AF and DC), they share the same height.
Area Calculation
- Area Formula: The area of a parallelogram is calculated using the formula: Area = Base × Height.
- Area of ABCD: Using base DC and the height from point A to line DC, we can express the area as Area(ABCD) = DC × Height.
- Area of EFCD: Similarly, for parallelogram EFCD, Area(EFCD) = DC × Height.
Conclusion
Since both parallelograms share the same base and height, their areas are equal:
- Area(ABCD) = Area(EFCD)
This relationship is crucial in understanding the properties of parallelograms, especially in geometric proofs and applications.
Application in Geometry
- Visual Representation: Drawing the parallelograms helps in visualizing the shared base and height, reinforcing the concept of equal areas.
- Further Exploration: Investigate how this principle applies to other shapes, such as triangles and trapezoids, to deepen understanding of geometric relationships.
This foundational knowledge extends to various geometric problems and proofs encountered in higher mathematics.
The areas of parallelograms are determined by the base and height. For parallelograms ABCD and EFCD, which share the same base DC and are situated between the same parallels AF and DC, we can analyze their areas effectively.
Key Characteristics
- Equal Bases: Both parallelograms have the same base, DC. This means that the length of base DC is identical for both shapes.
- Same Height: The height is the perpendicular distance from the base to the opposite side. Since both parallelograms are between the same parallels (AF and DC), they share the same height.
Area Calculation
- Area Formula: The area of a parallelogram is calculated using the formula: Area = Base × Height.
- Area of ABCD: Using base DC and the height from point A to line DC, we can express the area as Area(ABCD) = DC × Height.
- Area of EFCD: Similarly, for parallelogram EFCD, Area(EFCD) = DC × Height.
Conclusion
Since both parallelograms share the same base and height, their areas are equal:
- Area(ABCD) = Area(EFCD)
This relationship is crucial in understanding the properties of parallelograms, especially in geometric proofs and applications.
Application in Geometry
- Visual Representation: Drawing the parallelograms helps in visualizing the shared base and height, reinforcing the concept of equal areas.
- Further Exploration: Investigate how this principle applies to other shapes, such as triangles and trapezoids, to deepen understanding of geometric relationships.
This foundational knowledge extends to various geometric problems and proofs encountered in higher mathematics.
Attention Class 9 Students!
To make sure you are not studying endlessly, EduRev has designed Class 9 study material, with Structured Courses, Videos, & Test Series. Plus get personalized analysis, doubt solving and improvement plans to achieve a great score in Class 9.

|
Explore Courses for Class 9 exam
|

|
Similar Class 9 Doubts
Two Parallelogram ABCD and EFCD on the same base dc and between the same parallels AF and DC are given Related: Facts that Matter- Areas of Parallelograms and Triangles?
Question Description
Two Parallelogram ABCD and EFCD on the same base dc and between the same parallels AF and DC are given Related: Facts that Matter- Areas of Parallelograms and Triangles? for Class 9 2024 is part of Class 9 preparation. The Question and answers have been prepared according to the Class 9 exam syllabus. Information about Two Parallelogram ABCD and EFCD on the same base dc and between the same parallels AF and DC are given Related: Facts that Matter- Areas of Parallelograms and Triangles? covers all topics & solutions for Class 9 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Two Parallelogram ABCD and EFCD on the same base dc and between the same parallels AF and DC are given Related: Facts that Matter- Areas of Parallelograms and Triangles?.
Two Parallelogram ABCD and EFCD on the same base dc and between the same parallels AF and DC are given Related: Facts that Matter- Areas of Parallelograms and Triangles? for Class 9 2024 is part of Class 9 preparation. The Question and answers have been prepared according to the Class 9 exam syllabus. Information about Two Parallelogram ABCD and EFCD on the same base dc and between the same parallels AF and DC are given Related: Facts that Matter- Areas of Parallelograms and Triangles? covers all topics & solutions for Class 9 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Two Parallelogram ABCD and EFCD on the same base dc and between the same parallels AF and DC are given Related: Facts that Matter- Areas of Parallelograms and Triangles?.
Solutions for Two Parallelogram ABCD and EFCD on the same base dc and between the same parallels AF and DC are given Related: Facts that Matter- Areas of Parallelograms and Triangles? in English & in Hindi are available as part of our courses for Class 9.
Download more important topics, notes, lectures and mock test series for Class 9 Exam by signing up for free.
Here you can find the meaning of Two Parallelogram ABCD and EFCD on the same base dc and between the same parallels AF and DC are given Related: Facts that Matter- Areas of Parallelograms and Triangles? defined & explained in the simplest way possible. Besides giving the explanation of
Two Parallelogram ABCD and EFCD on the same base dc and between the same parallels AF and DC are given Related: Facts that Matter- Areas of Parallelograms and Triangles?, a detailed solution for Two Parallelogram ABCD and EFCD on the same base dc and between the same parallels AF and DC are given Related: Facts that Matter- Areas of Parallelograms and Triangles? has been provided alongside types of Two Parallelogram ABCD and EFCD on the same base dc and between the same parallels AF and DC are given Related: Facts that Matter- Areas of Parallelograms and Triangles? theory, EduRev gives you an
ample number of questions to practice Two Parallelogram ABCD and EFCD on the same base dc and between the same parallels AF and DC are given Related: Facts that Matter- Areas of Parallelograms and Triangles? tests, examples and also practice Class 9 tests.

|
Explore Courses for Class 9 exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.

























