Mechanical Engineering Exam > Mechanical Engineering Questions > A thin cylindrical tube closed at ends is sub...
Start Learning for Free
A thin cylindrical tube closed at ends is subjected to internal pressure. A torque is also applied to the tube. The principal stresses p1 and p2 developed are 80 unit and 20 unit respectively. If the yield stress is 240 units then what is the factor of safety according to maximum shear stress theory
- a)3
- b)4
- c)5
- d)2
Correct answer is option 'B'. Can you explain this answer?
Verified Answer
A thin cylindrical tube closed at ends is subjected to internal pressu...
Maximum shear stress due to applied loading
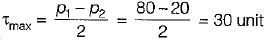
Maximum shear stress in material at yield stress under uni-axial tension
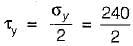
= 120 unit
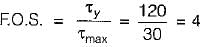
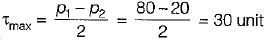
Maximum shear stress in material at yield stress under uni-axial tension
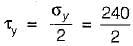
= 120 unit
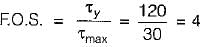
Most Upvoted Answer
A thin cylindrical tube closed at ends is subjected to internal pressu...
Given data:
Principal stress p1 = 80 units
Principal stress p2 = 20 units
Yield stress = 240 units
Explanation:
The maximum shear stress theory states that failure will occur when the maximum shear stress in a material exceeds its shear strength.
The maximum shear stress can be determined using the formula:
σ_max = (p1 - p2) / 2
where σ_max is the maximum shear stress and p1 and p2 are the principal stresses.
Substituting the given values, we have:
σ_max = (80 - 20) / 2
= 30 units
Now, the factor of safety (FOS) is given by:
FOS = Yield stress / Maximum shear stress
Substituting the given values, we have:
FOS = 240 / 30
= 8
Therefore, the factor of safety according to the maximum shear stress theory is 8.
However, the options provided are not in the form of a ratio. To convert the factor of safety to a ratio, we need to subtract 1 from the factor of safety and divide by 1. This gives us:
Ratio = (FOS - 1) / 1
Substituting the value of FOS, we have:
Ratio = (8 - 1) / 1
= 7 / 1
= 7
Therefore, the factor of safety according to the maximum shear stress theory is 7:1.
Conclusion:
The correct answer is option 'B', which corresponds to a factor of safety of 4.
Principal stress p1 = 80 units
Principal stress p2 = 20 units
Yield stress = 240 units
Explanation:
The maximum shear stress theory states that failure will occur when the maximum shear stress in a material exceeds its shear strength.
The maximum shear stress can be determined using the formula:
σ_max = (p1 - p2) / 2
where σ_max is the maximum shear stress and p1 and p2 are the principal stresses.
Substituting the given values, we have:
σ_max = (80 - 20) / 2
= 30 units
Now, the factor of safety (FOS) is given by:
FOS = Yield stress / Maximum shear stress
Substituting the given values, we have:
FOS = 240 / 30
= 8
Therefore, the factor of safety according to the maximum shear stress theory is 8.
However, the options provided are not in the form of a ratio. To convert the factor of safety to a ratio, we need to subtract 1 from the factor of safety and divide by 1. This gives us:
Ratio = (FOS - 1) / 1
Substituting the value of FOS, we have:
Ratio = (8 - 1) / 1
= 7 / 1
= 7
Therefore, the factor of safety according to the maximum shear stress theory is 7:1.
Conclusion:
The correct answer is option 'B', which corresponds to a factor of safety of 4.

|
Explore Courses for Mechanical Engineering exam
|

|
Similar Mechanical Engineering Doubts
A thin cylindrical tube closed at ends is subjected to internal pressure. A torque is also applied to the tube. The principal stresses p1 and p2 developed are 80 unit and 20 unit respectively. If the yield stress is 240 units then what is the factor of safety according to maximum shear stress theorya)3b)4c)5d)2Correct answer is option 'B'. Can you explain this answer?
Question Description
A thin cylindrical tube closed at ends is subjected to internal pressure. A torque is also applied to the tube. The principal stresses p1 and p2 developed are 80 unit and 20 unit respectively. If the yield stress is 240 units then what is the factor of safety according to maximum shear stress theorya)3b)4c)5d)2Correct answer is option 'B'. Can you explain this answer? for Mechanical Engineering 2025 is part of Mechanical Engineering preparation. The Question and answers have been prepared according to the Mechanical Engineering exam syllabus. Information about A thin cylindrical tube closed at ends is subjected to internal pressure. A torque is also applied to the tube. The principal stresses p1 and p2 developed are 80 unit and 20 unit respectively. If the yield stress is 240 units then what is the factor of safety according to maximum shear stress theorya)3b)4c)5d)2Correct answer is option 'B'. Can you explain this answer? covers all topics & solutions for Mechanical Engineering 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A thin cylindrical tube closed at ends is subjected to internal pressure. A torque is also applied to the tube. The principal stresses p1 and p2 developed are 80 unit and 20 unit respectively. If the yield stress is 240 units then what is the factor of safety according to maximum shear stress theorya)3b)4c)5d)2Correct answer is option 'B'. Can you explain this answer?.
A thin cylindrical tube closed at ends is subjected to internal pressure. A torque is also applied to the tube. The principal stresses p1 and p2 developed are 80 unit and 20 unit respectively. If the yield stress is 240 units then what is the factor of safety according to maximum shear stress theorya)3b)4c)5d)2Correct answer is option 'B'. Can you explain this answer? for Mechanical Engineering 2025 is part of Mechanical Engineering preparation. The Question and answers have been prepared according to the Mechanical Engineering exam syllabus. Information about A thin cylindrical tube closed at ends is subjected to internal pressure. A torque is also applied to the tube. The principal stresses p1 and p2 developed are 80 unit and 20 unit respectively. If the yield stress is 240 units then what is the factor of safety according to maximum shear stress theorya)3b)4c)5d)2Correct answer is option 'B'. Can you explain this answer? covers all topics & solutions for Mechanical Engineering 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A thin cylindrical tube closed at ends is subjected to internal pressure. A torque is also applied to the tube. The principal stresses p1 and p2 developed are 80 unit and 20 unit respectively. If the yield stress is 240 units then what is the factor of safety according to maximum shear stress theorya)3b)4c)5d)2Correct answer is option 'B'. Can you explain this answer?.
Solutions for A thin cylindrical tube closed at ends is subjected to internal pressure. A torque is also applied to the tube. The principal stresses p1 and p2 developed are 80 unit and 20 unit respectively. If the yield stress is 240 units then what is the factor of safety according to maximum shear stress theorya)3b)4c)5d)2Correct answer is option 'B'. Can you explain this answer? in English & in Hindi are available as part of our courses for Mechanical Engineering.
Download more important topics, notes, lectures and mock test series for Mechanical Engineering Exam by signing up for free.
Here you can find the meaning of A thin cylindrical tube closed at ends is subjected to internal pressure. A torque is also applied to the tube. The principal stresses p1 and p2 developed are 80 unit and 20 unit respectively. If the yield stress is 240 units then what is the factor of safety according to maximum shear stress theorya)3b)4c)5d)2Correct answer is option 'B'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
A thin cylindrical tube closed at ends is subjected to internal pressure. A torque is also applied to the tube. The principal stresses p1 and p2 developed are 80 unit and 20 unit respectively. If the yield stress is 240 units then what is the factor of safety according to maximum shear stress theorya)3b)4c)5d)2Correct answer is option 'B'. Can you explain this answer?, a detailed solution for A thin cylindrical tube closed at ends is subjected to internal pressure. A torque is also applied to the tube. The principal stresses p1 and p2 developed are 80 unit and 20 unit respectively. If the yield stress is 240 units then what is the factor of safety according to maximum shear stress theorya)3b)4c)5d)2Correct answer is option 'B'. Can you explain this answer? has been provided alongside types of A thin cylindrical tube closed at ends is subjected to internal pressure. A torque is also applied to the tube. The principal stresses p1 and p2 developed are 80 unit and 20 unit respectively. If the yield stress is 240 units then what is the factor of safety according to maximum shear stress theorya)3b)4c)5d)2Correct answer is option 'B'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice A thin cylindrical tube closed at ends is subjected to internal pressure. A torque is also applied to the tube. The principal stresses p1 and p2 developed are 80 unit and 20 unit respectively. If the yield stress is 240 units then what is the factor of safety according to maximum shear stress theorya)3b)4c)5d)2Correct answer is option 'B'. Can you explain this answer? tests, examples and also practice Mechanical Engineering tests.

|
Explore Courses for Mechanical Engineering exam
|

|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
























