Mechanical Engineering Exam > Mechanical Engineering Questions > A spherical steel ball of 12 mm diameter is i...
Start Learning for Free
A spherical steel ball of 12 mm diameter is initially at 1000 K. It is slowly cooled in a surrounding of 300 K. The heat transfer coefficient between the steel ball and the surroundings is 5 W/m2K. The thermal conductivity of steel is 20 W/mK. The ternperature difference between the centre and the surface of the steel ball is
[2011]
- a)large because conduction resistance is far higher than the convective resistance.
- b)large because conduction resistance is far less than the convective resistance.
- c)small because conduction resistance is far higher than the convective resistance.
- d)small because conduction resistance is far less than the convective resistance.
Correct answer is option 'D'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
A spherical steel ball of 12 mm diameter is initially at 1000 K. It is...
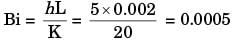
For the given condition, Biot number tends to zero, i.e. conduction resistance is far less than convection resistance. Therefore temperature between centre and surface is very small.
Most Upvoted Answer
A spherical steel ball of 12 mm diameter is initially at 1000 K. It is...
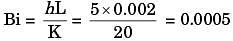
For the given condition, Biot number tends to zero, i.e. conduction resistance is far less than convection resistance. Therefore temperature between centre and surface is very small.
Free Test
| FREE | Start Free Test |
Community Answer
A spherical steel ball of 12 mm diameter is initially at 1000 K. It is...
Explanation:
1. Background:
In this problem, we have a spherical steel ball that is initially at a temperature of 1000 K. It is slowly cooled in a surrounding environment at a temperature of 300 K. We are given the heat transfer coefficient between the steel ball and the surroundings (h = 5 W/m2K) and the thermal conductivity of steel (k = 20 W/mK). We need to determine the temperature difference between the center and the surface of the steel ball.
2. Heat Transfer Modes:
Heat transfer can occur through conduction, convection, and radiation. In this problem, the dominant modes of heat transfer are conduction and convection.
3. Conduction Resistance:
Conduction is the transfer of heat through a solid material. The temperature difference across a material is the driving force for conduction. In this problem, the temperature difference between the center and the surface of the steel ball will create a temperature gradient, which drives the heat transfer through conduction.
4. Convective Resistance:
Convection is the transfer of heat between a solid surface and a fluid (liquid or gas) in motion. The heat transfer coefficient (h) represents the resistance of the fluid to the transfer of heat. A higher heat transfer coefficient indicates better convective heat transfer.
5. Comparison of Conduction and Convection Resistances:
To determine the temperature difference between the center and the surface of the steel ball, we need to compare the conduction and convection resistances.
- The conduction resistance is determined by the thermal conductivity of the material (k) and the distance over which heat is transferred. In this case, the distance would be the radius of the steel ball (r = 6 mm).
- The convective resistance is determined by the heat transfer coefficient (h) and the surface area over which heat is transferred. In this case, the surface area would be the surface area of the steel ball (A = 4πr^2).
6. Analysis:
To determine whether the conduction or convective resistance is dominant, we can compare the dimensionless numbers associated with each mode of heat transfer.
- The Biot number (Bi) is a ratio of the conduction resistance to the convective resistance and is given by Bi = hL/k, where L is the characteristic length (radius in this case).
- A high Biot number indicates that the convection resistance dominates, while a low Biot number indicates that the conduction resistance dominates.
In this problem, the Biot number can be calculated as Bi = (5 × 0.006)/(20) = 0.0015.
7. Conclusion:
Since the Biot number is very small (0.0015), it indicates that the conduction resistance is much smaller compared to the convective resistance. Therefore, the temperature difference between the center and the surface of the steel ball will be small. Hence, the correct answer is option D, "small because conduction resistance is far less than the convective resistance."
1. Background:
In this problem, we have a spherical steel ball that is initially at a temperature of 1000 K. It is slowly cooled in a surrounding environment at a temperature of 300 K. We are given the heat transfer coefficient between the steel ball and the surroundings (h = 5 W/m2K) and the thermal conductivity of steel (k = 20 W/mK). We need to determine the temperature difference between the center and the surface of the steel ball.
2. Heat Transfer Modes:
Heat transfer can occur through conduction, convection, and radiation. In this problem, the dominant modes of heat transfer are conduction and convection.
3. Conduction Resistance:
Conduction is the transfer of heat through a solid material. The temperature difference across a material is the driving force for conduction. In this problem, the temperature difference between the center and the surface of the steel ball will create a temperature gradient, which drives the heat transfer through conduction.
4. Convective Resistance:
Convection is the transfer of heat between a solid surface and a fluid (liquid or gas) in motion. The heat transfer coefficient (h) represents the resistance of the fluid to the transfer of heat. A higher heat transfer coefficient indicates better convective heat transfer.
5. Comparison of Conduction and Convection Resistances:
To determine the temperature difference between the center and the surface of the steel ball, we need to compare the conduction and convection resistances.
- The conduction resistance is determined by the thermal conductivity of the material (k) and the distance over which heat is transferred. In this case, the distance would be the radius of the steel ball (r = 6 mm).
- The convective resistance is determined by the heat transfer coefficient (h) and the surface area over which heat is transferred. In this case, the surface area would be the surface area of the steel ball (A = 4πr^2).
6. Analysis:
To determine whether the conduction or convective resistance is dominant, we can compare the dimensionless numbers associated with each mode of heat transfer.
- The Biot number (Bi) is a ratio of the conduction resistance to the convective resistance and is given by Bi = hL/k, where L is the characteristic length (radius in this case).
- A high Biot number indicates that the convection resistance dominates, while a low Biot number indicates that the conduction resistance dominates.
In this problem, the Biot number can be calculated as Bi = (5 × 0.006)/(20) = 0.0015.
7. Conclusion:
Since the Biot number is very small (0.0015), it indicates that the conduction resistance is much smaller compared to the convective resistance. Therefore, the temperature difference between the center and the surface of the steel ball will be small. Hence, the correct answer is option D, "small because conduction resistance is far less than the convective resistance."
Attention Mechanical Engineering Students!
To make sure you are not studying endlessly, EduRev has designed Mechanical Engineering study material, with Structured Courses, Videos, & Test Series. Plus get personalized analysis, doubt solving and improvement plans to achieve a great score in Mechanical Engineering.

|
Explore Courses for Mechanical Engineering exam
|

|
Similar Mechanical Engineering Doubts
A spherical steel ball of 12 mm diameter is initially at 1000 K. It is slowly cooled in a surrounding of 300 K. The heat transfer coefficient between the steel ball and the surroundings is 5 W/m2K. The thermal conductivity of steel is 20 W/mK. The ternperature difference between the centre and the surface of the steel ball is[2011]a)large because conduction resistance is far higher than the convective resistance.b)large because conduction resistance is far less than the convective resistance.c)small because conduction resistance is far higher than the convective resistance.d)small because conduction resistance is far less than the convective resistance.Correct answer is option 'D'. Can you explain this answer?
Question Description
A spherical steel ball of 12 mm diameter is initially at 1000 K. It is slowly cooled in a surrounding of 300 K. The heat transfer coefficient between the steel ball and the surroundings is 5 W/m2K. The thermal conductivity of steel is 20 W/mK. The ternperature difference between the centre and the surface of the steel ball is[2011]a)large because conduction resistance is far higher than the convective resistance.b)large because conduction resistance is far less than the convective resistance.c)small because conduction resistance is far higher than the convective resistance.d)small because conduction resistance is far less than the convective resistance.Correct answer is option 'D'. Can you explain this answer? for Mechanical Engineering 2024 is part of Mechanical Engineering preparation. The Question and answers have been prepared according to the Mechanical Engineering exam syllabus. Information about A spherical steel ball of 12 mm diameter is initially at 1000 K. It is slowly cooled in a surrounding of 300 K. The heat transfer coefficient between the steel ball and the surroundings is 5 W/m2K. The thermal conductivity of steel is 20 W/mK. The ternperature difference between the centre and the surface of the steel ball is[2011]a)large because conduction resistance is far higher than the convective resistance.b)large because conduction resistance is far less than the convective resistance.c)small because conduction resistance is far higher than the convective resistance.d)small because conduction resistance is far less than the convective resistance.Correct answer is option 'D'. Can you explain this answer? covers all topics & solutions for Mechanical Engineering 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A spherical steel ball of 12 mm diameter is initially at 1000 K. It is slowly cooled in a surrounding of 300 K. The heat transfer coefficient between the steel ball and the surroundings is 5 W/m2K. The thermal conductivity of steel is 20 W/mK. The ternperature difference between the centre and the surface of the steel ball is[2011]a)large because conduction resistance is far higher than the convective resistance.b)large because conduction resistance is far less than the convective resistance.c)small because conduction resistance is far higher than the convective resistance.d)small because conduction resistance is far less than the convective resistance.Correct answer is option 'D'. Can you explain this answer?.
A spherical steel ball of 12 mm diameter is initially at 1000 K. It is slowly cooled in a surrounding of 300 K. The heat transfer coefficient between the steel ball and the surroundings is 5 W/m2K. The thermal conductivity of steel is 20 W/mK. The ternperature difference between the centre and the surface of the steel ball is[2011]a)large because conduction resistance is far higher than the convective resistance.b)large because conduction resistance is far less than the convective resistance.c)small because conduction resistance is far higher than the convective resistance.d)small because conduction resistance is far less than the convective resistance.Correct answer is option 'D'. Can you explain this answer? for Mechanical Engineering 2024 is part of Mechanical Engineering preparation. The Question and answers have been prepared according to the Mechanical Engineering exam syllabus. Information about A spherical steel ball of 12 mm diameter is initially at 1000 K. It is slowly cooled in a surrounding of 300 K. The heat transfer coefficient between the steel ball and the surroundings is 5 W/m2K. The thermal conductivity of steel is 20 W/mK. The ternperature difference between the centre and the surface of the steel ball is[2011]a)large because conduction resistance is far higher than the convective resistance.b)large because conduction resistance is far less than the convective resistance.c)small because conduction resistance is far higher than the convective resistance.d)small because conduction resistance is far less than the convective resistance.Correct answer is option 'D'. Can you explain this answer? covers all topics & solutions for Mechanical Engineering 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A spherical steel ball of 12 mm diameter is initially at 1000 K. It is slowly cooled in a surrounding of 300 K. The heat transfer coefficient between the steel ball and the surroundings is 5 W/m2K. The thermal conductivity of steel is 20 W/mK. The ternperature difference between the centre and the surface of the steel ball is[2011]a)large because conduction resistance is far higher than the convective resistance.b)large because conduction resistance is far less than the convective resistance.c)small because conduction resistance is far higher than the convective resistance.d)small because conduction resistance is far less than the convective resistance.Correct answer is option 'D'. Can you explain this answer?.
Solutions for A spherical steel ball of 12 mm diameter is initially at 1000 K. It is slowly cooled in a surrounding of 300 K. The heat transfer coefficient between the steel ball and the surroundings is 5 W/m2K. The thermal conductivity of steel is 20 W/mK. The ternperature difference between the centre and the surface of the steel ball is[2011]a)large because conduction resistance is far higher than the convective resistance.b)large because conduction resistance is far less than the convective resistance.c)small because conduction resistance is far higher than the convective resistance.d)small because conduction resistance is far less than the convective resistance.Correct answer is option 'D'. Can you explain this answer? in English & in Hindi are available as part of our courses for Mechanical Engineering.
Download more important topics, notes, lectures and mock test series for Mechanical Engineering Exam by signing up for free.
Here you can find the meaning of A spherical steel ball of 12 mm diameter is initially at 1000 K. It is slowly cooled in a surrounding of 300 K. The heat transfer coefficient between the steel ball and the surroundings is 5 W/m2K. The thermal conductivity of steel is 20 W/mK. The ternperature difference between the centre and the surface of the steel ball is[2011]a)large because conduction resistance is far higher than the convective resistance.b)large because conduction resistance is far less than the convective resistance.c)small because conduction resistance is far higher than the convective resistance.d)small because conduction resistance is far less than the convective resistance.Correct answer is option 'D'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
A spherical steel ball of 12 mm diameter is initially at 1000 K. It is slowly cooled in a surrounding of 300 K. The heat transfer coefficient between the steel ball and the surroundings is 5 W/m2K. The thermal conductivity of steel is 20 W/mK. The ternperature difference between the centre and the surface of the steel ball is[2011]a)large because conduction resistance is far higher than the convective resistance.b)large because conduction resistance is far less than the convective resistance.c)small because conduction resistance is far higher than the convective resistance.d)small because conduction resistance is far less than the convective resistance.Correct answer is option 'D'. Can you explain this answer?, a detailed solution for A spherical steel ball of 12 mm diameter is initially at 1000 K. It is slowly cooled in a surrounding of 300 K. The heat transfer coefficient between the steel ball and the surroundings is 5 W/m2K. The thermal conductivity of steel is 20 W/mK. The ternperature difference between the centre and the surface of the steel ball is[2011]a)large because conduction resistance is far higher than the convective resistance.b)large because conduction resistance is far less than the convective resistance.c)small because conduction resistance is far higher than the convective resistance.d)small because conduction resistance is far less than the convective resistance.Correct answer is option 'D'. Can you explain this answer? has been provided alongside types of A spherical steel ball of 12 mm diameter is initially at 1000 K. It is slowly cooled in a surrounding of 300 K. The heat transfer coefficient between the steel ball and the surroundings is 5 W/m2K. The thermal conductivity of steel is 20 W/mK. The ternperature difference between the centre and the surface of the steel ball is[2011]a)large because conduction resistance is far higher than the convective resistance.b)large because conduction resistance is far less than the convective resistance.c)small because conduction resistance is far higher than the convective resistance.d)small because conduction resistance is far less than the convective resistance.Correct answer is option 'D'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice A spherical steel ball of 12 mm diameter is initially at 1000 K. It is slowly cooled in a surrounding of 300 K. The heat transfer coefficient between the steel ball and the surroundings is 5 W/m2K. The thermal conductivity of steel is 20 W/mK. The ternperature difference between the centre and the surface of the steel ball is[2011]a)large because conduction resistance is far higher than the convective resistance.b)large because conduction resistance is far less than the convective resistance.c)small because conduction resistance is far higher than the convective resistance.d)small because conduction resistance is far less than the convective resistance.Correct answer is option 'D'. Can you explain this answer? tests, examples and also practice Mechanical Engineering tests.

|
Explore Courses for Mechanical Engineering exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
























