JEE Exam > JEE Questions > A conducting circular loop is placed in a uni...
Start Learning for Free
A conducting circular loop is placed in a uniform magnetic field of 0.04 T with its plane perpendicular to the magnetic field. The radius of the loop starts shrinking at 2 mm/s. The induced emf in the loop when the radius is 2 cm is
- a)4.8 π μV
- b)0.8 π μV
- c)1.6 π μV
- d)3.2 π μV
Correct answer is option 'D'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Most Upvoted Answer
A conducting circular loop is placed in a uniform magnetic field of 0....
Induced emf in th e loop is gi ven by
e = -B. dA/dt where A is the area of the loop.
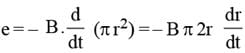
r = 2cm = 2 × 10–2 m
dr = 2 mm = 2 × 10–3 m
dt = 1s
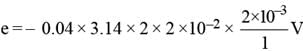
= 0.32 π × 10–5V
= 3.2 π ×10–6V
= 3.2 π μV
e = -B. dA/dt where A is the area of the loop.
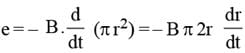
r = 2cm = 2 × 10–2 m
dr = 2 mm = 2 × 10–3 m
dt = 1s
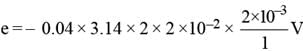
= 0.32 π × 10–5V
= 3.2 π ×10–6V
= 3.2 π μV
Free Test
FREE
| Start Free Test |
Community Answer
A conducting circular loop is placed in a uniform magnetic field of 0....
To find the induced emf in the loop, we can use Faraday's law of electromagnetic induction:
emf = -dφ/dt
where emf is the induced electromotive force, dφ is the change in magnetic flux, and dt is the change in time.
The magnetic flux through the loop is given by:
Φ = B⋅A
where B is the magnetic field strength and A is the area of the loop.
Since the loop is circular and its plane is perpendicular to the magnetic field, the area of the loop remains constant as the radius shrinks.
Therefore, the change in magnetic flux is simply the product of the change in magnetic field and the constant area:
dΦ/dt = B⋅dA/dt
Since dA/dt is the rate at which the area changes with time, and the radius is shrinking at 2 mm/s, we have:
dA/dt = -2πr(dr/dt)
where r is the radius of the loop.
Substituting this expression into the equation for the change in magnetic flux, we get:
dΦ/dt = -2πr(dr/dt)B
Now we can substitute this expression into Faraday's law of electromagnetic induction to find the induced emf:
emf = -dΦ/dt
= -(-2πr(dr/dt)B)
= 2πr(dr/dt)B
Substituting the given values of r = 2 cm = 0.02 m, dr/dt = -2 mm/s = -0.002 m/s, and B = 0.04 T, we can calculate the induced emf:
emf = 2π(0.02)(-0.002)(0.04)
= -0.00008π V
Since the emf is negative, it means that the induced current will flow in the opposite direction to the change in magnetic field.
Approximating π ≈ 3.14, the induced emf is approximately:
emf ≈ -0.00008(3.14) V
≈ -0.0002512 V
Therefore, the induced emf in the loop when the radius is 2 cm is approximately -0.0002512 V, or -251.2 μV.
emf = -dφ/dt
where emf is the induced electromotive force, dφ is the change in magnetic flux, and dt is the change in time.
The magnetic flux through the loop is given by:
Φ = B⋅A
where B is the magnetic field strength and A is the area of the loop.
Since the loop is circular and its plane is perpendicular to the magnetic field, the area of the loop remains constant as the radius shrinks.
Therefore, the change in magnetic flux is simply the product of the change in magnetic field and the constant area:
dΦ/dt = B⋅dA/dt
Since dA/dt is the rate at which the area changes with time, and the radius is shrinking at 2 mm/s, we have:
dA/dt = -2πr(dr/dt)
where r is the radius of the loop.
Substituting this expression into the equation for the change in magnetic flux, we get:
dΦ/dt = -2πr(dr/dt)B
Now we can substitute this expression into Faraday's law of electromagnetic induction to find the induced emf:
emf = -dΦ/dt
= -(-2πr(dr/dt)B)
= 2πr(dr/dt)B
Substituting the given values of r = 2 cm = 0.02 m, dr/dt = -2 mm/s = -0.002 m/s, and B = 0.04 T, we can calculate the induced emf:
emf = 2π(0.02)(-0.002)(0.04)
= -0.00008π V
Since the emf is negative, it means that the induced current will flow in the opposite direction to the change in magnetic field.
Approximating π ≈ 3.14, the induced emf is approximately:
emf ≈ -0.00008(3.14) V
≈ -0.0002512 V
Therefore, the induced emf in the loop when the radius is 2 cm is approximately -0.0002512 V, or -251.2 μV.
Attention JEE Students!
To make sure you are not studying endlessly, EduRev has designed JEE study material, with Structured Courses, Videos, & Test Series. Plus get personalized analysis, doubt solving and improvement plans to achieve a great score in JEE.

|
Explore Courses for JEE exam
|

|
A conducting circular loop is placed in a uniform magnetic field of 0.04 T with its plane perpendicular to the magnetic field. The radius of the loop starts shrinking at 2 mm/s. The induced emf in the loop when the radius is 2 cm isa)4.8 π μVb)0.8 π μVc)1.6 π μVd)3.2 π μVCorrect answer is option 'D'. Can you explain this answer?
Question Description
A conducting circular loop is placed in a uniform magnetic field of 0.04 T with its plane perpendicular to the magnetic field. The radius of the loop starts shrinking at 2 mm/s. The induced emf in the loop when the radius is 2 cm isa)4.8 π μVb)0.8 π μVc)1.6 π μVd)3.2 π μVCorrect answer is option 'D'. Can you explain this answer? for JEE 2024 is part of JEE preparation. The Question and answers have been prepared according to the JEE exam syllabus. Information about A conducting circular loop is placed in a uniform magnetic field of 0.04 T with its plane perpendicular to the magnetic field. The radius of the loop starts shrinking at 2 mm/s. The induced emf in the loop when the radius is 2 cm isa)4.8 π μVb)0.8 π μVc)1.6 π μVd)3.2 π μVCorrect answer is option 'D'. Can you explain this answer? covers all topics & solutions for JEE 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A conducting circular loop is placed in a uniform magnetic field of 0.04 T with its plane perpendicular to the magnetic field. The radius of the loop starts shrinking at 2 mm/s. The induced emf in the loop when the radius is 2 cm isa)4.8 π μVb)0.8 π μVc)1.6 π μVd)3.2 π μVCorrect answer is option 'D'. Can you explain this answer?.
A conducting circular loop is placed in a uniform magnetic field of 0.04 T with its plane perpendicular to the magnetic field. The radius of the loop starts shrinking at 2 mm/s. The induced emf in the loop when the radius is 2 cm isa)4.8 π μVb)0.8 π μVc)1.6 π μVd)3.2 π μVCorrect answer is option 'D'. Can you explain this answer? for JEE 2024 is part of JEE preparation. The Question and answers have been prepared according to the JEE exam syllabus. Information about A conducting circular loop is placed in a uniform magnetic field of 0.04 T with its plane perpendicular to the magnetic field. The radius of the loop starts shrinking at 2 mm/s. The induced emf in the loop when the radius is 2 cm isa)4.8 π μVb)0.8 π μVc)1.6 π μVd)3.2 π μVCorrect answer is option 'D'. Can you explain this answer? covers all topics & solutions for JEE 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A conducting circular loop is placed in a uniform magnetic field of 0.04 T with its plane perpendicular to the magnetic field. The radius of the loop starts shrinking at 2 mm/s. The induced emf in the loop when the radius is 2 cm isa)4.8 π μVb)0.8 π μVc)1.6 π μVd)3.2 π μVCorrect answer is option 'D'. Can you explain this answer?.
Solutions for A conducting circular loop is placed in a uniform magnetic field of 0.04 T with its plane perpendicular to the magnetic field. The radius of the loop starts shrinking at 2 mm/s. The induced emf in the loop when the radius is 2 cm isa)4.8 π μVb)0.8 π μVc)1.6 π μVd)3.2 π μVCorrect answer is option 'D'. Can you explain this answer? in English & in Hindi are available as part of our courses for JEE.
Download more important topics, notes, lectures and mock test series for JEE Exam by signing up for free.
Here you can find the meaning of A conducting circular loop is placed in a uniform magnetic field of 0.04 T with its plane perpendicular to the magnetic field. The radius of the loop starts shrinking at 2 mm/s. The induced emf in the loop when the radius is 2 cm isa)4.8 π μVb)0.8 π μVc)1.6 π μVd)3.2 π μVCorrect answer is option 'D'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
A conducting circular loop is placed in a uniform magnetic field of 0.04 T with its plane perpendicular to the magnetic field. The radius of the loop starts shrinking at 2 mm/s. The induced emf in the loop when the radius is 2 cm isa)4.8 π μVb)0.8 π μVc)1.6 π μVd)3.2 π μVCorrect answer is option 'D'. Can you explain this answer?, a detailed solution for A conducting circular loop is placed in a uniform magnetic field of 0.04 T with its plane perpendicular to the magnetic field. The radius of the loop starts shrinking at 2 mm/s. The induced emf in the loop when the radius is 2 cm isa)4.8 π μVb)0.8 π μVc)1.6 π μVd)3.2 π μVCorrect answer is option 'D'. Can you explain this answer? has been provided alongside types of A conducting circular loop is placed in a uniform magnetic field of 0.04 T with its plane perpendicular to the magnetic field. The radius of the loop starts shrinking at 2 mm/s. The induced emf in the loop when the radius is 2 cm isa)4.8 π μVb)0.8 π μVc)1.6 π μVd)3.2 π μVCorrect answer is option 'D'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice A conducting circular loop is placed in a uniform magnetic field of 0.04 T with its plane perpendicular to the magnetic field. The radius of the loop starts shrinking at 2 mm/s. The induced emf in the loop when the radius is 2 cm isa)4.8 π μVb)0.8 π μVc)1.6 π μVd)3.2 π μVCorrect answer is option 'D'. Can you explain this answer? tests, examples and also practice JEE tests.

|
Explore Courses for JEE exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.






















