CAT Exam > CAT Questions > Twenty five coloured beads are to be arranged...
Start Learning for Free
Twenty five coloured beads are to be arranged in a grid comprising of five rows and five columns. Each cell in the grid must contain exactly one bead. Each bead is coloured either Red, Blue or Green.
While arranging the beads along any of the five rows or along any of the five columns, the rules given below are to be followed:
1. Two adjacent beads along the same row or column are always of different colours.
2. There is at least one Green bead between any two Blue beads along the same row or column.
3. There is at least one Blue and at least one Green bead between any two Red beads along the same row or column.
1. Two adjacent beads along the same row or column are always of different colours.
2. There is at least one Green bead between any two Blue beads along the same row or column.
3. There is at least one Blue and at least one Green bead between any two Red beads along the same row or column.
Every unique, complete arrangement of twenty five beads is called a configuration.
The total number of possible configuration using beads of only two colours is:
Correct answer is '2'. Can you explain this answer?
Most Upvoted Answer
Twenty five coloured beads are to be arranged in a grid comprising of ...
As we need to use only two colours, in any row or column these two coloured beads will be
placed alternately like
placed alternately like

So we cannot place Red coloured beads at position 1 or two as between any two Red beads there must at least two beads (at least one green and at least one Blue). Hence, we can use only Green and Blue coloured beads.
We can have two possible configurations:
Configuration 1: Green bead is placed at top left corner
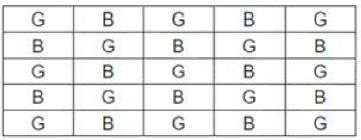
Configuration 2: Blue bead is placed at top left corner
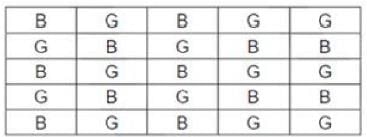
Free Test
FREE
| Start Free Test |
Community Answer
Twenty five coloured beads are to be arranged in a grid comprising of ...
Possible Configurations:
To find the total number of possible configurations using beads of only two colors, we need to consider the different combinations of colors that satisfy the given rules. Let's analyze each rule and its implications on the configurations.
Rule 1: Two adjacent beads along the same row or column are always of different colors.
This rule implies that if we have a row or column with alternating colors, we can extend it to form a valid configuration. For example, if we have a row with the pattern "Red-Blue-Red-Blue-Red", we can add any color in the remaining cells to form a valid configuration.
Rule 2: There is at least one Green bead between any two Blue beads along the same row or column.
This rule implies that we cannot have two consecutive Blue beads in a row or column. We can use this rule to further restrict the possible configurations.
Rule 3: There is at least one Blue and at least one Green bead between any two Red beads along the same row or column.
This rule implies that we cannot have two consecutive Red beads in a row or column. Again, we can use this rule to narrow down the possibilities.
Analysis:
Based on the given rules, let's consider the possible combinations of colors for a row or column:
1. Red-Blue-Red-Blue-Red:
In this pattern, we have alternating Red and Blue beads. We can add any color in the remaining cells to form a valid configuration. The possible configurations for this pattern are:
- Red-Blue-Red-Blue-Red
- Red-Blue-Red-Blue-Green
- Red-Blue-Red-Blue-Blue
- Red-Blue-Red-Blue-Red
2. Red-Blue-Red-Green-Red:
In this pattern, we have alternating Red and Blue beads, with a Green bead in the middle. We can add any color in the remaining cells to form a valid configuration. The possible configurations for this pattern are:
- Red-Blue-Red-Green-Red
- Red-Blue-Red-Green-Green
- Red-Blue-Red-Green-Blue
- Red-Blue-Red-Green-Red
3. Red-Blue-Red-Green-Green:
In this pattern, we have alternating Red and Blue beads, with two Green beads in the middle. We can add any color in the remaining cells to form a valid configuration. The possible configurations for this pattern are:
- Red-Blue-Red-Green-Green
- Red-Blue-Red-Green-Blue
- Red-Blue-Red-Green-Red
Total Possible Configurations:
Combining all the possible configurations from the above patterns, we have:
- Red-Blue-Red-Blue-Red
- Red-Blue-Red-Blue-Green
- Red-Blue-Red-Blue-Blue
- Red-Blue-Red-Blue-Red
- Red-Blue-Red-Green-Red
- Red-Blue-Red-Green-Green
- Red-Blue-Red-Green-Blue
- Red-Blue-Red-Green-Red
There are only two unique configurations:
- Red-Blue-Red-Blue-Green
- Red-Blue-Red-Green-Red
Therefore, the correct answer is '2'.
To find the total number of possible configurations using beads of only two colors, we need to consider the different combinations of colors that satisfy the given rules. Let's analyze each rule and its implications on the configurations.
Rule 1: Two adjacent beads along the same row or column are always of different colors.
This rule implies that if we have a row or column with alternating colors, we can extend it to form a valid configuration. For example, if we have a row with the pattern "Red-Blue-Red-Blue-Red", we can add any color in the remaining cells to form a valid configuration.
Rule 2: There is at least one Green bead between any two Blue beads along the same row or column.
This rule implies that we cannot have two consecutive Blue beads in a row or column. We can use this rule to further restrict the possible configurations.
Rule 3: There is at least one Blue and at least one Green bead between any two Red beads along the same row or column.
This rule implies that we cannot have two consecutive Red beads in a row or column. Again, we can use this rule to narrow down the possibilities.
Analysis:
Based on the given rules, let's consider the possible combinations of colors for a row or column:
1. Red-Blue-Red-Blue-Red:
In this pattern, we have alternating Red and Blue beads. We can add any color in the remaining cells to form a valid configuration. The possible configurations for this pattern are:
- Red-Blue-Red-Blue-Red
- Red-Blue-Red-Blue-Green
- Red-Blue-Red-Blue-Blue
- Red-Blue-Red-Blue-Red
2. Red-Blue-Red-Green-Red:
In this pattern, we have alternating Red and Blue beads, with a Green bead in the middle. We can add any color in the remaining cells to form a valid configuration. The possible configurations for this pattern are:
- Red-Blue-Red-Green-Red
- Red-Blue-Red-Green-Green
- Red-Blue-Red-Green-Blue
- Red-Blue-Red-Green-Red
3. Red-Blue-Red-Green-Green:
In this pattern, we have alternating Red and Blue beads, with two Green beads in the middle. We can add any color in the remaining cells to form a valid configuration. The possible configurations for this pattern are:
- Red-Blue-Red-Green-Green
- Red-Blue-Red-Green-Blue
- Red-Blue-Red-Green-Red
Total Possible Configurations:
Combining all the possible configurations from the above patterns, we have:
- Red-Blue-Red-Blue-Red
- Red-Blue-Red-Blue-Green
- Red-Blue-Red-Blue-Blue
- Red-Blue-Red-Blue-Red
- Red-Blue-Red-Green-Red
- Red-Blue-Red-Green-Green
- Red-Blue-Red-Green-Blue
- Red-Blue-Red-Green-Red
There are only two unique configurations:
- Red-Blue-Red-Blue-Green
- Red-Blue-Red-Green-Red
Therefore, the correct answer is '2'.

|
Explore Courses for CAT exam
|

|
Similar CAT Doubts
Twenty five coloured beads are to be arranged in a grid comprising of five rows and five columns. Each cell in the grid must contain exactly one bead. Each bead is coloured either Red, Blue or Green.While arranging the beads along any of the five rows or along any of the five columns, the rules given below are to be followed:1. Two adjacent beads along the same row or column are always of different colours.2. There is at least one Green bead between any two Blue beads along the same row or column.3. There is at least one Blue and at least one Green bead between any two Red beads along the same row or column.Every unique, complete arrangement of twenty five beads is called a configuration.The total number of possible configuration using beads of only two colours is:Correct answer is '2'. Can you explain this answer?
Question Description
Twenty five coloured beads are to be arranged in a grid comprising of five rows and five columns. Each cell in the grid must contain exactly one bead. Each bead is coloured either Red, Blue or Green.While arranging the beads along any of the five rows or along any of the five columns, the rules given below are to be followed:1. Two adjacent beads along the same row or column are always of different colours.2. There is at least one Green bead between any two Blue beads along the same row or column.3. There is at least one Blue and at least one Green bead between any two Red beads along the same row or column.Every unique, complete arrangement of twenty five beads is called a configuration.The total number of possible configuration using beads of only two colours is:Correct answer is '2'. Can you explain this answer? for CAT 2025 is part of CAT preparation. The Question and answers have been prepared according to the CAT exam syllabus. Information about Twenty five coloured beads are to be arranged in a grid comprising of five rows and five columns. Each cell in the grid must contain exactly one bead. Each bead is coloured either Red, Blue or Green.While arranging the beads along any of the five rows or along any of the five columns, the rules given below are to be followed:1. Two adjacent beads along the same row or column are always of different colours.2. There is at least one Green bead between any two Blue beads along the same row or column.3. There is at least one Blue and at least one Green bead between any two Red beads along the same row or column.Every unique, complete arrangement of twenty five beads is called a configuration.The total number of possible configuration using beads of only two colours is:Correct answer is '2'. Can you explain this answer? covers all topics & solutions for CAT 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Twenty five coloured beads are to be arranged in a grid comprising of five rows and five columns. Each cell in the grid must contain exactly one bead. Each bead is coloured either Red, Blue or Green.While arranging the beads along any of the five rows or along any of the five columns, the rules given below are to be followed:1. Two adjacent beads along the same row or column are always of different colours.2. There is at least one Green bead between any two Blue beads along the same row or column.3. There is at least one Blue and at least one Green bead between any two Red beads along the same row or column.Every unique, complete arrangement of twenty five beads is called a configuration.The total number of possible configuration using beads of only two colours is:Correct answer is '2'. Can you explain this answer?.
Twenty five coloured beads are to be arranged in a grid comprising of five rows and five columns. Each cell in the grid must contain exactly one bead. Each bead is coloured either Red, Blue or Green.While arranging the beads along any of the five rows or along any of the five columns, the rules given below are to be followed:1. Two adjacent beads along the same row or column are always of different colours.2. There is at least one Green bead between any two Blue beads along the same row or column.3. There is at least one Blue and at least one Green bead between any two Red beads along the same row or column.Every unique, complete arrangement of twenty five beads is called a configuration.The total number of possible configuration using beads of only two colours is:Correct answer is '2'. Can you explain this answer? for CAT 2025 is part of CAT preparation. The Question and answers have been prepared according to the CAT exam syllabus. Information about Twenty five coloured beads are to be arranged in a grid comprising of five rows and five columns. Each cell in the grid must contain exactly one bead. Each bead is coloured either Red, Blue or Green.While arranging the beads along any of the five rows or along any of the five columns, the rules given below are to be followed:1. Two adjacent beads along the same row or column are always of different colours.2. There is at least one Green bead between any two Blue beads along the same row or column.3. There is at least one Blue and at least one Green bead between any two Red beads along the same row or column.Every unique, complete arrangement of twenty five beads is called a configuration.The total number of possible configuration using beads of only two colours is:Correct answer is '2'. Can you explain this answer? covers all topics & solutions for CAT 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Twenty five coloured beads are to be arranged in a grid comprising of five rows and five columns. Each cell in the grid must contain exactly one bead. Each bead is coloured either Red, Blue or Green.While arranging the beads along any of the five rows or along any of the five columns, the rules given below are to be followed:1. Two adjacent beads along the same row or column are always of different colours.2. There is at least one Green bead between any two Blue beads along the same row or column.3. There is at least one Blue and at least one Green bead between any two Red beads along the same row or column.Every unique, complete arrangement of twenty five beads is called a configuration.The total number of possible configuration using beads of only two colours is:Correct answer is '2'. Can you explain this answer?.
Solutions for Twenty five coloured beads are to be arranged in a grid comprising of five rows and five columns. Each cell in the grid must contain exactly one bead. Each bead is coloured either Red, Blue or Green.While arranging the beads along any of the five rows or along any of the five columns, the rules given below are to be followed:1. Two adjacent beads along the same row or column are always of different colours.2. There is at least one Green bead between any two Blue beads along the same row or column.3. There is at least one Blue and at least one Green bead between any two Red beads along the same row or column.Every unique, complete arrangement of twenty five beads is called a configuration.The total number of possible configuration using beads of only two colours is:Correct answer is '2'. Can you explain this answer? in English & in Hindi are available as part of our courses for CAT.
Download more important topics, notes, lectures and mock test series for CAT Exam by signing up for free.
Here you can find the meaning of Twenty five coloured beads are to be arranged in a grid comprising of five rows and five columns. Each cell in the grid must contain exactly one bead. Each bead is coloured either Red, Blue or Green.While arranging the beads along any of the five rows or along any of the five columns, the rules given below are to be followed:1. Two adjacent beads along the same row or column are always of different colours.2. There is at least one Green bead between any two Blue beads along the same row or column.3. There is at least one Blue and at least one Green bead between any two Red beads along the same row or column.Every unique, complete arrangement of twenty five beads is called a configuration.The total number of possible configuration using beads of only two colours is:Correct answer is '2'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
Twenty five coloured beads are to be arranged in a grid comprising of five rows and five columns. Each cell in the grid must contain exactly one bead. Each bead is coloured either Red, Blue or Green.While arranging the beads along any of the five rows or along any of the five columns, the rules given below are to be followed:1. Two adjacent beads along the same row or column are always of different colours.2. There is at least one Green bead between any two Blue beads along the same row or column.3. There is at least one Blue and at least one Green bead between any two Red beads along the same row or column.Every unique, complete arrangement of twenty five beads is called a configuration.The total number of possible configuration using beads of only two colours is:Correct answer is '2'. Can you explain this answer?, a detailed solution for Twenty five coloured beads are to be arranged in a grid comprising of five rows and five columns. Each cell in the grid must contain exactly one bead. Each bead is coloured either Red, Blue or Green.While arranging the beads along any of the five rows or along any of the five columns, the rules given below are to be followed:1. Two adjacent beads along the same row or column are always of different colours.2. There is at least one Green bead between any two Blue beads along the same row or column.3. There is at least one Blue and at least one Green bead between any two Red beads along the same row or column.Every unique, complete arrangement of twenty five beads is called a configuration.The total number of possible configuration using beads of only two colours is:Correct answer is '2'. Can you explain this answer? has been provided alongside types of Twenty five coloured beads are to be arranged in a grid comprising of five rows and five columns. Each cell in the grid must contain exactly one bead. Each bead is coloured either Red, Blue or Green.While arranging the beads along any of the five rows or along any of the five columns, the rules given below are to be followed:1. Two adjacent beads along the same row or column are always of different colours.2. There is at least one Green bead between any two Blue beads along the same row or column.3. There is at least one Blue and at least one Green bead between any two Red beads along the same row or column.Every unique, complete arrangement of twenty five beads is called a configuration.The total number of possible configuration using beads of only two colours is:Correct answer is '2'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice Twenty five coloured beads are to be arranged in a grid comprising of five rows and five columns. Each cell in the grid must contain exactly one bead. Each bead is coloured either Red, Blue or Green.While arranging the beads along any of the five rows or along any of the five columns, the rules given below are to be followed:1. Two adjacent beads along the same row or column are always of different colours.2. There is at least one Green bead between any two Blue beads along the same row or column.3. There is at least one Blue and at least one Green bead between any two Red beads along the same row or column.Every unique, complete arrangement of twenty five beads is called a configuration.The total number of possible configuration using beads of only two colours is:Correct answer is '2'. Can you explain this answer? tests, examples and also practice CAT tests.

|
Explore Courses for CAT exam
|

|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
























