CAT Exam > CAT Questions > Two players are playing a game called Pick an...
Start Learning for Free
Two players are playing a game called 'Pick an alpha'. In this game, there are 2 variables, the first one is 'a', the starting alphabet, and the second one 'n' the maximum range of alphabets starting from 'a'. The game proceeds as follows. One of the players picks an alphabet from the first n alphabets starting from 'a', say 'm', now the second player has to pick an alphabet from the first n alphabets starting from the alphabet just after 'm', and in a similar way, it continues till one player picks the alphabet Z. The player to choose Z loses the game. If at any point during the game, less than n alphabets remain, the player has to choose from these remaining alphabets. Both the players pick alphabets that maximise their chance to win.
Suppose, the value of 'a' is G and the value of n is 3. So, the first player has to pick an alphabet among G, H, I. Suppose he picks I. Then the second player has to pick among J, K and L. It continues in a similar fashion.
Based on the information given above, answer the questions that follow.
Q. Suppose the rules of the game are changed such that the one who picks Z wins. What should the player who is starting the game pick in his first chance so that he definitely wins? a = A, n = 4.
- a)A
- b)B
- c)C
- d)D
Correct answer is option 'A'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
Two players are playing a game called Pick an alpha. In this game, the...
Let us think of it this way. The alphabets from A to Z can be thought of as numbers from 1 to 26 from which the two players pick.
If the player starting the game wants to win, he has to make sure that the other one is left with only one number, i.e the last number 26, in his last chance, which he has to pick necessarily.
Now let us consider a consecutive pair of chances. Whichever number a player picks from the n different choices, the second player can always pick a number such that to adjust it and make a sum of n + 1.
So, suppose, n = 6. If the first player chooses 3, the second player can choose 4 to make the sum 7.
So,
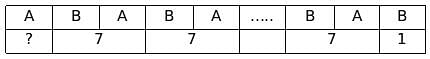
The sum of all numbers should be the total number of characters, that is the alphabet representing 'a' to the alphabet 'Z'. In this case,
26 - ( 3 x 7 + 1) = 4, i.e D.
In this question,
If a = A, this means a = 1.
n = 4
n + 1 = 5
Total number of alphabets = 26
So, he should choose,
26 - ( 5 x 5 ) = 26 - 25 = 1
Hence, A.
Alternate Solution:
Let the player who starts be P1 and the other be P2. We have to figure out which starting positions are definite losing positions.
Z is a winning position.
Any starting position from W to Z, you pick Z ensuring that you win.
V is a losing position because the opponent will fall in the winning position W-Z.
R-U are winning positions because the opponent has to choose V which is a losing position.
So we see the pattern that V, Q, L, G, B are losing positions. The corresponding numbers are 22, 17, 12, 7, 2.
As B is definitely losing position, the first player should choose A to put the opponent on B.
Most Upvoted Answer
Two players are playing a game called Pick an alpha. In this game, the...
Understanding the Game Mechanics
The game involves two players alternately picking letters from a specified range. The goal is to be the player who picks the letter Z, as this player wins according to the new rules.
Game Setup
- Starting letter (a): A
- Range of letters to choose from (n): 4
- Letters available: A, B, C, D, E, F, G, H, I, J, K, L, M, N, O, P, Q, R, S, T, U, V, W, X, Y, Z
Strategies for Winning
To determine the best starting move, players must consider the outcomes after each possible choice:
1. First Move Options: The first player can choose from A, B, C, or D.
2. Subsequent Moves: Depending on the first player's choice, the second player will then have their own set of letters to choose from, and the game continues until a player picks Z.
Optimal First Move
- Choosing A: If the first player picks A, the options for the second player become B, C, D, or E. This sequence continues, ultimately allowing the first player to control the flow of the game.
- Winning Path: By consistently forcing the opponent into positions where they have fewer advantageous choices, the first player can maneuver toward picking Z.
Conclusion
By starting with the letter A, the first player sets themselves up for a strategic advantage, ensuring they can always respond in a way that maintains control over the game's direction. This leads to a guaranteed victory, highlighting the importance of the initial choice in structured games like this.
The game involves two players alternately picking letters from a specified range. The goal is to be the player who picks the letter Z, as this player wins according to the new rules.
Game Setup
- Starting letter (a): A
- Range of letters to choose from (n): 4
- Letters available: A, B, C, D, E, F, G, H, I, J, K, L, M, N, O, P, Q, R, S, T, U, V, W, X, Y, Z
Strategies for Winning
To determine the best starting move, players must consider the outcomes after each possible choice:
1. First Move Options: The first player can choose from A, B, C, or D.
2. Subsequent Moves: Depending on the first player's choice, the second player will then have their own set of letters to choose from, and the game continues until a player picks Z.
Optimal First Move
- Choosing A: If the first player picks A, the options for the second player become B, C, D, or E. This sequence continues, ultimately allowing the first player to control the flow of the game.
- Winning Path: By consistently forcing the opponent into positions where they have fewer advantageous choices, the first player can maneuver toward picking Z.
Conclusion
By starting with the letter A, the first player sets themselves up for a strategic advantage, ensuring they can always respond in a way that maintains control over the game's direction. This leads to a guaranteed victory, highlighting the importance of the initial choice in structured games like this.
Attention CAT Students!
To make sure you are not studying endlessly, EduRev has designed CAT study material, with Structured Courses, Videos, & Test Series. Plus get personalized analysis, doubt solving and improvement plans to achieve a great score in CAT.

|
Explore Courses for CAT exam
|

|
Similar CAT Doubts
Two players are playing a game called Pick an alpha. In this game, there are 2 variables, the first one is a, the starting alphabet, and the second one n the maximum range of alphabets starting from a. The game proceeds as follows. One of the players picks an alphabet from the first n alphabets starting from a, say m, now the second player has to pick an alphabet from the first n alphabets starting from the alphabet just afterm, and in a similar way, it continues till one player picks the alphabet Z. The player to choose Z loses the game. If at any point during the game, less than n alphabets remain, the player has to choose from these remaining alphabets.Both the players pick alphabets that maximise their chance to win.Suppose, the value of a is G and the value of n is 3. So, the first player has to pick an alphabet among G, H, I. Suppose he picks I. Then the second player has to pick among J, K and L. It continues in a similar fashion.Based on the information given above, answer the questions that follow.Q. Suppose the rules of the game are changed such that the one who picks Z wins. What should the player who is starting the game pick in his first chance so that he definitely wins? a = A, n = 4.a)Ab)Bc)Cd)DCorrect answer is option 'A'. Can you explain this answer?
Question Description
Two players are playing a game called Pick an alpha. In this game, there are 2 variables, the first one is a, the starting alphabet, and the second one n the maximum range of alphabets starting from a. The game proceeds as follows. One of the players picks an alphabet from the first n alphabets starting from a, say m, now the second player has to pick an alphabet from the first n alphabets starting from the alphabet just afterm, and in a similar way, it continues till one player picks the alphabet Z. The player to choose Z loses the game. If at any point during the game, less than n alphabets remain, the player has to choose from these remaining alphabets.Both the players pick alphabets that maximise their chance to win.Suppose, the value of a is G and the value of n is 3. So, the first player has to pick an alphabet among G, H, I. Suppose he picks I. Then the second player has to pick among J, K and L. It continues in a similar fashion.Based on the information given above, answer the questions that follow.Q. Suppose the rules of the game are changed such that the one who picks Z wins. What should the player who is starting the game pick in his first chance so that he definitely wins? a = A, n = 4.a)Ab)Bc)Cd)DCorrect answer is option 'A'. Can you explain this answer? for CAT 2024 is part of CAT preparation. The Question and answers have been prepared according to the CAT exam syllabus. Information about Two players are playing a game called Pick an alpha. In this game, there are 2 variables, the first one is a, the starting alphabet, and the second one n the maximum range of alphabets starting from a. The game proceeds as follows. One of the players picks an alphabet from the first n alphabets starting from a, say m, now the second player has to pick an alphabet from the first n alphabets starting from the alphabet just afterm, and in a similar way, it continues till one player picks the alphabet Z. The player to choose Z loses the game. If at any point during the game, less than n alphabets remain, the player has to choose from these remaining alphabets.Both the players pick alphabets that maximise their chance to win.Suppose, the value of a is G and the value of n is 3. So, the first player has to pick an alphabet among G, H, I. Suppose he picks I. Then the second player has to pick among J, K and L. It continues in a similar fashion.Based on the information given above, answer the questions that follow.Q. Suppose the rules of the game are changed such that the one who picks Z wins. What should the player who is starting the game pick in his first chance so that he definitely wins? a = A, n = 4.a)Ab)Bc)Cd)DCorrect answer is option 'A'. Can you explain this answer? covers all topics & solutions for CAT 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Two players are playing a game called Pick an alpha. In this game, there are 2 variables, the first one is a, the starting alphabet, and the second one n the maximum range of alphabets starting from a. The game proceeds as follows. One of the players picks an alphabet from the first n alphabets starting from a, say m, now the second player has to pick an alphabet from the first n alphabets starting from the alphabet just afterm, and in a similar way, it continues till one player picks the alphabet Z. The player to choose Z loses the game. If at any point during the game, less than n alphabets remain, the player has to choose from these remaining alphabets.Both the players pick alphabets that maximise their chance to win.Suppose, the value of a is G and the value of n is 3. So, the first player has to pick an alphabet among G, H, I. Suppose he picks I. Then the second player has to pick among J, K and L. It continues in a similar fashion.Based on the information given above, answer the questions that follow.Q. Suppose the rules of the game are changed such that the one who picks Z wins. What should the player who is starting the game pick in his first chance so that he definitely wins? a = A, n = 4.a)Ab)Bc)Cd)DCorrect answer is option 'A'. Can you explain this answer?.
Two players are playing a game called Pick an alpha. In this game, there are 2 variables, the first one is a, the starting alphabet, and the second one n the maximum range of alphabets starting from a. The game proceeds as follows. One of the players picks an alphabet from the first n alphabets starting from a, say m, now the second player has to pick an alphabet from the first n alphabets starting from the alphabet just afterm, and in a similar way, it continues till one player picks the alphabet Z. The player to choose Z loses the game. If at any point during the game, less than n alphabets remain, the player has to choose from these remaining alphabets.Both the players pick alphabets that maximise their chance to win.Suppose, the value of a is G and the value of n is 3. So, the first player has to pick an alphabet among G, H, I. Suppose he picks I. Then the second player has to pick among J, K and L. It continues in a similar fashion.Based on the information given above, answer the questions that follow.Q. Suppose the rules of the game are changed such that the one who picks Z wins. What should the player who is starting the game pick in his first chance so that he definitely wins? a = A, n = 4.a)Ab)Bc)Cd)DCorrect answer is option 'A'. Can you explain this answer? for CAT 2024 is part of CAT preparation. The Question and answers have been prepared according to the CAT exam syllabus. Information about Two players are playing a game called Pick an alpha. In this game, there are 2 variables, the first one is a, the starting alphabet, and the second one n the maximum range of alphabets starting from a. The game proceeds as follows. One of the players picks an alphabet from the first n alphabets starting from a, say m, now the second player has to pick an alphabet from the first n alphabets starting from the alphabet just afterm, and in a similar way, it continues till one player picks the alphabet Z. The player to choose Z loses the game. If at any point during the game, less than n alphabets remain, the player has to choose from these remaining alphabets.Both the players pick alphabets that maximise their chance to win.Suppose, the value of a is G and the value of n is 3. So, the first player has to pick an alphabet among G, H, I. Suppose he picks I. Then the second player has to pick among J, K and L. It continues in a similar fashion.Based on the information given above, answer the questions that follow.Q. Suppose the rules of the game are changed such that the one who picks Z wins. What should the player who is starting the game pick in his first chance so that he definitely wins? a = A, n = 4.a)Ab)Bc)Cd)DCorrect answer is option 'A'. Can you explain this answer? covers all topics & solutions for CAT 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Two players are playing a game called Pick an alpha. In this game, there are 2 variables, the first one is a, the starting alphabet, and the second one n the maximum range of alphabets starting from a. The game proceeds as follows. One of the players picks an alphabet from the first n alphabets starting from a, say m, now the second player has to pick an alphabet from the first n alphabets starting from the alphabet just afterm, and in a similar way, it continues till one player picks the alphabet Z. The player to choose Z loses the game. If at any point during the game, less than n alphabets remain, the player has to choose from these remaining alphabets.Both the players pick alphabets that maximise their chance to win.Suppose, the value of a is G and the value of n is 3. So, the first player has to pick an alphabet among G, H, I. Suppose he picks I. Then the second player has to pick among J, K and L. It continues in a similar fashion.Based on the information given above, answer the questions that follow.Q. Suppose the rules of the game are changed such that the one who picks Z wins. What should the player who is starting the game pick in his first chance so that he definitely wins? a = A, n = 4.a)Ab)Bc)Cd)DCorrect answer is option 'A'. Can you explain this answer?.
Solutions for Two players are playing a game called Pick an alpha. In this game, there are 2 variables, the first one is a, the starting alphabet, and the second one n the maximum range of alphabets starting from a. The game proceeds as follows. One of the players picks an alphabet from the first n alphabets starting from a, say m, now the second player has to pick an alphabet from the first n alphabets starting from the alphabet just afterm, and in a similar way, it continues till one player picks the alphabet Z. The player to choose Z loses the game. If at any point during the game, less than n alphabets remain, the player has to choose from these remaining alphabets.Both the players pick alphabets that maximise their chance to win.Suppose, the value of a is G and the value of n is 3. So, the first player has to pick an alphabet among G, H, I. Suppose he picks I. Then the second player has to pick among J, K and L. It continues in a similar fashion.Based on the information given above, answer the questions that follow.Q. Suppose the rules of the game are changed such that the one who picks Z wins. What should the player who is starting the game pick in his first chance so that he definitely wins? a = A, n = 4.a)Ab)Bc)Cd)DCorrect answer is option 'A'. Can you explain this answer? in English & in Hindi are available as part of our courses for CAT.
Download more important topics, notes, lectures and mock test series for CAT Exam by signing up for free.
Here you can find the meaning of Two players are playing a game called Pick an alpha. In this game, there are 2 variables, the first one is a, the starting alphabet, and the second one n the maximum range of alphabets starting from a. The game proceeds as follows. One of the players picks an alphabet from the first n alphabets starting from a, say m, now the second player has to pick an alphabet from the first n alphabets starting from the alphabet just afterm, and in a similar way, it continues till one player picks the alphabet Z. The player to choose Z loses the game. If at any point during the game, less than n alphabets remain, the player has to choose from these remaining alphabets.Both the players pick alphabets that maximise their chance to win.Suppose, the value of a is G and the value of n is 3. So, the first player has to pick an alphabet among G, H, I. Suppose he picks I. Then the second player has to pick among J, K and L. It continues in a similar fashion.Based on the information given above, answer the questions that follow.Q. Suppose the rules of the game are changed such that the one who picks Z wins. What should the player who is starting the game pick in his first chance so that he definitely wins? a = A, n = 4.a)Ab)Bc)Cd)DCorrect answer is option 'A'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
Two players are playing a game called Pick an alpha. In this game, there are 2 variables, the first one is a, the starting alphabet, and the second one n the maximum range of alphabets starting from a. The game proceeds as follows. One of the players picks an alphabet from the first n alphabets starting from a, say m, now the second player has to pick an alphabet from the first n alphabets starting from the alphabet just afterm, and in a similar way, it continues till one player picks the alphabet Z. The player to choose Z loses the game. If at any point during the game, less than n alphabets remain, the player has to choose from these remaining alphabets.Both the players pick alphabets that maximise their chance to win.Suppose, the value of a is G and the value of n is 3. So, the first player has to pick an alphabet among G, H, I. Suppose he picks I. Then the second player has to pick among J, K and L. It continues in a similar fashion.Based on the information given above, answer the questions that follow.Q. Suppose the rules of the game are changed such that the one who picks Z wins. What should the player who is starting the game pick in his first chance so that he definitely wins? a = A, n = 4.a)Ab)Bc)Cd)DCorrect answer is option 'A'. Can you explain this answer?, a detailed solution for Two players are playing a game called Pick an alpha. In this game, there are 2 variables, the first one is a, the starting alphabet, and the second one n the maximum range of alphabets starting from a. The game proceeds as follows. One of the players picks an alphabet from the first n alphabets starting from a, say m, now the second player has to pick an alphabet from the first n alphabets starting from the alphabet just afterm, and in a similar way, it continues till one player picks the alphabet Z. The player to choose Z loses the game. If at any point during the game, less than n alphabets remain, the player has to choose from these remaining alphabets.Both the players pick alphabets that maximise their chance to win.Suppose, the value of a is G and the value of n is 3. So, the first player has to pick an alphabet among G, H, I. Suppose he picks I. Then the second player has to pick among J, K and L. It continues in a similar fashion.Based on the information given above, answer the questions that follow.Q. Suppose the rules of the game are changed such that the one who picks Z wins. What should the player who is starting the game pick in his first chance so that he definitely wins? a = A, n = 4.a)Ab)Bc)Cd)DCorrect answer is option 'A'. Can you explain this answer? has been provided alongside types of Two players are playing a game called Pick an alpha. In this game, there are 2 variables, the first one is a, the starting alphabet, and the second one n the maximum range of alphabets starting from a. The game proceeds as follows. One of the players picks an alphabet from the first n alphabets starting from a, say m, now the second player has to pick an alphabet from the first n alphabets starting from the alphabet just afterm, and in a similar way, it continues till one player picks the alphabet Z. The player to choose Z loses the game. If at any point during the game, less than n alphabets remain, the player has to choose from these remaining alphabets.Both the players pick alphabets that maximise their chance to win.Suppose, the value of a is G and the value of n is 3. So, the first player has to pick an alphabet among G, H, I. Suppose he picks I. Then the second player has to pick among J, K and L. It continues in a similar fashion.Based on the information given above, answer the questions that follow.Q. Suppose the rules of the game are changed such that the one who picks Z wins. What should the player who is starting the game pick in his first chance so that he definitely wins? a = A, n = 4.a)Ab)Bc)Cd)DCorrect answer is option 'A'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice Two players are playing a game called Pick an alpha. In this game, there are 2 variables, the first one is a, the starting alphabet, and the second one n the maximum range of alphabets starting from a. The game proceeds as follows. One of the players picks an alphabet from the first n alphabets starting from a, say m, now the second player has to pick an alphabet from the first n alphabets starting from the alphabet just afterm, and in a similar way, it continues till one player picks the alphabet Z. The player to choose Z loses the game. If at any point during the game, less than n alphabets remain, the player has to choose from these remaining alphabets.Both the players pick alphabets that maximise their chance to win.Suppose, the value of a is G and the value of n is 3. So, the first player has to pick an alphabet among G, H, I. Suppose he picks I. Then the second player has to pick among J, K and L. It continues in a similar fashion.Based on the information given above, answer the questions that follow.Q. Suppose the rules of the game are changed such that the one who picks Z wins. What should the player who is starting the game pick in his first chance so that he definitely wins? a = A, n = 4.a)Ab)Bc)Cd)DCorrect answer is option 'A'. Can you explain this answer? tests, examples and also practice CAT tests.

|
Explore Courses for CAT exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
























