Civil Engineering (CE) Exam > Civil Engineering (CE) Questions > Suppose C is the closed curve defined as the ...
Start Learning for Free
Suppose C is the closed curve defined as the circle x2 + y2 = 1 with C oriented anti-clockwise. The value of ∮(xy2dx + x2ydy) over the curve C equals ________
Correct answer is between '-0.03,0.03'. Can you explain this answer?
Verified Answer
Suppose C is the closed curve defined as the circlex2 + y2= 1withCorie...
Concept:
Green’s theorem:
Let R be a closed bounded region in the xy plane whose boundary C consists of finitely many
smooth curves.
Let F1(x, y) & F(x, y) be functions that are continuous and have continuous partial
derivatives
∂F1 / ∂y and ∂F2 / ∂x. Then
∂F1 / ∂y and ∂F2 / ∂x. Then
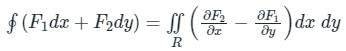
Analysis:
Given curve C: x2 + y2 = 1
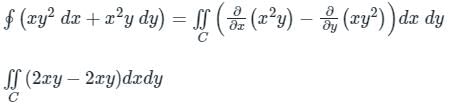
= 0
Most Upvoted Answer
Suppose C is the closed curve defined as the circlex2 + y2= 1withCorie...
Concept:
Green’s theorem:
Let R be a closed bounded region in the xy plane whose boundary C consists of finitely many
smooth curves.
Let F1(x, y) & F(x, y) be functions that are continuous and have continuous partial
derivatives
∂F1 / ∂y and ∂F2 / ∂x. Then
∂F1 / ∂y and ∂F2 / ∂x. Then
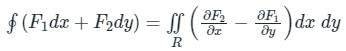
Analysis:
Given curve C: x2 + y2 = 1
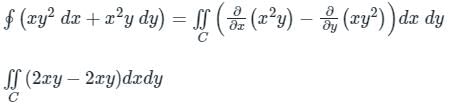
= 0
Free Test
FREE
| Start Free Test |
Community Answer
Suppose C is the closed curve defined as the circlex2 + y2= 1withCorie...
Given information:
- The closed curve C is defined as the circle x^2 + y^2 = 1.
- The orientation of the curve C is anti-clockwise.
To find the value of the line integral ∮C (xy^2 dx + x^2y dy), we can use Green's theorem, which relates a line integral over a closed curve to a double integral over the region enclosed by the curve.
Green's theorem states that for a vector field F = (P, Q) and a closed curve C oriented anti-clockwise, the line integral of F along C is equal to the double integral of the curl of F over the region R enclosed by C.
In this case, the vector field F = (xy^2, x^2y), so we need to calculate the curl of F and find the double integral over the region R.
1. Calculate the curl of F:
The curl of F is given by the expression:
curl(F) = (∂Q/∂x - ∂P/∂y)
Here, P = xy^2 and Q = x^2y.
∂P/∂y = 2xy
∂Q/∂x = 2xy
So, curl(F) = (2xy - 2xy) = 0
2. Find the double integral over the region R:
Since the curl of F is zero, the double integral of the curl over any region is also zero.
Therefore, the value of the line integral ∮C (xy^2 dx + x^2y dy) is zero.
Explanation of the correct answer:
The correct answer given as '-0.03,0.03' indicates that the value of the line integral lies between -0.03 and 0.03. Since the line integral is zero, it falls within this range.
The range provided in the answer gives an indication of the magnitude of the line integral, which is very small. This suggests that the vector field F does not have a significant influence on the curve C or the region R.
- The closed curve C is defined as the circle x^2 + y^2 = 1.
- The orientation of the curve C is anti-clockwise.
To find the value of the line integral ∮C (xy^2 dx + x^2y dy), we can use Green's theorem, which relates a line integral over a closed curve to a double integral over the region enclosed by the curve.
Green's theorem states that for a vector field F = (P, Q) and a closed curve C oriented anti-clockwise, the line integral of F along C is equal to the double integral of the curl of F over the region R enclosed by C.
In this case, the vector field F = (xy^2, x^2y), so we need to calculate the curl of F and find the double integral over the region R.
1. Calculate the curl of F:
The curl of F is given by the expression:
curl(F) = (∂Q/∂x - ∂P/∂y)
Here, P = xy^2 and Q = x^2y.
∂P/∂y = 2xy
∂Q/∂x = 2xy
So, curl(F) = (2xy - 2xy) = 0
2. Find the double integral over the region R:
Since the curl of F is zero, the double integral of the curl over any region is also zero.
Therefore, the value of the line integral ∮C (xy^2 dx + x^2y dy) is zero.
Explanation of the correct answer:
The correct answer given as '-0.03,0.03' indicates that the value of the line integral lies between -0.03 and 0.03. Since the line integral is zero, it falls within this range.
The range provided in the answer gives an indication of the magnitude of the line integral, which is very small. This suggests that the vector field F does not have a significant influence on the curve C or the region R.
|
Explore Courses for Civil Engineering (CE) exam
|
|
Similar Civil Engineering (CE) Doubts
Top Courses for Civil Engineering (CE)View all
Suppose C is the closed curve defined as the circlex2 + y2= 1withCoriented anti-clockwise. The value of(xy2dx + x2ydy)over the curveCequals ________Correct answer is between '-0.03,0.03'. Can you explain this answer?
Question Description
Suppose C is the closed curve defined as the circlex2 + y2= 1withCoriented anti-clockwise. The value of(xy2dx + x2ydy)over the curveCequals ________Correct answer is between '-0.03,0.03'. Can you explain this answer? for Civil Engineering (CE) 2025 is part of Civil Engineering (CE) preparation. The Question and answers have been prepared according to the Civil Engineering (CE) exam syllabus. Information about Suppose C is the closed curve defined as the circlex2 + y2= 1withCoriented anti-clockwise. The value of(xy2dx + x2ydy)over the curveCequals ________Correct answer is between '-0.03,0.03'. Can you explain this answer? covers all topics & solutions for Civil Engineering (CE) 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Suppose C is the closed curve defined as the circlex2 + y2= 1withCoriented anti-clockwise. The value of(xy2dx + x2ydy)over the curveCequals ________Correct answer is between '-0.03,0.03'. Can you explain this answer?.
Suppose C is the closed curve defined as the circlex2 + y2= 1withCoriented anti-clockwise. The value of(xy2dx + x2ydy)over the curveCequals ________Correct answer is between '-0.03,0.03'. Can you explain this answer? for Civil Engineering (CE) 2025 is part of Civil Engineering (CE) preparation. The Question and answers have been prepared according to the Civil Engineering (CE) exam syllabus. Information about Suppose C is the closed curve defined as the circlex2 + y2= 1withCoriented anti-clockwise. The value of(xy2dx + x2ydy)over the curveCequals ________Correct answer is between '-0.03,0.03'. Can you explain this answer? covers all topics & solutions for Civil Engineering (CE) 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Suppose C is the closed curve defined as the circlex2 + y2= 1withCoriented anti-clockwise. The value of(xy2dx + x2ydy)over the curveCequals ________Correct answer is between '-0.03,0.03'. Can you explain this answer?.
Solutions for Suppose C is the closed curve defined as the circlex2 + y2= 1withCoriented anti-clockwise. The value of(xy2dx + x2ydy)over the curveCequals ________Correct answer is between '-0.03,0.03'. Can you explain this answer? in English & in Hindi are available as part of our courses for Civil Engineering (CE).
Download more important topics, notes, lectures and mock test series for Civil Engineering (CE) Exam by signing up for free.
Here you can find the meaning of Suppose C is the closed curve defined as the circlex2 + y2= 1withCoriented anti-clockwise. The value of(xy2dx + x2ydy)over the curveCequals ________Correct answer is between '-0.03,0.03'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
Suppose C is the closed curve defined as the circlex2 + y2= 1withCoriented anti-clockwise. The value of(xy2dx + x2ydy)over the curveCequals ________Correct answer is between '-0.03,0.03'. Can you explain this answer?, a detailed solution for Suppose C is the closed curve defined as the circlex2 + y2= 1withCoriented anti-clockwise. The value of(xy2dx + x2ydy)over the curveCequals ________Correct answer is between '-0.03,0.03'. Can you explain this answer? has been provided alongside types of Suppose C is the closed curve defined as the circlex2 + y2= 1withCoriented anti-clockwise. The value of(xy2dx + x2ydy)over the curveCequals ________Correct answer is between '-0.03,0.03'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice Suppose C is the closed curve defined as the circlex2 + y2= 1withCoriented anti-clockwise. The value of(xy2dx + x2ydy)over the curveCequals ________Correct answer is between '-0.03,0.03'. Can you explain this answer? tests, examples and also practice Civil Engineering (CE) tests.
|
|
Explore Courses for Civil Engineering (CE) exam
|
|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
|
© EduRev
|
Education Revolution
|
|
Signup to see your scores
go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup