Civil Engineering (CE) Exam > Civil Engineering (CE) Questions > If the permissible stress in steel in tension...
Start Learning for Free
If the permissible stress in steel in tension is 140 N / mm2, then the depth of neutral axis for a single reinforced rectangular balanced section will be
- a)0.45d
- b)0.30d
- c)0.40d
- d)0.35d
Correct answer is option 'C'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Most Upvoted Answer
If the permissible stress in steel in tension is 140 N / mm2, then the...
A balanced section is one in which the area of tension steel is such that the failure of both concrete and steel occurs simultaneously.
For WSM method:
The critical depth of the neutral axis is given by –
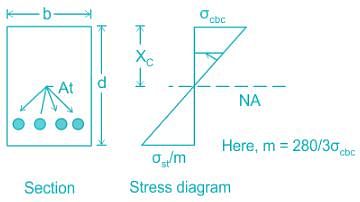
From stress distribution diagram,
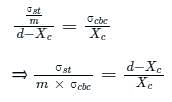
Where, Xc = kd (k = Neutral axis depth factor for balanced section)
Put the value of modular ratio,
Thus the neutral axis factor for the balanced section depends only on σst and is independent of σcbc.
Calculation:
σst = 140 N / mm2
Neutral axis depth factor for balanced section
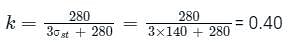
Hence, the depth of neutral axis for a single reinforced rectangular balanced section is 0.40d.
For WSM method:
The critical depth of the neutral axis is given by –
From stress distribution diagram,
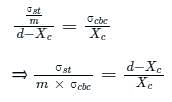
Where, Xc = kd (k = Neutral axis depth factor for balanced section)
Put the value of modular ratio,
Thus the neutral axis factor for the balanced section depends only on σst and is independent of σcbc.
Calculation:
σst = 140 N / mm2
Neutral axis depth factor for balanced section
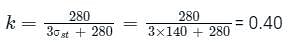
Hence, the depth of neutral axis for a single reinforced rectangular balanced section is 0.40d.
Free Test
FREE
| Start Free Test |
Community Answer
If the permissible stress in steel in tension is 140 N / mm2, then the...
Explanation:
In a reinforced concrete beam, the neutral axis is the line that separates the compression zone (concrete) from the tension zone (steel reinforcement). The depth of the neutral axis is denoted by 'x'.
The permissible stress in steel in tension is given as 140 N/mm².
For a single reinforced rectangular balanced section, the depth of the neutral axis can be determined using the following formula:
x = (σ_st / σ_c) * d
Where:
x = depth of the neutral axis
σ_st = permissible stress in steel in tension (140 N/mm²)
σ_c = permissible stress in concrete in compression (usually taken as 0.45√fck for M20 grade concrete, where fck is the characteristic compressive strength of concrete)
d = effective depth of the beam
Since the question does not provide the value of σ_c or the grade of concrete, we assume it to be M20 grade concrete.
Calculation:
Given: σ_st = 140 N/mm²
Assuming M20 grade concrete, we have σ_c = 0.45√fck = 0.45√20 = 0.45 * 4.47 = 2.01 N/mm²
Substituting the values in the formula, we get:
x = (140 / 2.01) * d
Simplifying,
x ≈ 69.65 * d
Therefore, the depth of the neutral axis (x) for a single reinforced rectangular balanced section is approximately 0.40d (where d is the effective depth of the beam).
Hence, the correct answer is option 'C' (0.40d).
In a reinforced concrete beam, the neutral axis is the line that separates the compression zone (concrete) from the tension zone (steel reinforcement). The depth of the neutral axis is denoted by 'x'.
The permissible stress in steel in tension is given as 140 N/mm².
For a single reinforced rectangular balanced section, the depth of the neutral axis can be determined using the following formula:
x = (σ_st / σ_c) * d
Where:
x = depth of the neutral axis
σ_st = permissible stress in steel in tension (140 N/mm²)
σ_c = permissible stress in concrete in compression (usually taken as 0.45√fck for M20 grade concrete, where fck is the characteristic compressive strength of concrete)
d = effective depth of the beam
Since the question does not provide the value of σ_c or the grade of concrete, we assume it to be M20 grade concrete.
Calculation:
Given: σ_st = 140 N/mm²
Assuming M20 grade concrete, we have σ_c = 0.45√fck = 0.45√20 = 0.45 * 4.47 = 2.01 N/mm²
Substituting the values in the formula, we get:
x = (140 / 2.01) * d
Simplifying,
x ≈ 69.65 * d
Therefore, the depth of the neutral axis (x) for a single reinforced rectangular balanced section is approximately 0.40d (where d is the effective depth of the beam).
Hence, the correct answer is option 'C' (0.40d).
Attention Civil Engineering (CE) Students!
To make sure you are not studying endlessly, EduRev has designed Civil Engineering (CE) study material, with Structured Courses, Videos, & Test Series. Plus get personalized analysis, doubt solving and improvement plans to achieve a great score in Civil Engineering (CE).
|
Explore Courses for Civil Engineering (CE) exam
|
|
Similar Civil Engineering (CE) Doubts
Top Courses for Civil Engineering (CE)View all
If the permissible stress in steel in tension is 140 N / mm2, then the depth of neutral axis for a single reinforced rectangular balanced section will bea)0.45db)0.30dc)0.40dd)0.35dCorrect answer is option 'C'. Can you explain this answer?
Question Description
If the permissible stress in steel in tension is 140 N / mm2, then the depth of neutral axis for a single reinforced rectangular balanced section will bea)0.45db)0.30dc)0.40dd)0.35dCorrect answer is option 'C'. Can you explain this answer? for Civil Engineering (CE) 2024 is part of Civil Engineering (CE) preparation. The Question and answers have been prepared according to the Civil Engineering (CE) exam syllabus. Information about If the permissible stress in steel in tension is 140 N / mm2, then the depth of neutral axis for a single reinforced rectangular balanced section will bea)0.45db)0.30dc)0.40dd)0.35dCorrect answer is option 'C'. Can you explain this answer? covers all topics & solutions for Civil Engineering (CE) 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for If the permissible stress in steel in tension is 140 N / mm2, then the depth of neutral axis for a single reinforced rectangular balanced section will bea)0.45db)0.30dc)0.40dd)0.35dCorrect answer is option 'C'. Can you explain this answer?.
If the permissible stress in steel in tension is 140 N / mm2, then the depth of neutral axis for a single reinforced rectangular balanced section will bea)0.45db)0.30dc)0.40dd)0.35dCorrect answer is option 'C'. Can you explain this answer? for Civil Engineering (CE) 2024 is part of Civil Engineering (CE) preparation. The Question and answers have been prepared according to the Civil Engineering (CE) exam syllabus. Information about If the permissible stress in steel in tension is 140 N / mm2, then the depth of neutral axis for a single reinforced rectangular balanced section will bea)0.45db)0.30dc)0.40dd)0.35dCorrect answer is option 'C'. Can you explain this answer? covers all topics & solutions for Civil Engineering (CE) 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for If the permissible stress in steel in tension is 140 N / mm2, then the depth of neutral axis for a single reinforced rectangular balanced section will bea)0.45db)0.30dc)0.40dd)0.35dCorrect answer is option 'C'. Can you explain this answer?.
Solutions for If the permissible stress in steel in tension is 140 N / mm2, then the depth of neutral axis for a single reinforced rectangular balanced section will bea)0.45db)0.30dc)0.40dd)0.35dCorrect answer is option 'C'. Can you explain this answer? in English & in Hindi are available as part of our courses for Civil Engineering (CE).
Download more important topics, notes, lectures and mock test series for Civil Engineering (CE) Exam by signing up for free.
Here you can find the meaning of If the permissible stress in steel in tension is 140 N / mm2, then the depth of neutral axis for a single reinforced rectangular balanced section will bea)0.45db)0.30dc)0.40dd)0.35dCorrect answer is option 'C'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
If the permissible stress in steel in tension is 140 N / mm2, then the depth of neutral axis for a single reinforced rectangular balanced section will bea)0.45db)0.30dc)0.40dd)0.35dCorrect answer is option 'C'. Can you explain this answer?, a detailed solution for If the permissible stress in steel in tension is 140 N / mm2, then the depth of neutral axis for a single reinforced rectangular balanced section will bea)0.45db)0.30dc)0.40dd)0.35dCorrect answer is option 'C'. Can you explain this answer? has been provided alongside types of If the permissible stress in steel in tension is 140 N / mm2, then the depth of neutral axis for a single reinforced rectangular balanced section will bea)0.45db)0.30dc)0.40dd)0.35dCorrect answer is option 'C'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice If the permissible stress in steel in tension is 140 N / mm2, then the depth of neutral axis for a single reinforced rectangular balanced section will bea)0.45db)0.30dc)0.40dd)0.35dCorrect answer is option 'C'. Can you explain this answer? tests, examples and also practice Civil Engineering (CE) tests.
|
|
Explore Courses for Civil Engineering (CE) exam
|
|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
|
© EduRev
|
Education Revolution
|
Follow Us
|
Signup to see your scores
go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup