Mechanical Engineering Exam > Mechanical Engineering Questions > Which of the following is CORRECT for indeter...
Start Learning for Free
Which of the following is CORRECT for indeterminate beam condition?
- a)Number of unknown components should be equal to the number of equilibrium equations
- b)Number of unknown components should be less than the number of equilibrium equations
- c)Number of unknown components should be greater than the number of equilibrium equations
- d)Number of unknown components should be zero
Correct answer is option 'C'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Most Upvoted Answer
Which of the following is CORRECT for indeterminate beam condition?a)N...
A beam is said to be in static equilibrium when the beam is initially at rest and remains at rest when subjected to a system of forces and couples.
Generally, there are 3 equilibrium equations for a planer structure.
The conditions of zero resultant force and zero resultant couple can be expressed as:
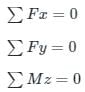
where Fx represents forces along the horizontal or x-axis, Fy represents forces along the vertical or y-axis, and Mz represents the sum of moments taken around any point on the beam.
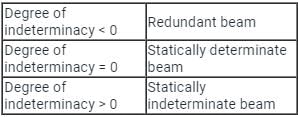
Degree of indeterminacy = Total number of unknown forces - Total number of equilibrium equations
Eg. Statically indeterminate structure
Here, the total number of unknown forces = 7
total number of equilibrium equations = 3
So, the degree of indeterminacy = 7 - 3 = 4, which is greater than zero so it is a statically indeterminate structure.
Eg. Statically determinate structure
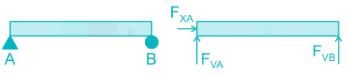
Here, the total number of unknown forces = 3
Total number of equilibrium equations = 3
So, the degree of indeterminacy = 3 - 3 = 0, so it is a statically determinate structure.
Generally, there are 3 equilibrium equations for a planer structure.
The conditions of zero resultant force and zero resultant couple can be expressed as:
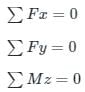
where Fx represents forces along the horizontal or x-axis, Fy represents forces along the vertical or y-axis, and Mz represents the sum of moments taken around any point on the beam.
Degree of indeterminacy = Total number of unknown forces - Total number of equilibrium equations
Eg. Statically indeterminate structure
Here, the total number of unknown forces = 7
total number of equilibrium equations = 3
So, the degree of indeterminacy = 7 - 3 = 4, which is greater than zero so it is a statically indeterminate structure.
Eg. Statically determinate structure
Here, the total number of unknown forces = 3
Total number of equilibrium equations = 3
So, the degree of indeterminacy = 3 - 3 = 0, so it is a statically determinate structure.
Attention Mechanical Engineering Students!
To make sure you are not studying endlessly, EduRev has designed Mechanical Engineering study material, with Structured Courses, Videos, & Test Series. Plus get personalized analysis, doubt solving and improvement plans to achieve a great score in Mechanical Engineering.
|
Explore Courses for Mechanical Engineering exam
|
|
Top Courses for Mechanical EngineeringView all
Which of the following is CORRECT for indeterminate beam condition?a)Number of unknown components should be equal to the number of equilibrium equationsb)Number of unknown components should be less than the number of equilibrium equationsc)Number of unknown components should be greater than the number of equilibrium equationsd)Number of unknown components should be zeroCorrect answer is option 'C'. Can you explain this answer?
Question Description
Which of the following is CORRECT for indeterminate beam condition?a)Number of unknown components should be equal to the number of equilibrium equationsb)Number of unknown components should be less than the number of equilibrium equationsc)Number of unknown components should be greater than the number of equilibrium equationsd)Number of unknown components should be zeroCorrect answer is option 'C'. Can you explain this answer? for Mechanical Engineering 2024 is part of Mechanical Engineering preparation. The Question and answers have been prepared according to the Mechanical Engineering exam syllabus. Information about Which of the following is CORRECT for indeterminate beam condition?a)Number of unknown components should be equal to the number of equilibrium equationsb)Number of unknown components should be less than the number of equilibrium equationsc)Number of unknown components should be greater than the number of equilibrium equationsd)Number of unknown components should be zeroCorrect answer is option 'C'. Can you explain this answer? covers all topics & solutions for Mechanical Engineering 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Which of the following is CORRECT for indeterminate beam condition?a)Number of unknown components should be equal to the number of equilibrium equationsb)Number of unknown components should be less than the number of equilibrium equationsc)Number of unknown components should be greater than the number of equilibrium equationsd)Number of unknown components should be zeroCorrect answer is option 'C'. Can you explain this answer?.
Which of the following is CORRECT for indeterminate beam condition?a)Number of unknown components should be equal to the number of equilibrium equationsb)Number of unknown components should be less than the number of equilibrium equationsc)Number of unknown components should be greater than the number of equilibrium equationsd)Number of unknown components should be zeroCorrect answer is option 'C'. Can you explain this answer? for Mechanical Engineering 2024 is part of Mechanical Engineering preparation. The Question and answers have been prepared according to the Mechanical Engineering exam syllabus. Information about Which of the following is CORRECT for indeterminate beam condition?a)Number of unknown components should be equal to the number of equilibrium equationsb)Number of unknown components should be less than the number of equilibrium equationsc)Number of unknown components should be greater than the number of equilibrium equationsd)Number of unknown components should be zeroCorrect answer is option 'C'. Can you explain this answer? covers all topics & solutions for Mechanical Engineering 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Which of the following is CORRECT for indeterminate beam condition?a)Number of unknown components should be equal to the number of equilibrium equationsb)Number of unknown components should be less than the number of equilibrium equationsc)Number of unknown components should be greater than the number of equilibrium equationsd)Number of unknown components should be zeroCorrect answer is option 'C'. Can you explain this answer?.
Solutions for Which of the following is CORRECT for indeterminate beam condition?a)Number of unknown components should be equal to the number of equilibrium equationsb)Number of unknown components should be less than the number of equilibrium equationsc)Number of unknown components should be greater than the number of equilibrium equationsd)Number of unknown components should be zeroCorrect answer is option 'C'. Can you explain this answer? in English & in Hindi are available as part of our courses for Mechanical Engineering.
Download more important topics, notes, lectures and mock test series for Mechanical Engineering Exam by signing up for free.
Here you can find the meaning of Which of the following is CORRECT for indeterminate beam condition?a)Number of unknown components should be equal to the number of equilibrium equationsb)Number of unknown components should be less than the number of equilibrium equationsc)Number of unknown components should be greater than the number of equilibrium equationsd)Number of unknown components should be zeroCorrect answer is option 'C'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
Which of the following is CORRECT for indeterminate beam condition?a)Number of unknown components should be equal to the number of equilibrium equationsb)Number of unknown components should be less than the number of equilibrium equationsc)Number of unknown components should be greater than the number of equilibrium equationsd)Number of unknown components should be zeroCorrect answer is option 'C'. Can you explain this answer?, a detailed solution for Which of the following is CORRECT for indeterminate beam condition?a)Number of unknown components should be equal to the number of equilibrium equationsb)Number of unknown components should be less than the number of equilibrium equationsc)Number of unknown components should be greater than the number of equilibrium equationsd)Number of unknown components should be zeroCorrect answer is option 'C'. Can you explain this answer? has been provided alongside types of Which of the following is CORRECT for indeterminate beam condition?a)Number of unknown components should be equal to the number of equilibrium equationsb)Number of unknown components should be less than the number of equilibrium equationsc)Number of unknown components should be greater than the number of equilibrium equationsd)Number of unknown components should be zeroCorrect answer is option 'C'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice Which of the following is CORRECT for indeterminate beam condition?a)Number of unknown components should be equal to the number of equilibrium equationsb)Number of unknown components should be less than the number of equilibrium equationsc)Number of unknown components should be greater than the number of equilibrium equationsd)Number of unknown components should be zeroCorrect answer is option 'C'. Can you explain this answer? tests, examples and also practice Mechanical Engineering tests.
|
|
Explore Courses for Mechanical Engineering exam
|
|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
|
© EduRev
|
Education Revolution
|
Follow Us
|
Signup to see your scores
go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup