Why there is a need of Gibbs energy in thermodynamics?

EduRev

All Courses

Explore Courses UPSC Exam UPSC Test Series Free Notes EduRev Infinity

Open App

IIT JAM Exam > IIT JAM Questions > Why there is a need of Gibbs energy in thermo...

Join the Discussion on the App

Why there is a need of Gibbs energy in thermodynamics?

Join for Free

Join for Free

Verified Answer

Why there is a need of Gibbs energy in thermodynamics?

Gibbs Free Energy is the energy available in a system to do work (reversibly, if you want to be nitpicky). That means energy that isn’t dissipated through heat or expansion of the system.

This system, by the way, can be anything you want; your body, a backpack, or a specific chemical reaction. Every system has enthalpy or heat content, represented by H, which you can think of as the energy already in the system. Every system also has statistical entropy, represented by S (I’m not going to explain entropy, because there are plenty of great explanations on Quora already). Simply put, the equation G = H - TS means that the amount of energy available to do work is equal to the heat content minus the probability of energy being dissipated through entropy-related “expansion” (I use the term expansion here very liberally; the system doesn’t have to literally be expanding).

A simple, everyday application is the battery. Batteries operate on the principle of voltage, and voltage is simply a “kind” of Gibbs free energy. Here, you can see, in action, the Gibbs free energy being harnessed to produce work. A battery works by separating a reaction into two half-reactions:

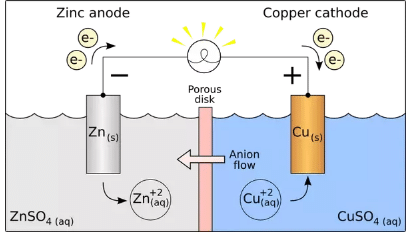
As you can see here, half of this redox reaction occurs at the cathode, while the other half occurs at the anode. This reaction involves energy, some of which we know (by the equation above) goes into rearranging atoms, and expanding the system, but the rest of which can be exploited to power a light-bulb.

The reaction proceeds gradually, and each incremental step of the reaction “releases” Gibbs free energy, which has to travel through a conductor in the form of electricity to complete the circuit. If that still doesn’t help, think of it as this:

This question is part of UPSC exam. View all IIT JAM courses

View all answers Start learning for free

Explore Courses for IIT JAM exam

Why there is a need of Gibbs energy in thermodynamics?

Share with a Friend

Answer this doubt

Question Description
Why there is a need of Gibbs energy in thermodynamics? for IIT JAM 2024 is part of IIT JAM preparation. The Question and answers have been prepared according to the IIT JAM exam syllabus. Information about Why there is a need of Gibbs energy in thermodynamics? covers all topics & solutions for IIT JAM 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Why there is a need of Gibbs energy in thermodynamics?.

Solutions for Why there is a need of Gibbs energy in thermodynamics? in English & in Hindi are available as part of our courses for IIT JAM. Download more important topics, notes, lectures and mock test series for IIT JAM Exam by signing up for free.

Here you can find the meaning of Why there is a need of Gibbs energy in thermodynamics? defined & explained in the simplest way possible. Besides giving the explanation of Why there is a need of Gibbs energy in thermodynamics?, a detailed solution for Why there is a need of Gibbs energy in thermodynamics? has been provided alongside types of Why there is a need of Gibbs energy in thermodynamics? theory, EduRev gives you an ample number of questions to practice Why there is a need of Gibbs energy in thermodynamics? tests, examples and also practice IIT JAM tests.

Explore Courses for IIT JAM exam