Class 11 Exam > Class 11 Questions > 3 identical sphere of equal radius are placed...
Start Learning for Free
3 identical sphere of equal radius are placed on a table in contact. Find the center of mass of spheres.?
Verified Answer
3 identical sphere of equal radius are placed on a table in contact. F...
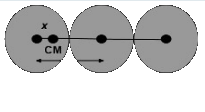
Let the mass of sphere is 'm' and center of mass is at a distance xm away from the center of A.
Now,
X=ΣmiXiΣ/mi =m(x)+m(2R-X)+M(4R-x)/3= m(-x+6R)/3m=-X+X/3=2R
X=1.5R
SO, the center of mass will be at a point 1.5 times of radius distant from the center of sphere A.
 This question is part of UPSC exam. View all Class 11 courses
This question is part of UPSC exam. View all Class 11 courses
Most Upvoted Answer
3 identical sphere of equal radius are placed on a table in contact. F...
Finding the Center of Mass of Identical Spheres in Contact
To find the center of mass of three identical spheres that are in contact with each other, we can follow a systematic approach. Let's break down the solution into several steps:
Step 1: Understanding the Problem
We are given three identical spheres placed on a table in contact with each other. The task is to determine the location of their center of mass.
Step 2: Identifying Key Information
To solve the problem, we need to consider certain key points:
1. The spheres are identical, which means they have the same mass and radius.
2. The spheres are in contact, forming a triangular arrangement.
Step 3: Visualizing the Situation
Before proceeding with the calculations, it is helpful to visualize the scenario. Imagine three identical spheres in contact with each other, forming an equilateral triangle. We need to find the center of mass within this triangular arrangement.
Step 4: Symmetry Considerations
Due to the symmetry of the equilateral triangle formed by the spheres, we can conclude that the center of mass of the system will also lie at the center of the triangle.
Step 5: Calculating the Center of Mass
The center of mass of an equilateral triangle is located at the intersection point of its medians. In this case, the medians of the triangle formed by the spheres coincide with the line segments joining the centers of the spheres.
To calculate the center of mass, we can find the midpoint of the line segment connecting any two sphere centers. Since all the spheres are identical, the center of mass will be equidistant from each sphere.
Step 6: Determining the Coordinates
Let's assume the centers of the spheres are A, B, and C. We can assign coordinates to these points as follows:
- Sphere A: (0, 0)
- Sphere B: (2r, 0) (where 'r' is the radius of the spheres)
- Sphere C: (r, √3r)
Using these coordinates, we can find the midpoint of AB and AC to locate the center of mass.
The midpoint of AB is ((0 + 2r)/2, (0 + 0)/2) = (r, 0)
The midpoint of AC is ((0 + r)/2, (0 + √3r)/2) = (r/2, √3r/2)
Hence, the center of mass of the three spheres is located at (r/2, √3r/2) within the equilateral triangle formed by the spheres.
In conclusion, the center of mass of three identical spheres placed on a table in contact with each other is located at (r/2, √3r/2), where 'r' represents the radius of the spheres.
To find the center of mass of three identical spheres that are in contact with each other, we can follow a systematic approach. Let's break down the solution into several steps:
Step 1: Understanding the Problem
We are given three identical spheres placed on a table in contact with each other. The task is to determine the location of their center of mass.
Step 2: Identifying Key Information
To solve the problem, we need to consider certain key points:
1. The spheres are identical, which means they have the same mass and radius.
2. The spheres are in contact, forming a triangular arrangement.
Step 3: Visualizing the Situation
Before proceeding with the calculations, it is helpful to visualize the scenario. Imagine three identical spheres in contact with each other, forming an equilateral triangle. We need to find the center of mass within this triangular arrangement.
Step 4: Symmetry Considerations
Due to the symmetry of the equilateral triangle formed by the spheres, we can conclude that the center of mass of the system will also lie at the center of the triangle.
Step 5: Calculating the Center of Mass
The center of mass of an equilateral triangle is located at the intersection point of its medians. In this case, the medians of the triangle formed by the spheres coincide with the line segments joining the centers of the spheres.
To calculate the center of mass, we can find the midpoint of the line segment connecting any two sphere centers. Since all the spheres are identical, the center of mass will be equidistant from each sphere.
Step 6: Determining the Coordinates
Let's assume the centers of the spheres are A, B, and C. We can assign coordinates to these points as follows:
- Sphere A: (0, 0)
- Sphere B: (2r, 0) (where 'r' is the radius of the spheres)
- Sphere C: (r, √3r)
Using these coordinates, we can find the midpoint of AB and AC to locate the center of mass.
The midpoint of AB is ((0 + 2r)/2, (0 + 0)/2) = (r, 0)
The midpoint of AC is ((0 + r)/2, (0 + √3r)/2) = (r/2, √3r/2)
Hence, the center of mass of the three spheres is located at (r/2, √3r/2) within the equilateral triangle formed by the spheres.
In conclusion, the center of mass of three identical spheres placed on a table in contact with each other is located at (r/2, √3r/2), where 'r' represents the radius of the spheres.
Attention Class 11 Students!
To make sure you are not studying endlessly, EduRev has designed Class 11 study material, with Structured Courses, Videos, & Test Series. Plus get personalized analysis, doubt solving and improvement plans to achieve a great score in Class 11.

|
Explore Courses for Class 11 exam
|

|
3 identical sphere of equal radius are placed on a table in contact. Find the center of mass of spheres.?
Question Description
3 identical sphere of equal radius are placed on a table in contact. Find the center of mass of spheres.? for Class 11 2024 is part of Class 11 preparation. The Question and answers have been prepared according to the Class 11 exam syllabus. Information about 3 identical sphere of equal radius are placed on a table in contact. Find the center of mass of spheres.? covers all topics & solutions for Class 11 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for 3 identical sphere of equal radius are placed on a table in contact. Find the center of mass of spheres.?.
3 identical sphere of equal radius are placed on a table in contact. Find the center of mass of spheres.? for Class 11 2024 is part of Class 11 preparation. The Question and answers have been prepared according to the Class 11 exam syllabus. Information about 3 identical sphere of equal radius are placed on a table in contact. Find the center of mass of spheres.? covers all topics & solutions for Class 11 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for 3 identical sphere of equal radius are placed on a table in contact. Find the center of mass of spheres.?.
Solutions for 3 identical sphere of equal radius are placed on a table in contact. Find the center of mass of spheres.? in English & in Hindi are available as part of our courses for Class 11.
Download more important topics, notes, lectures and mock test series for Class 11 Exam by signing up for free.
Here you can find the meaning of 3 identical sphere of equal radius are placed on a table in contact. Find the center of mass of spheres.? defined & explained in the simplest way possible. Besides giving the explanation of
3 identical sphere of equal radius are placed on a table in contact. Find the center of mass of spheres.?, a detailed solution for 3 identical sphere of equal radius are placed on a table in contact. Find the center of mass of spheres.? has been provided alongside types of 3 identical sphere of equal radius are placed on a table in contact. Find the center of mass of spheres.? theory, EduRev gives you an
ample number of questions to practice 3 identical sphere of equal radius are placed on a table in contact. Find the center of mass of spheres.? tests, examples and also practice Class 11 tests.

|
Explore Courses for Class 11 exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.























