Class 5 Exam > Class 5 Questions > A ball is dropped from top of a tower of 100m...
Start Learning for Free
A ball is dropped from top of a tower of 100m height. Simultaneously another ball was thrown upward from bottom of the tower with a speed of 50 m/s (g=10 m/s2). They will cross each other after
- a)1s
- b)2s
- c)3s
- d)4s
Correct answer is option 'B'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
A ball is dropped from top of a tower of 100m height. Simultaneously a...
The time taken by both balls at point P has same time ' t ' seconds .
⇒ for first ball , that is in the diagram from A to P
S = ut + 1/2 at^2
we know that u = 0 and a = g
⇒ ( 100 - x ) = (0)t + 1/2 gt^2------------(1)
now, for the second ball from B to P in the diagram ,
S = t + 1/2 at^2
where u = 50 m/s and a = -g
therefore, x = 50(t) - 1/2gt^2 ------------------(2)
by adding equation (1) and (2) we will get ,
100 = 50 t
implies, t = 100 / 50
therefore t = 2 sec .
therefore the time that they cross each other is 2 seconds.
The image that i had attached will show the pictorial representation of the experiment .
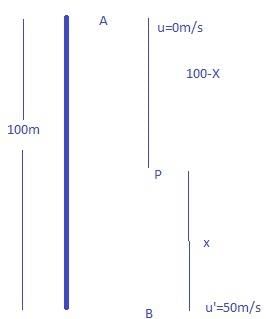
The image that i had attached will show the pictorial representation of the experiment .
Most Upvoted Answer
A ball is dropped from top of a tower of 100m height. Simultaneously a...
Introduction:
In this scenario, we have two balls - one is dropped from the top of a tower and the other is thrown upward from the bottom of the tower. We need to determine when they will cross each other.
Given information:
- Height of the tower = 100m
- Initial speed of the upward thrown ball = 50m/s
- Acceleration due to gravity (g) = 10m/s²
Analysis:
To solve this problem, we can analyze the motion of the two balls separately and determine the time at which their paths intersect.
Ball dropped from the top:
Let's consider the ball dropped from the top of the tower. The initial velocity of this ball is zero because it is simply dropped. We can use the equation of motion to calculate the time it takes for this ball to reach the ground.
Using the equation:
h = ut + (1/2)gt²
Where:
h = height (100m)
u = initial velocity (0m/s)
g = acceleration due to gravity (10m/s²)
t = time
Plugging in the values, we get:
100 = 0 + (1/2)(10)(t²)
100 = 5t²
t² = 20
t = √20 ≈ 4.47s
Ball thrown upward from the bottom:
Now let's consider the ball thrown upward from the bottom of the tower. We need to find the time it takes for this ball to reach the same height as the dropped ball.
Using the equation:
v = u + gt
Where:
v = final velocity (0m/s at the highest point)
u = initial velocity (50m/s)
g = acceleration due to gravity (10m/s²)
t = time
Plugging in the values, we get:
0 = 50 - 10t
10t = 50
t = 5s
Crossing point:
Since the ball thrown upward takes 5 seconds to reach the same height as the dropped ball, and the dropped ball takes 4.47 seconds to reach the ground, the two balls will cross each other between these two time intervals.
Therefore, the correct answer is option 'B', which represents 2 seconds as the approximate time at which they will cross each other.
In this scenario, we have two balls - one is dropped from the top of a tower and the other is thrown upward from the bottom of the tower. We need to determine when they will cross each other.
Given information:
- Height of the tower = 100m
- Initial speed of the upward thrown ball = 50m/s
- Acceleration due to gravity (g) = 10m/s²
Analysis:
To solve this problem, we can analyze the motion of the two balls separately and determine the time at which their paths intersect.
Ball dropped from the top:
Let's consider the ball dropped from the top of the tower. The initial velocity of this ball is zero because it is simply dropped. We can use the equation of motion to calculate the time it takes for this ball to reach the ground.
Using the equation:
h = ut + (1/2)gt²
Where:
h = height (100m)
u = initial velocity (0m/s)
g = acceleration due to gravity (10m/s²)
t = time
Plugging in the values, we get:
100 = 0 + (1/2)(10)(t²)
100 = 5t²
t² = 20
t = √20 ≈ 4.47s
Ball thrown upward from the bottom:
Now let's consider the ball thrown upward from the bottom of the tower. We need to find the time it takes for this ball to reach the same height as the dropped ball.
Using the equation:
v = u + gt
Where:
v = final velocity (0m/s at the highest point)
u = initial velocity (50m/s)
g = acceleration due to gravity (10m/s²)
t = time
Plugging in the values, we get:
0 = 50 - 10t
10t = 50
t = 5s
Crossing point:
Since the ball thrown upward takes 5 seconds to reach the same height as the dropped ball, and the dropped ball takes 4.47 seconds to reach the ground, the two balls will cross each other between these two time intervals.
Therefore, the correct answer is option 'B', which represents 2 seconds as the approximate time at which they will cross each other.
Attention Class 5 Students!
To make sure you are not studying endlessly, EduRev has designed Class 5 study material, with Structured Courses, Videos, & Test Series. Plus get personalized analysis, doubt solving and improvement plans to achieve a great score in Class 5.
|
Explore Courses for Class 5 exam
|
|
Similar Class 5 Doubts
Top Courses for Class 5View all
A ball is dropped from top of a tower of 100m height. Simultaneously another ball was thrown upward from bottom of the tower with a speed of 50 m/s (g=10 m/s2). They will cross each other aftera)1sb)2sc)3sd)4sCorrect answer is option 'B'. Can you explain this answer?
Question Description
A ball is dropped from top of a tower of 100m height. Simultaneously another ball was thrown upward from bottom of the tower with a speed of 50 m/s (g=10 m/s2). They will cross each other aftera)1sb)2sc)3sd)4sCorrect answer is option 'B'. Can you explain this answer? for Class 5 2024 is part of Class 5 preparation. The Question and answers have been prepared according to the Class 5 exam syllabus. Information about A ball is dropped from top of a tower of 100m height. Simultaneously another ball was thrown upward from bottom of the tower with a speed of 50 m/s (g=10 m/s2). They will cross each other aftera)1sb)2sc)3sd)4sCorrect answer is option 'B'. Can you explain this answer? covers all topics & solutions for Class 5 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A ball is dropped from top of a tower of 100m height. Simultaneously another ball was thrown upward from bottom of the tower with a speed of 50 m/s (g=10 m/s2). They will cross each other aftera)1sb)2sc)3sd)4sCorrect answer is option 'B'. Can you explain this answer?.
A ball is dropped from top of a tower of 100m height. Simultaneously another ball was thrown upward from bottom of the tower with a speed of 50 m/s (g=10 m/s2). They will cross each other aftera)1sb)2sc)3sd)4sCorrect answer is option 'B'. Can you explain this answer? for Class 5 2024 is part of Class 5 preparation. The Question and answers have been prepared according to the Class 5 exam syllabus. Information about A ball is dropped from top of a tower of 100m height. Simultaneously another ball was thrown upward from bottom of the tower with a speed of 50 m/s (g=10 m/s2). They will cross each other aftera)1sb)2sc)3sd)4sCorrect answer is option 'B'. Can you explain this answer? covers all topics & solutions for Class 5 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A ball is dropped from top of a tower of 100m height. Simultaneously another ball was thrown upward from bottom of the tower with a speed of 50 m/s (g=10 m/s2). They will cross each other aftera)1sb)2sc)3sd)4sCorrect answer is option 'B'. Can you explain this answer?.
Solutions for A ball is dropped from top of a tower of 100m height. Simultaneously another ball was thrown upward from bottom of the tower with a speed of 50 m/s (g=10 m/s2). They will cross each other aftera)1sb)2sc)3sd)4sCorrect answer is option 'B'. Can you explain this answer? in English & in Hindi are available as part of our courses for Class 5.
Download more important topics, notes, lectures and mock test series for Class 5 Exam by signing up for free.
Here you can find the meaning of A ball is dropped from top of a tower of 100m height. Simultaneously another ball was thrown upward from bottom of the tower with a speed of 50 m/s (g=10 m/s2). They will cross each other aftera)1sb)2sc)3sd)4sCorrect answer is option 'B'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
A ball is dropped from top of a tower of 100m height. Simultaneously another ball was thrown upward from bottom of the tower with a speed of 50 m/s (g=10 m/s2). They will cross each other aftera)1sb)2sc)3sd)4sCorrect answer is option 'B'. Can you explain this answer?, a detailed solution for A ball is dropped from top of a tower of 100m height. Simultaneously another ball was thrown upward from bottom of the tower with a speed of 50 m/s (g=10 m/s2). They will cross each other aftera)1sb)2sc)3sd)4sCorrect answer is option 'B'. Can you explain this answer? has been provided alongside types of A ball is dropped from top of a tower of 100m height. Simultaneously another ball was thrown upward from bottom of the tower with a speed of 50 m/s (g=10 m/s2). They will cross each other aftera)1sb)2sc)3sd)4sCorrect answer is option 'B'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice A ball is dropped from top of a tower of 100m height. Simultaneously another ball was thrown upward from bottom of the tower with a speed of 50 m/s (g=10 m/s2). They will cross each other aftera)1sb)2sc)3sd)4sCorrect answer is option 'B'. Can you explain this answer? tests, examples and also practice Class 5 tests.
|
|
Explore Courses for Class 5 exam
|
|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
|
© EduRev
|
Education Revolution
|
Follow Us
|
Signup to see your scores
go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup