JEE Exam > JEE Questions > Derive an expression for maximum work in isot...
Start Learning for Free
Derive an expression for maximum work in isothermal reversible expansion of "n" moles of an ideal gas.?
Most Upvoted Answer
Derive an expression for maximum work in isothermal reversible expansi...
Let us consider n moles of ideal gas enclosed in a cylinder fitted with a weightless and frictionless piston. The work of expansion for a small change of volume dV against the external pressure P is given by

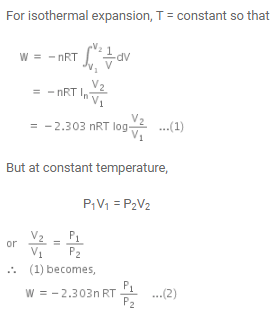

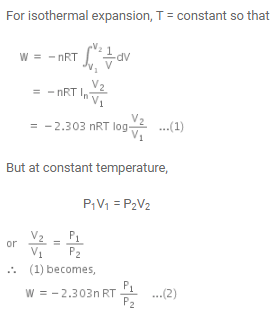
Community Answer
Derive an expression for maximum work in isothermal reversible expansi...
Introduction:
In an isothermal reversible expansion, a gas expands and does work while maintaining a constant temperature. The work done by the gas depends on the initial and final volumes of the gas, and can be calculated using the ideal gas law and the first law of thermodynamics.
Derivation:
To derive the expression for maximum work in an isothermal reversible expansion, we can use the following steps:
1. First Law of Thermodynamics:
The first law of thermodynamics states that the change in internal energy (ΔU) of a system is equal to the heat added to the system (Q) minus the work done by the system (W):
ΔU = Q - W
2. Ideal Gas Law:
For an ideal gas, the internal energy change is related to the temperature change (ΔT) by the equation:
ΔU = nCvΔT
where n is the number of moles of gas and Cv is the molar heat capacity at constant volume.
3. Isothermal Process:
In an isothermal process, the temperature of the gas remains constant, so ΔT = 0. Therefore, the change in internal energy is zero:
ΔU = nCvΔT = 0
4. Work done by the gas:
Since ΔU = 0, we can rewrite the first law of thermodynamics equation as:
0 = Q - W
W = Q
5. Isothermal Expansion:
During an isothermal expansion, the gas does work by pushing against a constant external pressure (Pext). The work done by the gas can be calculated using the equation:
W = ∫PextdV
where Pext is the constant external pressure and dV is the differential change in volume.
6. Reversible Expansion:
In a reversible expansion, the external pressure is infinitesimally close to the internal pressure of the gas at each step. Therefore, the external pressure can be considered equal to the internal pressure (Pint) of the gas.
7. Gas Law:
Using the ideal gas law, the internal pressure of the gas can be expressed as:
Pint = nRT/V
where R is the ideal gas constant and V is the volume of the gas.
8. Substituting values:
Substituting the expression for internal pressure (Pint) into the work equation, we get:
W = ∫(nRT/V)dV
9. Integrating:
Integrating the above equation, we obtain the expression for work done in an isothermal reversible expansion:
W = nRT ln(Vf/Vi)
Conclusion:
The expression for maximum work in an isothermal reversible expansion of "n" moles of an ideal gas is given by W = nRT ln(Vf/Vi), where n is the number of moles, R is the ideal gas constant, T is the temperature, Vi is the initial volume, and Vf is the final volume of the gas. This expression can be derived using the first law of thermodynamics, the ideal gas law, and the principles of isothermal and reversible processes.
In an isothermal reversible expansion, a gas expands and does work while maintaining a constant temperature. The work done by the gas depends on the initial and final volumes of the gas, and can be calculated using the ideal gas law and the first law of thermodynamics.
Derivation:
To derive the expression for maximum work in an isothermal reversible expansion, we can use the following steps:
1. First Law of Thermodynamics:
The first law of thermodynamics states that the change in internal energy (ΔU) of a system is equal to the heat added to the system (Q) minus the work done by the system (W):
ΔU = Q - W
2. Ideal Gas Law:
For an ideal gas, the internal energy change is related to the temperature change (ΔT) by the equation:
ΔU = nCvΔT
where n is the number of moles of gas and Cv is the molar heat capacity at constant volume.
3. Isothermal Process:
In an isothermal process, the temperature of the gas remains constant, so ΔT = 0. Therefore, the change in internal energy is zero:
ΔU = nCvΔT = 0
4. Work done by the gas:
Since ΔU = 0, we can rewrite the first law of thermodynamics equation as:
0 = Q - W
W = Q
5. Isothermal Expansion:
During an isothermal expansion, the gas does work by pushing against a constant external pressure (Pext). The work done by the gas can be calculated using the equation:
W = ∫PextdV
where Pext is the constant external pressure and dV is the differential change in volume.
6. Reversible Expansion:
In a reversible expansion, the external pressure is infinitesimally close to the internal pressure of the gas at each step. Therefore, the external pressure can be considered equal to the internal pressure (Pint) of the gas.
7. Gas Law:
Using the ideal gas law, the internal pressure of the gas can be expressed as:
Pint = nRT/V
where R is the ideal gas constant and V is the volume of the gas.
8. Substituting values:
Substituting the expression for internal pressure (Pint) into the work equation, we get:
W = ∫(nRT/V)dV
9. Integrating:
Integrating the above equation, we obtain the expression for work done in an isothermal reversible expansion:
W = nRT ln(Vf/Vi)
Conclusion:
The expression for maximum work in an isothermal reversible expansion of "n" moles of an ideal gas is given by W = nRT ln(Vf/Vi), where n is the number of moles, R is the ideal gas constant, T is the temperature, Vi is the initial volume, and Vf is the final volume of the gas. This expression can be derived using the first law of thermodynamics, the ideal gas law, and the principles of isothermal and reversible processes.

|
Explore Courses for JEE exam
|

|
Similar JEE Doubts
Derive an expression for maximum work in isothermal reversible expansion of "n" moles of an ideal gas.?
Question Description
Derive an expression for maximum work in isothermal reversible expansion of "n" moles of an ideal gas.? for JEE 2025 is part of JEE preparation. The Question and answers have been prepared according to the JEE exam syllabus. Information about Derive an expression for maximum work in isothermal reversible expansion of "n" moles of an ideal gas.? covers all topics & solutions for JEE 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Derive an expression for maximum work in isothermal reversible expansion of "n" moles of an ideal gas.?.
Derive an expression for maximum work in isothermal reversible expansion of "n" moles of an ideal gas.? for JEE 2025 is part of JEE preparation. The Question and answers have been prepared according to the JEE exam syllabus. Information about Derive an expression for maximum work in isothermal reversible expansion of "n" moles of an ideal gas.? covers all topics & solutions for JEE 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Derive an expression for maximum work in isothermal reversible expansion of "n" moles of an ideal gas.?.
Solutions for Derive an expression for maximum work in isothermal reversible expansion of "n" moles of an ideal gas.? in English & in Hindi are available as part of our courses for JEE.
Download more important topics, notes, lectures and mock test series for JEE Exam by signing up for free.
Here you can find the meaning of Derive an expression for maximum work in isothermal reversible expansion of "n" moles of an ideal gas.? defined & explained in the simplest way possible. Besides giving the explanation of
Derive an expression for maximum work in isothermal reversible expansion of "n" moles of an ideal gas.?, a detailed solution for Derive an expression for maximum work in isothermal reversible expansion of "n" moles of an ideal gas.? has been provided alongside types of Derive an expression for maximum work in isothermal reversible expansion of "n" moles of an ideal gas.? theory, EduRev gives you an
ample number of questions to practice Derive an expression for maximum work in isothermal reversible expansion of "n" moles of an ideal gas.? tests, examples and also practice JEE tests.

|
Explore Courses for JEE exam
|

|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
























