Chemistry Exam > Chemistry Questions > Hydrogen peroxide in aqueous solution decompo...
Start Learning for Free
Hydrogen peroxide in aqueous solution decomposes on warming to give oxygen acc. To the equation , 2H2O2 (aq.) → 2H2O (l) + O2 (g) . Under conditions where 1 mole of gas occupies 24dm3. 100cm3 of xM solution of H2O2 produces 3dm3 of O2. The x is:
- a)2.5
- b)1
- c)0.5
- d)0.25
Correct answer is option 'A'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
Hydrogen peroxide in aqueous solution decomposes on warming to give ox...
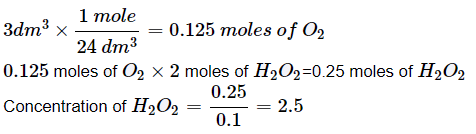
Most Upvoted Answer
Hydrogen peroxide in aqueous solution decomposes on warming to give ox...
Decomposition of Hydrogen Peroxide
Hydrogen peroxide (H2O2) in aqueous solution decomposes on warming to give oxygen according to the equation: 2H2O2 (aq.) 2H2O (l) O2 (g).
Volume of Oxygen Produced
Under conditions where 1 mole of gas occupies 24 dm3, 100 cm3 of x M solution of H2O2 produces 3 dm3 of O2.
Determination of x
To determine the value of x, we need to use the following formula:
n = V/Vm
where n is the number of moles, V is the volume of gas produced, and Vm is the molar volume of gas at the given temperature and pressure (24 dm3/mol).
From the given data, we have:
n = 3/24 = 0.125 mol
We know that the balanced equation for the decomposition of hydrogen peroxide is:
2H2O2 (aq.) 2H2O (l) O2 (g)
Therefore, for every 2 moles of H2O2 that decompose, 1 mole of O2 is produced. Therefore, the number of moles of H2O2 that decompose to produce 0.125 mol of O2 is:
n(H2O2) = (2/1) x 0.125 = 0.25 mol
Finally, we can calculate the concentration of the H2O2 solution as follows:
x = n/V
where n is the number of moles of H2O2 and V is the volume of the solution in dm3.
From the given data, we have:
V = 100/1000 = 0.1 dm3
x = 0.25/0.1 = 2.5 M
Therefore, the correct answer is option A (2.5).
Hydrogen peroxide (H2O2) in aqueous solution decomposes on warming to give oxygen according to the equation: 2H2O2 (aq.) 2H2O (l) O2 (g).
Volume of Oxygen Produced
Under conditions where 1 mole of gas occupies 24 dm3, 100 cm3 of x M solution of H2O2 produces 3 dm3 of O2.
Determination of x
To determine the value of x, we need to use the following formula:
n = V/Vm
where n is the number of moles, V is the volume of gas produced, and Vm is the molar volume of gas at the given temperature and pressure (24 dm3/mol).
From the given data, we have:
n = 3/24 = 0.125 mol
We know that the balanced equation for the decomposition of hydrogen peroxide is:
2H2O2 (aq.) 2H2O (l) O2 (g)
Therefore, for every 2 moles of H2O2 that decompose, 1 mole of O2 is produced. Therefore, the number of moles of H2O2 that decompose to produce 0.125 mol of O2 is:
n(H2O2) = (2/1) x 0.125 = 0.25 mol
Finally, we can calculate the concentration of the H2O2 solution as follows:
x = n/V
where n is the number of moles of H2O2 and V is the volume of the solution in dm3.
From the given data, we have:
V = 100/1000 = 0.1 dm3
x = 0.25/0.1 = 2.5 M
Therefore, the correct answer is option A (2.5).
Free Test
FREE
| Start Free Test |
Community Answer
Hydrogen peroxide in aqueous solution decomposes on warming to give ox...
3L of O2 is produced, no. of moles = 3/24 = 0.125mol
2 mol H2O2 react to produce 1 mol O2, therefore there must be 0.125*2 = 0.250mol of H2O2
Now, molarity = n/V
V = 100mL = 0.1L
Therefore, x = 0.25/0.1 = 2.5 M (option A)
2 mol H2O2 react to produce 1 mol O2, therefore there must be 0.125*2 = 0.250mol of H2O2
Now, molarity = n/V
V = 100mL = 0.1L
Therefore, x = 0.25/0.1 = 2.5 M (option A)
|
Explore Courses for Chemistry exam
|
|
Similar Chemistry Doubts
Hydrogen peroxide in aqueous solution decomposes on warming to give oxygen acc. To the equation , 2H2O2 (aq.) → 2H2O (l) + O2 (g) . Under conditions where 1 mole of gas occupies 24dm3. 100cm3 of xM solution of H2O2 produces 3dm3 of O2. The x is:a)2.5b)1c)0.5d)0.25Correct answer is option 'A'. Can you explain this answer?
Question Description
Hydrogen peroxide in aqueous solution decomposes on warming to give oxygen acc. To the equation , 2H2O2 (aq.) → 2H2O (l) + O2 (g) . Under conditions where 1 mole of gas occupies 24dm3. 100cm3 of xM solution of H2O2 produces 3dm3 of O2. The x is:a)2.5b)1c)0.5d)0.25Correct answer is option 'A'. Can you explain this answer? for Chemistry 2024 is part of Chemistry preparation. The Question and answers have been prepared according to the Chemistry exam syllabus. Information about Hydrogen peroxide in aqueous solution decomposes on warming to give oxygen acc. To the equation , 2H2O2 (aq.) → 2H2O (l) + O2 (g) . Under conditions where 1 mole of gas occupies 24dm3. 100cm3 of xM solution of H2O2 produces 3dm3 of O2. The x is:a)2.5b)1c)0.5d)0.25Correct answer is option 'A'. Can you explain this answer? covers all topics & solutions for Chemistry 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Hydrogen peroxide in aqueous solution decomposes on warming to give oxygen acc. To the equation , 2H2O2 (aq.) → 2H2O (l) + O2 (g) . Under conditions where 1 mole of gas occupies 24dm3. 100cm3 of xM solution of H2O2 produces 3dm3 of O2. The x is:a)2.5b)1c)0.5d)0.25Correct answer is option 'A'. Can you explain this answer?.
Hydrogen peroxide in aqueous solution decomposes on warming to give oxygen acc. To the equation , 2H2O2 (aq.) → 2H2O (l) + O2 (g) . Under conditions where 1 mole of gas occupies 24dm3. 100cm3 of xM solution of H2O2 produces 3dm3 of O2. The x is:a)2.5b)1c)0.5d)0.25Correct answer is option 'A'. Can you explain this answer? for Chemistry 2024 is part of Chemistry preparation. The Question and answers have been prepared according to the Chemistry exam syllabus. Information about Hydrogen peroxide in aqueous solution decomposes on warming to give oxygen acc. To the equation , 2H2O2 (aq.) → 2H2O (l) + O2 (g) . Under conditions where 1 mole of gas occupies 24dm3. 100cm3 of xM solution of H2O2 produces 3dm3 of O2. The x is:a)2.5b)1c)0.5d)0.25Correct answer is option 'A'. Can you explain this answer? covers all topics & solutions for Chemistry 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Hydrogen peroxide in aqueous solution decomposes on warming to give oxygen acc. To the equation , 2H2O2 (aq.) → 2H2O (l) + O2 (g) . Under conditions where 1 mole of gas occupies 24dm3. 100cm3 of xM solution of H2O2 produces 3dm3 of O2. The x is:a)2.5b)1c)0.5d)0.25Correct answer is option 'A'. Can you explain this answer?.
Solutions for Hydrogen peroxide in aqueous solution decomposes on warming to give oxygen acc. To the equation , 2H2O2 (aq.) → 2H2O (l) + O2 (g) . Under conditions where 1 mole of gas occupies 24dm3. 100cm3 of xM solution of H2O2 produces 3dm3 of O2. The x is:a)2.5b)1c)0.5d)0.25Correct answer is option 'A'. Can you explain this answer? in English & in Hindi are available as part of our courses for Chemistry.
Download more important topics, notes, lectures and mock test series for Chemistry Exam by signing up for free.
Here you can find the meaning of Hydrogen peroxide in aqueous solution decomposes on warming to give oxygen acc. To the equation , 2H2O2 (aq.) → 2H2O (l) + O2 (g) . Under conditions where 1 mole of gas occupies 24dm3. 100cm3 of xM solution of H2O2 produces 3dm3 of O2. The x is:a)2.5b)1c)0.5d)0.25Correct answer is option 'A'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
Hydrogen peroxide in aqueous solution decomposes on warming to give oxygen acc. To the equation , 2H2O2 (aq.) → 2H2O (l) + O2 (g) . Under conditions where 1 mole of gas occupies 24dm3. 100cm3 of xM solution of H2O2 produces 3dm3 of O2. The x is:a)2.5b)1c)0.5d)0.25Correct answer is option 'A'. Can you explain this answer?, a detailed solution for Hydrogen peroxide in aqueous solution decomposes on warming to give oxygen acc. To the equation , 2H2O2 (aq.) → 2H2O (l) + O2 (g) . Under conditions where 1 mole of gas occupies 24dm3. 100cm3 of xM solution of H2O2 produces 3dm3 of O2. The x is:a)2.5b)1c)0.5d)0.25Correct answer is option 'A'. Can you explain this answer? has been provided alongside types of Hydrogen peroxide in aqueous solution decomposes on warming to give oxygen acc. To the equation , 2H2O2 (aq.) → 2H2O (l) + O2 (g) . Under conditions where 1 mole of gas occupies 24dm3. 100cm3 of xM solution of H2O2 produces 3dm3 of O2. The x is:a)2.5b)1c)0.5d)0.25Correct answer is option 'A'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice Hydrogen peroxide in aqueous solution decomposes on warming to give oxygen acc. To the equation , 2H2O2 (aq.) → 2H2O (l) + O2 (g) . Under conditions where 1 mole of gas occupies 24dm3. 100cm3 of xM solution of H2O2 produces 3dm3 of O2. The x is:a)2.5b)1c)0.5d)0.25Correct answer is option 'A'. Can you explain this answer? tests, examples and also practice Chemistry tests.
|
|
Explore Courses for Chemistry exam
|
|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
|
© EduRev
|
Education Revolution
|
Follow Us
|
Signup to see your scores
go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup