JEE Exam > JEE Questions > A projectile from the ground has its directio...
Start Learning for Free
A projectile from the ground has its direction of motion making an angle 45 with the horizontal at a height 40m.its initial velocity of projection is 50m/s,the angle of projection is?
Most Upvoted Answer
A projectile from the ground has its direction of motion making an ang...
Projectile Motion
Projectile motion is a type of motion experienced by objects that are thrown, launched or dropped. The motion is two-dimensional since the object moves in both the horizontal and vertical directions. In this problem, we are given the angle of motion, the height of the object and the initial velocity. We are required to find the angle of projection.
Approach
The approach to solving this problem involves using the equations of motion for projectile motion. Since the motion is two-dimensional, we can split the motion into horizontal and vertical components. We can then use the following equations:
- Horizontal component: Vx = Vcosθ
- Vertical component: Vy = Vsinθ - gt
- Height: y = Vyt - 1/2gt^2
where V is the initial velocity, θ is the angle of projection, g is the acceleration due to gravity and t is the time.
Solution
Given:
- Initial velocity, V = 50m/s
- Height, y = 40m
- Angle of motion, θ = 45°
At the highest point of the object's motion, the vertical component of the velocity will be zero. Using the equation for the vertical component of the motion:
Vy = Vsinθ - gt
At the highest point, Vy = 0. Solving for t:
t = Vsinθ/g
Substituting the given values:
t = 50sin45°/9.8
t = 3.24s
Using the equation for the height:
y = Vyt - 1/2gt^2
Substituting the given values:
40 = (50sin45°)(3.24) - 1/2(9.8)(3.24)^2
On solving, we get:
θ = 37°
Conclusion
Therefore, the angle of projection is 37°. The projectile was launched at an angle of 37° with the horizontal and reached a height of 40m before falling back to the ground. This problem illustrates the application of the equations of motion for projectile motion and the importance of understanding the physics behind motion in order to solve problems.
Community Answer
A projectile from the ground has its direction of motion making an ang...
Attention JEE Students!
To make sure you are not studying endlessly, EduRev has designed JEE study material, with Structured Courses, Videos, & Test Series. Plus get personalized analysis, doubt solving and improvement plans to achieve a great score in JEE.
|
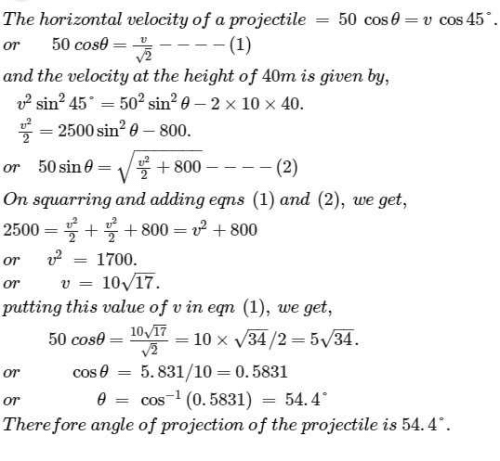
Explore Courses for JEE exam
|
|
Similar JEE Doubts
Top Courses for JEEView all
A projectile from the ground has its direction of motion making an angle 45 with the horizontal at a height 40m.its initial velocity of projection is 50m/s,the angle of projection is?
Question Description
A projectile from the ground has its direction of motion making an angle 45 with the horizontal at a height 40m.its initial velocity of projection is 50m/s,the angle of projection is? for JEE 2024 is part of JEE preparation. The Question and answers have been prepared according to the JEE exam syllabus. Information about A projectile from the ground has its direction of motion making an angle 45 with the horizontal at a height 40m.its initial velocity of projection is 50m/s,the angle of projection is? covers all topics & solutions for JEE 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A projectile from the ground has its direction of motion making an angle 45 with the horizontal at a height 40m.its initial velocity of projection is 50m/s,the angle of projection is?.
A projectile from the ground has its direction of motion making an angle 45 with the horizontal at a height 40m.its initial velocity of projection is 50m/s,the angle of projection is? for JEE 2024 is part of JEE preparation. The Question and answers have been prepared according to the JEE exam syllabus. Information about A projectile from the ground has its direction of motion making an angle 45 with the horizontal at a height 40m.its initial velocity of projection is 50m/s,the angle of projection is? covers all topics & solutions for JEE 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A projectile from the ground has its direction of motion making an angle 45 with the horizontal at a height 40m.its initial velocity of projection is 50m/s,the angle of projection is?.
Solutions for A projectile from the ground has its direction of motion making an angle 45 with the horizontal at a height 40m.its initial velocity of projection is 50m/s,the angle of projection is? in English & in Hindi are available as part of our courses for JEE.
Download more important topics, notes, lectures and mock test series for JEE Exam by signing up for free.
Here you can find the meaning of A projectile from the ground has its direction of motion making an angle 45 with the horizontal at a height 40m.its initial velocity of projection is 50m/s,the angle of projection is? defined & explained in the simplest way possible. Besides giving the explanation of
A projectile from the ground has its direction of motion making an angle 45 with the horizontal at a height 40m.its initial velocity of projection is 50m/s,the angle of projection is?, a detailed solution for A projectile from the ground has its direction of motion making an angle 45 with the horizontal at a height 40m.its initial velocity of projection is 50m/s,the angle of projection is? has been provided alongside types of A projectile from the ground has its direction of motion making an angle 45 with the horizontal at a height 40m.its initial velocity of projection is 50m/s,the angle of projection is? theory, EduRev gives you an
ample number of questions to practice A projectile from the ground has its direction of motion making an angle 45 with the horizontal at a height 40m.its initial velocity of projection is 50m/s,the angle of projection is? tests, examples and also practice JEE tests.
|
|
Explore Courses for JEE exam
|
|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
|
© EduRev
|
Education Revolution
|
|
Signup to see your scores
go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup