Assignment - Polynomials, Class 9 Mathematics PDF Download
TRUE AND FALSE TYPE QUESTIONS
1. When 14x3 – 3x2 + 4x + 2 is divided by 2x – 1, the remainder is 5.
2. 4x2 – 8x + 15 is exactly divisible by 2x – 1.
3. x2 – 5x + 6 cannot be written as a product of two linear factors.
4. x3 – 3x2y + 3xy2 – y3 is exactly divisible by x – y.
5. (2a + b)2 – (2b + a)2 = 3(a2 – b2) is an identify.
6. (x2 + 1)4 = (x4 – 6x2 + 1)2 + 16 (x3 – x)2 in an identity.
FILL IN THE BLANKS
1. If a + b = 2, then the value of a3 + b3 + 6ab is equal to .......................
2. The coefficient of 'a' in (a + b + c) (x + y + z) + (b + c – a) (x + y – z) + (c + a – b) (y + z – x) is .......................
3. (x + y)2 + (x – y)2 ÷ 
reduces to .......................
4. The expression 
for all values of x. Then A and B are respectively equal to............
5. The coefficient of x2 in (px2 + 4x + r) × (4x2 – 3qx – 5) is .......................
6. The value of {x + (y – z)}2 – {y – (z + x)}2 + {z – (x + y)}2 when x = 1, y = –2, z = 3 is equal to .......................
7. 
then the value of m .......................
8. abx2 + (a2 – b2)x – ab when expressed in linear factors is equal to .......................
9. x2 (y – z) + y2(z – x) + z2(x – y) = N(x – y) (y – z), (z – x), then N must have value .......................
10. 4x2 – 2bx + ab – a2 when expressed in linear factors is equal to .......................
VERY SHORT ANSWER TYPE QUESTIONS
1. Which of the following expressions are polynomial or not :
(i) 11x + 1
(ii) 7x2 – 5x + 5
(iii) t3 – 2t + 1
2. Write the coefficient of x3 in each of the following :
(i) 3x3 – 3x + 2 (ii) 14x3 – 2x3 + 5x – 7x2 (iii) √2 x2 + 1 (iv) 3/4x3 + 2x – 3
3. Write the degree of each of the following polynomials :
(i) 3x2 – 4x + 2 (ii) 7x3 + 2x2 + x (iii) 5 – x2 (iv) 1 + 2x + 3x2 – 11x4
4. Classify the following as linear, quadratic and cubic polynomials :
(i) x3 – 4 (ii) x2 + 1 (iii) 5x2 – 3x + 7 (iv) 1 + 5x (v) 4r3
5. Find the value of the following polynomial at the indicate value of variables :
(i) p(x) = 5x2 – 3x + 7 at x = 1
(ii) q(y) = 3y2 – 4y + √2 at y = 2
(iii) p(t) = 4t4 + 5t3 – t2 + 6 at t = a
6. Find the zeroes of each of the following polynomials :
(i) P(x) = x – 4
(ii) g(x) = 2x + 1
(iii) P(x) = (x + 1) (x + 2)
(iv) P(x) = (x –1) (x – 2) (x – 3)
(v) P(x) = 7x2
(vi) P(x) = rx + s, r 
0
7. Verify whether the following are zeroes of the polynomial indicated against them :
(i) P(x) = 5x – 1, x = 15
(ii) P(x) (x – 2) (x – 5), x = 2, 5
(iii) S(x) = x2, x = 0, 1
(iv) P(x) = 3x2 – 1, 
(v) g(x) = 5x2 + 7x, x = 0, –7/5
8. Use remainder theorem to find remainder when P(x) is dividedby q(x) in the following questions :
(i) P(x) = 2x2 – 5x + 7, q(x) = x – 1
(ii) P(x) = x9 – 5x4 + 1, q(x) = x + 1
(iii) P(x) = 4x3 – 12x2 + 11x – 5, q(x) = x – 1
2
(iv) P(x) = x4 + x3 + x2 – 5x + 1 q(x) = x + 1
Use factor theorem to verify in each of the following that q(x) is a factor of p(x)
9. (i) P(x) = 3x2 – 5x + 2, q(x) = 3x – 2
(ii) P(x) = x4 – x3 + x – 1 q(x) = x + 1
(iii) P(x) = x5 – x4 + 3x2 – 2x + 4 q(x) = x – 2
10. Find the value of k if (x – 2) is a factor of 2x3 – 6x2 + 5x + k.
11. Find the value of k if (x + 3) is a factor of 3x2 + kx + 6.
12. For what value of k is y3 + ky + 2k – 2 exactly divisible by (y + 1) ?
13. For what value of m is 2x3 + mx2 + 11x + m + 3 exactly divisible by (2x – 1) ?
14. Find the value of a if (2y + 3) is a factor of 2y3 + 9y2 – y – a.
15. Show that 3 is a zero of the polynomial x3 + x2 – 17x + 15.
16. Show that –1 is a zero of the polynomial 2x3 – x2 + x + 4.
17. Show that 1 is not a zero of the polynomial 4x4 – 3x3 + 2x2 – 5x + 1.
18. Using factor theorem, show that a – b is a factor of a(b2 – c2) + b(c2 – a2) + c(a2 – b2).
19. Using factor theorem, factorize the polynomial x2 – 5x + 6.
20. Find the value of p, if (x + 1) is a factor of polynomial 2x3 – 2x2 + x + p.
21. Find the value of a, if x + 2 is a factor of x3 – 2ax2 + 16.
FACTORIZE EACH OF THE FOLLOWING EXPRESSIONS
22. x2 – x – 42.
23. 1 + 2x + x2.
24. 6 – 5y – y2.
25. x2 – 9ax + 18a2.
26. a2 + 46a + 205.
27. k2 – 26k + 133.
28. ab + ac – b2 – bc.
29. 2xy – x + z – 2zy.
30. 100 – 9p2.
31. p4 – 81q4.
32. 1/5x2 + 2x – 15.
33. 7√2 x2 – 10x – 4√2 .
34. 6xy + 6 – 9y – 4x.
35. 1 – 256x4.
36. x4 – (2y – 3z)2.
37. (2a + 1)2 – 9b4.
38. 24√3 x3 – 125y3.
39. 
40. a6x4 – a4x6.
41. If one of the factors of x2 + x – 20 is (x + 5), find other factor.
42. Find the positive squares root of 36x2 + 60x + 25.
43. Simplify : (i)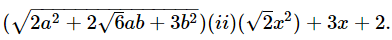 
44. Factorise : 5(a + 2b)2 – 7(a + 2b) + 2.
45. Factorise : 125(x – y)3 + (5y – 3z)3 + (3z – 5x)3.
46. Factorise : 27x3 + y3 + z3 – 9xyz.
47. Find the product : (i) (x + 2) (x + 9) (ii) (x + 8) (x – 2) (iii) (z – 3) (z – 5) (iv) (z2 + 4) (z2 – 5)
48. Evaluate the following : (i) 103 × 105 (ii) 98 × 99 (iii) 104 × 95
49. Write : 
in expand form.
50. Write : 
is expand form.
SHORT ANSWER TYPE QUESTION
51. Write the expansions of each of the following : (i) (9x + 2y + z)2 (ii) (3x – 2y – z)2
52. Simplify : (2a + b + c)2 + (2a – b – c)2
53. Write the expansion of the following : (i) (2x + 3y)3 (ii) (p – yz)3.
54. Find the value of 27x3 + 8y3 if 3x + 2y = 20 and xy = 14/9 .
55. Find the value of a3 – 27b3 if a – 3b = – 6 and ab = – 10.
56. Evaluate : (i) (101)3 (ii) (399)3.
57. Find the product of following :
(i) (x + 3) (x2 – 3x + 9) (ii) (5a – 3b) (25a2 + 15ab + 9b2)
58. Factorize :
(i) 1 – 27z3 (ii) 250x3 – 16y3 (iii) xy3 + 729x4
59. If x + y + z = 8 and xy + yz + zx = 20, find the value of x3 + y3 + z3 – 3xyz.
60. If a + b + c = 9 and a2 + b2 + c2 = 35, find the value of a3 + b3 + c3 – 3abc.
61. If p + q + r = 1 and pq + qr + pr = –1 and pqr = –1, find the value of p3 + q3 + r3.
62. Factorize : (x – y)3 + (y – z)3 + (z – x)3.
63. Find the value of : (25)3 – (29)3 + (4)3.
LONG ANSWER TYPE QUESTION
64. With out actual division, prove that a4 + 2a3 – 2a2 + 2a – 3 is exactly divisible a2 + 2a – 3.
65. If (x + 1) and (x – 1) are the factors of mx3 + x2 – 2x + n, find the value of m and n.
66. The polynomials kx3 + 3x2 – 3 and 2x3 – 5x + k when divided by (x – 4) leave the same remainder or in each case. Find the value of k.
67. What must be added to x3 – 3x2 – 12x + 19 so that result is exactly divisible by x2 + x – 6?
68. What must be subtrated from x3 – 6x2 – 15x + 80 so that result is exactly divisible by x2 + x – 12 ?
69. Using factor theorem, factorise the polynomial x4 – 2x3 – 7x2 + 8x + 12.
70. Let A and B are the remainders when the polynomial y3 + 2y2 – 5ay – 7 and y3 + ay2 – 12y + 6 are divided by y + 1 and y – 2 respectively. If 2A + B = 6, find the value of a.
71. Simplify : (a + b)3 + (a – b)3 + 6a(a2 – b2).
72. Show that if a + b is not zero, then the equation a(x – a) = 2ab – b(x – b) has a solution x = a + b.
73. Show that if 2(a2 + b2) = (a + b)2, then a = b.
74. Find the value of :
(i) x3 + y3 – 12xy + 64 when x + y = – 4
(ii) x3 – 8y3 – 36xy – 216 when x = 2y + 6.
(iii) (x – a)3 + (x – b)3 + (x – c)3 – 3(x – a) (x – b) (x – c) when a + b + c = 3x.
75. Prove that a3 + b3 + c3 – 3abc = 1
2 (a + b + c) [(a – b)2 + (b – c)2 + (c – a)2]
76. Prove that (a + b)3 + (b + c)3 + (c + a)3 – 3(a + b) (b + c) (c + a) = 2(a3 + b3 + c3 – 3abc)
77. If x2 – 1 is a factor of ax4 + bx3 + cx2 + dx + e, show that a + c + e = b + d = 0.
78. Using factor theorem, show that (x + y), (y + z) and (z + x) are the factors of (x + y – z)3 – (x3 + y3 + z3).
79. If (3x – 1)4 = a4x4 + a3x3 + a2x2 + a1x + a0, then find the value of a4 +3a3 + 9a2 + 27a1 + 81a0.
ANSWER KEY
TRUE & FALSE
1. T
2. F
3. F
4. T
5. T
6. T
FILL IN THE BLANKS
1. [8]
2. [–x + y + 3z]
3. 2xy
4. A = 2, B = –1
5. –5p – 12q + 4r
6. –4
7. 25
8. (bx + a) (ax – b)
9. –1
10. (2x – a) (2x – b + a)
VERY SHORT ANSWER TYPE QUESTIONS
1. (i), (ii), (iii), (vi)
2. (i) 1, (ii) –2, (iii) 0, (iv) 34
3. (i) 2, (ii) 3, (iii), 2, (iv) 4
4. (i) Cubic, (ii) Quadratic, (iii) Quadratic, (iv) Linear (v) Cubic
5. (i) 9, (ii) 4+ 11, (iii) 4a4 + 5a3 – a2 + 6
6. (i) 4, (ii) –1/2, (iii) –1, –2, (iv) 1, 2, 3, (v) 0, (vi) –s/r
7. (i) Yes, (ii) Yes, both, (iii) x = 0, x = 1 is not zero, (iv) 
is not zero (v) Yes, both
8. (i) 4, (ii) –5, (iii) –2, (iv) 7
10. [–2]
11. [11]
12. [3]
13. [–7]
14. [15]
19. (x – 2) (x – 3)
20. [p = 5]
21. [a = 1]
22. [(x + 6) (x – 7)]
23. [(x + 1)2]
24. (y – 1) (y + 6)
25. (x – 6a) (x – 3a)
26. (a + 41) (a + 5)
27. (k – 7) (k – 19)
28. (a – b) (b + c)
29. (x – z) (2y – 1)
30. (10 – 3p) (10 + 3p)
31. (p + 3q) (p – 3q) (p2 + 9q2)
32. 1/5(x + 15) (x – 5)
33. (x – √2 ) (7√2 x + 4)
34. (3y – 2) (2x – 3)
35. (1 – 4x) (1 + 4x) (1 + 16x2)
36. (x2 – 2y + 3z) (x2 + 2y – 3z)
37. (2a + 1 – 3b2) (2a + 1 + 3b2)
38. (2√3 x – 5y) (12x2 + 10√3 xy + 25y2)
39. (5a + b/3 ) (25a2 – 5/3 ab + b2/9 )
40. a4x4 (a – x) (a + x)
41. (x – 4)
42. (6x + 5)
43. (i) ( 2a + 3b), (ii) ( 2x + 1) (x + 2)
44. (5a + 10b – 2) (a + 2b – 1)
45. 3(5x – 5y) + (5y – 3z) + (3z – 5x)
46. (3x + y + z) (9x2 + y2 + z2 – 3xy – yz – 3xz)
47. (i) x2 + 11x + 18, (ii) x2 + 6x – 16, (iii) z – 8z + 5, (iv) z4 – z2 – 20
48. (i) 10815, (ii) 9702, (iii) 9880
49. 
50. 
SHORT ANSWER TYPE QUESTIONS
51. (i) 81x2 + 4y2 + z2 + 36xy + 4yz + 18zx, (ii) 9x2 + 4y2 + z2 – 12xy + 4yz – 6xz
52. [8a2 + 2b2 + 2c2 + 4bc]
53. (i) 8x3 + 27y3 + 36x.y + 54xy., (ii) p3 – y3z3 – 3p2yz + 3py2z2
54. [7440]
55. [324]
56. (i) 1030301, (ii) 63521199
57. (i) x3 + 27, (ii) [125a3 – 27b3]
58. (i) (1 – 3z) (1 + 3z + 9z2), (ii) 2(5x – 2y) (25x2 + 10xy + 4y2), (iii) x(y + 9x) (y2 + 81x – 9xy)
59. [32]
60. [108]
61. [1]
62. [3(x – y) (y – z) (z – x)]
63. [–8700]
LONG ANSWER TYPE QUESTIONS
65. [m = 2, n = –1]
66. [k = 1]
67. [2x + 5]
68. [4x – 4]
69. (x + 1) (x + 2) (x – 2) (x – 3)
70. [a = 2]
71. 8a3
74. (i) 0 (ii) 0 (iii) 0
79. [0]
FAQs on Assignment - Polynomials, Class 9 Mathematics
| 1. What are polynomials? |  |
| 2. What is the degree of a polynomial? | |
| 3. How do you add and subtract polynomials? | |
| 4. What is the factor theorem? | |
| 5. How do you solve polynomial equations? | |
|
29.8K Views |
|
4.97/5 Rating |
|
Nov 25, 2024 Last updated |
|
Explore Courses for Class 9 exam
|
|
Assignment - Polynomials
,Class 9 Mathematics
,Semester Notes
,Assignment - Polynomials
,MCQs
,video lectures
,Class 9 Mathematics
,Assignment - Polynomials
,Free
,Class 9 Mathematics
,Previous Year Questions with Solutions
,Viva Questions
,Important questions
,Summary
,practice quizzes
,mock tests for examination
,ppt
,shortcuts and tricks
,Extra Questions
,Exam
,study material
,past year papers
,Objective type Questions
,Sample Paper
;
Assignment - Polynomials, Class 9 Mathematics Free PDF Download
Importance of Assignment - Polynomials, Class 9 Mathematics
Assignment - Polynomials, Class 9 Mathematics Notes
Assignment - Polynomials, Class 9 Mathematics Class 9 Questions
Study Assignment - Polynomials, Class 9 Mathematics on the App
|
© EduRev
|
Education Revolution
|
Follow Us
|
