Butterworth Filters | Analog and Digital Electronics - Electrical Engineering (EE) PDF Download
| Table of contents |

|
| Butterworth Filter |

|
| Butterworth Filter Design |

|
| Butterworth Filter Design using Cauer Topology |

|
| Butterworth Filter Design using Sallen-Key Topology |

|
| Digital Butterworth Filter |

|
The process or device used for filtering a signal from unwanted component is termed as a filter and is also called as a signal processing filter. To reduce the background noise and suppress the interfering signals by removing some frequencies is called as filtering. There are various types of filters which are classified based on various criteria such as linearity-linear or non-linear, time-time variant or time invariant, analog or digital, active or passive, and so on. Let us consider linear continuous time filters such as Chebyshev filter, Bessel filter, Butterworth filter, and Elliptic filter. Here, in this article let us discuss about Butterworth filter construction along with its applications.
Butterworth Filter
The signal processing filter which is having a flat frequency response in the passband can be termed as Butterworth filter and is also called as a maximally flat magnitude filter. In 1930 physicist and the British engineer Stephen Butterworth described about a Butterworth filter in his “on the theory of filter amplifiers” paper for the first time. Hence, this type of filter named as Butterworth filter. There are various types of Butterworth filters such as low pass Butterworth filter and digital Butterworth filter.
Butterworth Filter Design
The filters are used for shaping the signal’s frequency spectrum in communication systems or control systems. The corner frequency or cutoff frequency is given by the equation:
The Butterworth filter has frequency response as flat as mathematically possible, hence it is also called as a maximally flat magnitude filter (from 0Hz to cut-off frequency at -3dB without any ripples). The quality factor for this type is just Q=0.707 and thus, all high frequencies above the cut-off point band rolls down to zero at 20dB per decade or 6dB per octave in the stop band.
The Butterworth filter changes from pass band to stop-band by achieving pass band flatness at the expense of wide transition bands and it is considered as the main disadvantage of Butterworth filter. The low pass Butterworth filter standard approximations for various filter orders along with the ideal frequency response which is termed as a “brick wall” are shown below.
If the Butterworth filter order increases, then the cascaded stages within the Butterworth filter design increases and also the brick wall response & filter gets closer as shown in the above figure.
The frequency response of the nth order Butterworth filter is given as
Where ‘n’ indicates the filter order, ‘ω’ = 2πƒ, Epsilon ε is maximum pass band gain, (Amax). If we define Amax at cut-off frequency -3dB corner point (ƒc), then ε will be equal to one and thus ε2 will also be equal to one. But, if we want to define Amax at another voltage gain value, consider 1dB, or 1.1220 (1dB = 20logAmax) then the value of ε can be found by:
Where, H0 represents the maximum pass band gain and H1 represents the minimum pass band gain. Now, if we transpose the above equation, then we will get
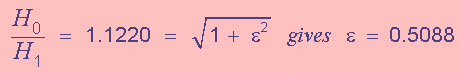
By using the standard voltage transfer function, we can define the frequency response of Butterworth filter as
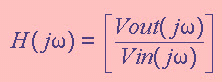
Where, Vout indicates voltage of output signal, Vin indicates input voltage signal, j is square root of -1, and ‘ω’ = 2πƒ is the radian frequency. The above equation can be represented in S-domain as given below
In general, there are various topologies used for implementing the linear analog filters. But, Cauer topology is typically used for passive realization and Sallen-Key topology is typically used for active realization.
Butterworth Filter Design using Cauer Topology
The Butterworth filter can be realized using passive components such as series inductors and shunt capacitors with Cauer topology – Cauer 1-form as shown in the figure below.
Where, Kth element of the circuit is given by
The filters starting with the series elements are voltage driven and the filters starting with shunt elements are current driven.
Butterworth Filter Design using Sallen-Key Topology
The Butterworth filter (linear analog filter) can be realized using passive components and active components such as resistors, capacitors, and operational amplifiers with Sallen-key topology.
The conjugate pair of poles can be implemented using each Sallen-key stage and to implement the overall filter we must cascade all stages in series. In case of real pole, to implement it separately as an RC circuit the active stages must be cascaded. The transfer function of the second order Sallen-Key circuit shown in the above figure is given by
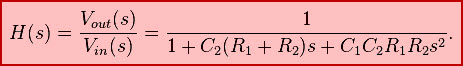
Digital Butterworth Filter
The Butterworth filter design can be implemented digitally based on two methods matched z-transform and bilinear transform. An analog filter design can be descritized using these two methods. If we consider Butterworth filter which has all-pole filters, then both the methods impulse variance and matched z-transform are said to be equivalent.
Application of Butterworth Filter
- The Butterworth filter is typically used in data converter applications as an anti-aliasing filter because of its maximum flat pass band nature.
- The radar target track display can be designed using Butterworth filter.
- The Butterworth filters are frequently used in high quality audio applications.
- In the motion analysis, digital Butterworth filters are used.
|
135 videos|181 docs|71 tests
|



















