Best Study Material for Electrical Engineering (EE) Exam
Electrical Engineering (EE) Exam > Electrical Engineering (EE) Notes > Engineering Mathematics for Electrical Engineering > Cauchy’s integral theorem and integral formula
Cauchy’s integral theorem and integral formula | Engineering Mathematics for Electrical Engineering - Electrical Engineering (EE) PDF Download
| Table of contents |
|
| Cauchy’s Integral Formula |
|
| Cauchy’s Integral Theorem |
|
| Generalisation of Cauchy’s Integral Formula |
|
| Solved Numericals |
|
Cauchy’s Integral Formula
Cauchy's integral formula is a fundamental result in complex analysis, a branch of mathematics. It asserts that a holomorphic function defined on a disk is completely determined by its values on the boundary of the disk. This formula provides integral representations for all derivatives of a holomorphic function. Named after Augustin-Louis Cauchy, this theorem is a cornerstone of complex analysis.Simply Connected Region
A region is termed as simply connected if, for any closed curve C within the region, all points inside C are also contained within the region. This implies that the region has no holes or isolated boundary points.
Cauchy’s Integral Theorem
Statement: If f(z) is an analytic function in a simply-connected region R, then ∫c f(z) dz = 0 for every closed contour c contained in R.If f(z) is an analytic function and its derivative f'(z) is continuous at all points within and on a simple closed curve C, then ∫c f(z) dz = 0.
Cauchy’s Integral Formula
If a complex function f(z) is analytic within and on a closed contour c inside a simply-connected domain, and if z0 is any point in the middle of C, then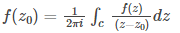
Here, the integral should be taken in the positive sense around c.Generalisation of Cauchy’s Integral Formula
If f(z) is an analytic function within and on a simple closed curve C and if z0 is any point within c, then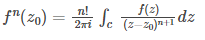
Converse of Cauchy’s Integral TheoremIf a complex function f(z) is continuous throughout the simple connected domain D and if ∫c f(z) dz = 0 for every closed contour c in D, then f(z) will be an analytic function in D.
This theorem is also known as Morera’s theorem.
 |
Download the notes
Cauchy’s integral theorem and integral formula
|
Download as PDF |
Download as PDF
Solved Numericals
Q1. The value of the integral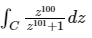
where C is the circle of radius 2 centred at the origin taken in the anti-clockwise direction is:Solution:
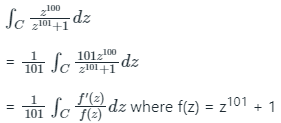
f(z) is a polynomial of degree 101 so number of zeros of f(z) is 101
Hence using Cauchy's argument principle,
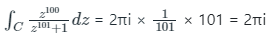
Q2. What is the value of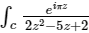 
, where C is the circle |z| = 1 is with positive orientation.
Solution: Given,
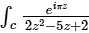
C is the circle |z| = 1 is with positive orientation
Let f(z) =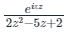 
Putting 2z2 - 5z + 2 = 0
⇒ 2z2 - 4z - z + 2 = 0
⇒ 2z(z - 2) - 1(z - 2) = 0
⇒ (z - 2)(2z - 1) = 0
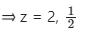
Now z =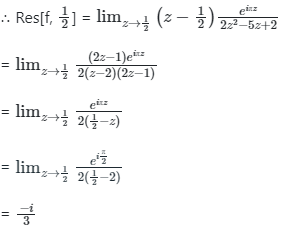 
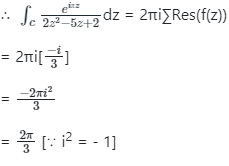
∴ The value of the given integral is 2π/3.
Q3. The value of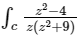 
is where C is a circle |z| = 1,
Solution: Given,
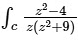
C is a circle, lzl=1
Let f(z) =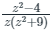 
Putting z(z2 + 9) = 0
⇒ z = 0, 3i, - 3i
Now, z = 0 lies inside C.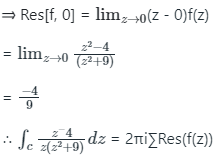
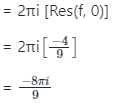
∴ The value of given integral is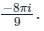
Q4. Let γ be the positively oriented circle in the complex plane given by {z ∈ ℂ: |z – 1| = 1}. Then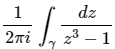 
equals:
Solution: Given,
Let γ be the positively oriented circle in the complex plane given by {z ∈ ℂ: |z – 1| = 1}. Then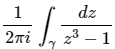
Cauchy Integral Formula -
Let f(z) be analytic in a region D and let C be a closed curve in D. If a is any point in D, then
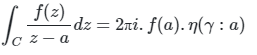
here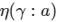 
is a winding number. The winding number measures the number of times a path (counter-clockwise) winds around a point.
We have γ = {z ∈ ℂ: |z – 1| = 1}.
Now using Cauchy Integral formula we get -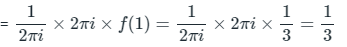
The document Cauchy’s integral theorem and integral formula | Engineering Mathematics for Electrical Engineering - Electrical Engineering (EE) is a part of the Electrical Engineering (EE) Course Engineering Mathematics for Electrical Engineering.
All you need of Electrical Engineering (EE) at this link: Electrical Engineering (EE)
|
44 videos|102 docs|58 tests
|
FAQs on Cauchy’s integral theorem and integral formula - Engineering Mathematics for Electrical Engineering - Electrical Engineering (EE)
| 1. What is Cauchy’s Integral Formula? |  |
| 2. How is Cauchy’s Integral Formula different from Cauchy’s Integral Theorem? | |
Ans. Cauchy’s Integral Formula is used to calculate the value of a function at a point inside a closed curve \( C \), while Cauchy’s Integral Theorem states that if a function is analytic inside and on a simple closed curve \( C \), then the line integral of that function around \( C \) is equal to zero.
| 3. What is the significance of Cauchy’s Integral Formula in Mechanical Engineering? | |
Ans. In Mechanical Engineering, Cauchy’s Integral Formula is often used in the analysis and design of systems involving fluid flow, heat transfer, and stress distribution. It helps in calculating complex integrals to determine important parameters in engineering applications.
| 4. How is Cauchy’s Integral Formula applied in real-world engineering problems? | |
Ans. Cauchy’s Integral Formula is applied in real-world engineering problems to analyze the stress distribution in mechanical components, predict the flow of fluids in pipes and channels, and determine the temperature distribution in heat transfer systems. It helps engineers make informed decisions based on mathematical models.
| 5. What are some common misconceptions about Cauchy’s Integral Formula and Theorem? | |
Ans. One common misconception is that Cauchy’s Integral Formula and Theorem are only applicable in theoretical mathematics and have no practical engineering applications. In reality, these concepts play a crucial role in solving complex engineering problems and optimizing design processes.
Related Exams
About this Document
4.66/5
Rating
Mar 29, 2025
Last updated
Document Description: Cauchy’s integral theorem and integral formula for Electrical Engineering (EE) 2025 is part of Engineering Mathematics for Electrical Engineering preparation.
The notes and questions for Cauchy’s integral theorem and integral formula have been prepared according to the Electrical Engineering (EE) exam syllabus. Information about Cauchy’s integral theorem and integral formula covers topics
like Cauchy’s Integral Formula, Cauchy’s Integral Theorem, Generalisation of Cauchy’s Integral Formula, Solved Numericals and Cauchy’s integral theorem and integral formula Example, for Electrical Engineering (EE) 2025 Exam. Find important definitions, questions, notes, meanings, examples, exercises and tests below for Cauchy’s integral theorem and integral formula.
Introduction of Cauchy’s integral theorem and integral formula in English is available as part of our Engineering Mathematics for Electrical Engineering
for Electrical Engineering (EE) & Cauchy’s integral theorem and integral formula in Hindi for Engineering Mathematics for Electrical Engineering course.
Download more important topics related with notes, lectures and mock test series for Electrical Engineering (EE)
Exam by signing up for free. Electrical Engineering (EE): Cauchy’s integral theorem and integral formula | Engineering Mathematics for Electrical Engineering - Electrical Engineering (EE)
Description
Full syllabus notes, lecture & questions for Cauchy’s integral theorem and integral formula | Engineering Mathematics for Electrical Engineering - Electrical Engineering (EE) - Electrical Engineering (EE) | Plus excerises question with solution to help you revise complete syllabus for Engineering Mathematics for Electrical Engineering | Best notes, free PDF download
Information about Cauchy’s integral theorem and integral formula
In this doc you can find the meaning of Cauchy’s integral theorem and integral formula defined & explained in the simplest way possible. Besides explaining types of
Cauchy’s integral theorem and integral formula theory, EduRev gives you an ample number of questions to practice Cauchy’s integral theorem and integral formula tests, examples and also practice Electrical Engineering (EE)
tests
Related Searches
shortcuts and tricks
,mock tests for examination
,Cauchy’s integral theorem and integral formula | Engineering Mathematics for Electrical Engineering - Electrical Engineering (EE)
,Sample Paper
,Previous Year Questions with Solutions
,video lectures
,Summary
,Cauchy’s integral theorem and integral formula | Engineering Mathematics for Electrical Engineering - Electrical Engineering (EE)
,practice quizzes
,Viva Questions
,Cauchy’s integral theorem and integral formula | Engineering Mathematics for Electrical Engineering - Electrical Engineering (EE)
,ppt
,Important questions
,MCQs
,Extra Questions
,Semester Notes
,Exam
,Objective type Questions
,past year papers
,study material
,Free
;
Additional Information about Cauchy’s integral theorem and integral formula for Electrical Engineering (EE) Preparation
Cauchy’s integral theorem and integral formula Free PDF Download
The Cauchy’s integral theorem and integral formula is an invaluable resource that delves deep into the core of the Electrical Engineering (EE) exam.
These study notes are curated by experts and cover all the essential topics and concepts, making your preparation more efficient and effective.
With the help of these notes, you can grasp complex subjects quickly, revise important points easily,
and reinforce your understanding of key concepts. The study notes are presented in a concise and easy-to-understand manner,
allowing you to optimize your learning process. Whether you're looking for best-recommended books, sample papers, study material,
or toppers' notes, this PDF has got you covered. Download the Cauchy’s integral theorem and integral formula now and kickstart your journey towards success in the Electrical Engineering (EE) exam.
Importance of Cauchy’s integral theorem and integral formula
The importance of Cauchy’s integral theorem and integral formula cannot be overstated, especially for Electrical Engineering (EE) aspirants.
This document holds the key to success in the Electrical Engineering (EE) exam.
It offers a detailed understanding of the concept, providing invaluable insights into the topic.
By knowing the concepts well in advance, students can plan their preparation effectively.
Utilize this indispensable guide for a well-rounded preparation and achieve your desired results.
Cauchy’s integral theorem and integral formula Notes
Cauchy’s integral theorem and integral formula Notes offer in-depth insights into the specific topic to help you master it with ease.
This comprehensive document covers all aspects related to Cauchy’s integral theorem and integral formula.
It includes detailed information about the exam syllabus, recommended books, and study materials for a well-rounded preparation.
Practice papers and question papers enable you to assess your progress effectively.
Additionally, the paper analysis provides valuable tips for tackling the exam strategically.
Access to Toppers' notes gives you an edge in understanding complex concepts.
Whether you're a beginner or aiming for advanced proficiency, Cauchy’s integral theorem and integral formula Notes on EduRev are your ultimate resource for success.
Cauchy’s integral theorem and integral formula Electrical Engineering (EE) Questions
The "Cauchy’s integral theorem and integral formula Electrical Engineering (EE) Questions" guide is a valuable resource for all aspiring students preparing for the
Electrical Engineering (EE) exam. It focuses on providing a wide range of practice questions to help students gauge
their understanding of the exam topics. These questions cover the entire syllabus, ensuring comprehensive preparation.
The guide includes previous years' question papers for students to familiarize themselves with the exam's format and difficulty level.
Additionally, it offers subject-specific question banks, allowing students to focus on weak areas and improve their performance.
Study Cauchy’s integral theorem and integral formula on the App
Students of Electrical Engineering (EE) can study Cauchy’s integral theorem and integral formula alongwith tests & analysis from the EduRev app,
which will help them while preparing for their exam. Apart from the Cauchy’s integral theorem and integral formula,
students can also utilize the EduRev App for other study materials such as previous year question papers, syllabus, important questions, etc.
The EduRev App will make your learning easier as you can access it from anywhere you want.
The content of Cauchy’s integral theorem and integral formula is prepared as per the latest Electrical Engineering (EE) syllabus.
|
© EduRev
|
Education Revolution
|
|
Signup to see your scores
go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup