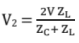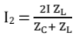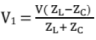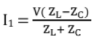Chapter 10 - Power System (Part - 1) | Additional Study Material for Mechanical Engineering PDF Download
1
. Transmission and Distribution
Basic Concepts and Line Constants in Transmission

Transmission Line: It is a connection (or) line between the remote generating station and the distribution center.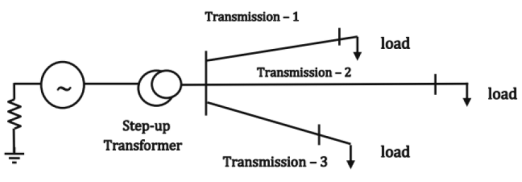
Level of Voltages
- Low voltage:- 220V 1-phase (or) 415 V 3-phase
- High voltage:- 11kV, 33kV
- Extra high voltage:- 66kV, 132kV, 220kV and 400kV
- Ultra high voltage:- 765kV and above
Note: Most of power generation in India at 11 kV
Necessity of Extra High Voltages for Transmission System
- The size of the conductor is reduced so that the cost of the conductor is reduced.
- The transmission line loss will be reduced.
- The transmission efficiency will increase
The selection of operating voltage to transmit the power is a compromise between saving of conductor cost and extra cost required for insulation.
Types of Conductor
Solid Copper Conductor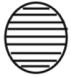 Solid(i) High Cost
Solid(i) High Cost
(ii) High Tensile Strength
(iii) Difficult to string the conductors.
(iv) High skin effect while using on ac system
Stranded Conductor: It consists of two or more smaller cross sectional strands (or) filaments which are twisted together to get the required strength and running in parallel to increase the current capacity for the given operating voltage.
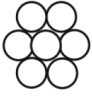 Stranded
Stranded
ACSR (Aluminum Conductor and Steel Rainforced)
Advantages
- Required tensile strength
- Less cost of transmission
- Easy stringing
- Reduced skin effect when compared to solid (or) Homogenous stranded conductor.
- Self GMD is increased as inductance is less
Skin Effect: The non uniform distribution of current through the given cross sectional area of the conductor when it is operated on alternating current system is called skin effect. The main reason for the skin effect is non-uniform distribution of flux linkages. The skin effect will result in
(i) Increased effective resistance (Rac)
(ii) Internal Inductance will increase (Lin)
(iii) External Inductance will reduced (Lexternal)
(iv) Non uniform distribution of current
Bundle conductors: Whenever the operating voltage goes beyond 270kv, it is preferable to use more than one sub conductor/phase which is known as bundle conductor.
Advantages of Bundle Conductor
(i) Voltage gradient (or) field intensity will reduced
(ii) Due to reduced field Intensity the critical disruptive voltage will increase. So the corona loss is reduced.
(iii) The reduced corona loss will reduce the communication interference with the adjoining communication lines.
(iv) Due to increased GMR Inductance/phase will be reduced and capacitance/phase will increase.
(v) Characteristic impedance will be reduced.
(vi) Characteristic impedance loading will increase.
Geometric Mean (or) Mutual Distance and Geometric Mean Radius
These are mathematical concepts which are useful to evaluate the inductance and capacitance of 3-phase transmission lines.
GMD: Used for the calculation of Inductance and capacitance.
GMR: Used only for the calculation of Inductance
GMR: The transmission line conductor having only one conductor per phase, the GMR is the distance between the centers to the circumference (i.e.) it is equal to radius of the conductor. However due to internal external flux linkages it is equal to r' = re-1

GMD: Mutual distance: If a point 'p' is surrounded by 'n' other points which are scattered in the space. The mutual distance of'p' will be the Geometric means of the individual distance between the point 'p' and n other points.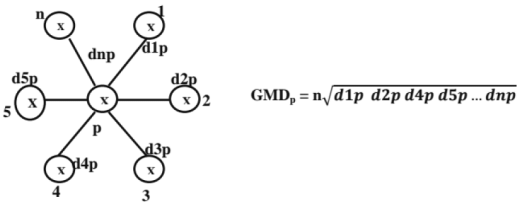
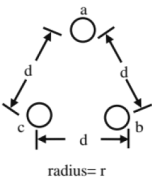
Self GMD: GMR is employed whenever there is only one conductor/phase. However there may be cases where more than sub conductor/phase are also employed to avoid the concept of corona. The concept which is used to calculate the self distance for the sub conductor configuration is called self GMD.
Transposition of lines:- The concept of transposition of lines is considered if the load is balance. However, it is a old concept and not suitable for modern power system. The inductance/phase of un-transposed unsymmetrical will be the average inductances of the three phases.
Transmission line parameters: The Transmission line consists of a series combination of resistance, Inductance and a parallel combination of capacitance conductance.
Resistance Calculation: It is expressed as K = ρl/a Ω / km. In case of transmission line, the distance is in kilometers. So the parameters will be calculated based on per km length.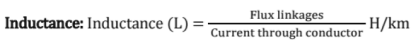
It will produce magnetic field and the energy stored in the inductor Q1 = 1/2 LI2.
Inductance of 1-Φ 2-wire (or) loop Inductance (or) Circuit Inductance
Lab = 0.4 loge (d/r1)] mH/km [copper conductor]
Inductance of 1-Phase 2-Wire as Earth Return
La = 0.2 loge (h/r) height > d
Inductance of 3-phase 3-wire Transmission
line Unsymmetrical Transmission Line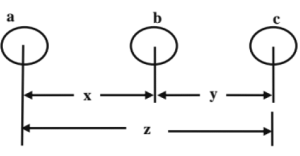
L = 0.2 loge (GMD / GMR) mH/km / phase.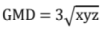
GMR = r'
In Case of Symmetrical Conductors
Inductance /phase = 0.2 loge (GMD/GMR) mH/phase/km
GMD = d
GMR = r'
Capacitance Calculation
Capacitance of 1-Phase 2-Wire System - Isolated Earth Plane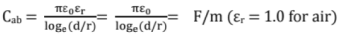
Where, d = distance between the conductors and, r = radius of the conductor.
Capacitance to Neutral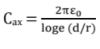
Capacitance of 3-Phase 3-Wire Transmission Line
Unsymmetrical Spacing
By using transposition of lines, the capacitance / phase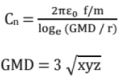
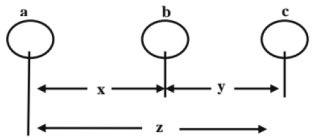
Symmetric Spacing
x = y = z = d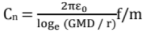
GMD = d distance of separation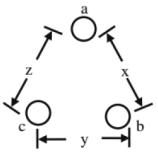
Capacitance of 1-Ø 2-Wire Transmission Line With The Effect of Earth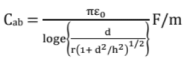
Where h = height of the conductor from ground
Performance of Transmission Lines The transmission line is a static device. So the performance for the transmission line is analyzed by considering the efficiency and voltage regulation.
The transmission line is a static device. So the performance for the transmission line is analyzed by considering the efficiency and voltage regulation.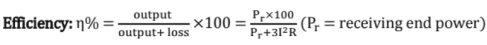
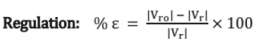
Vro = no load receiving voltage
Vr = full load receiving voltage
Representation of transmission lines: The transmission can be represented based on the length in which the power is carried out.
(a) short transmission lines: less than 80km.
(b) medium transmission line: 80km to 2 5 Okm.
(c) long transmission line: more than 250km.
Uniform Distributed Parameters: These parameters are physical and electrically not separable.
Uniform distributed parameters are considered to evaluate the transient behavior of long transmission lines, (i.e.) switch closed condition. Exact mathematical solution is considered to evaluate the sending end voltage and current.
Lumped parameters are considered to evaluate the steady state behavior of long transmission lines. The two possible network configurations are.
(i) Equivalent - T
(ii) Equivalent - π
The most and effective way of representing the transmission line is using two port network configuration. Port means pair of terminals.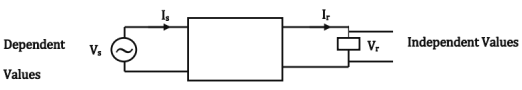
The dependent values are expressed in terms of independent values, with certain parameters and those parameters are called transmission line parameters (or) A, B, C and D parameters.
Vs = AVr + BIr .......... (1)
Is = CVr + Dlr ..........(2)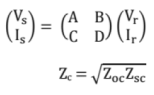
where Zoc = A/C = sending end impedance with receiving end O.C
Zsc = B/D = sending end impedance with receiving end S.C
Zc = the characteristic impedance of the line.
For a symmetrical transmission line A = D
For a reciprocal transmission line → AD-BC = 1
Short transmission line: Series combination of resistance and Inductance. & we can take shunt capacitance is almost negligible.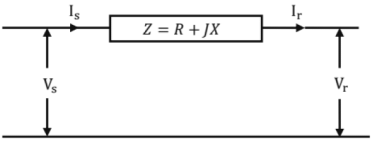 Vs = Zls + Vr = ZIr + Vr
Vs = Zls + Vr = ZIr + Vr
Is = Ir = Ir + OVr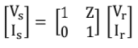
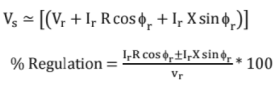
'+' stands for lagging p.f
'-' stands for leading p.f
Medium Transmission line
Nominal -T Network
A = 1 + ZY / 2 C = Y
B = Z (1 + ZY / 4) D = 1 + ZY / 2
A = D and AD - BC = 1
∴ Symmetric and reciprocal network
Nominal - II
A = 1 + ZY / 2, B = Z, C = Y (1 + ZY / 4), D = 1 + ZY/2
A = D and AD - BC = 1. So the model is symmetric and reciprocal.
Load end Capacitance
A = 1 + ZY, B = Z, C = Y and D = 1.0
Sending end Capacitance
A = 1.0,13 = Z, C = Y and D = 1 + ZY
Power Transfer Equations: Receiving End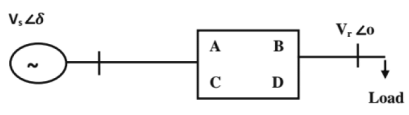 The receiving end power transfer Sr = Vr lr. The purpose of the conjugate is to ensure that the real power is always positive and to assign the polarity for inductive or capacitive reactive powers.
The receiving end power transfer Sr = Vr lr. The purpose of the conjugate is to ensure that the real power is always positive and to assign the polarity for inductive or capacitive reactive powers.
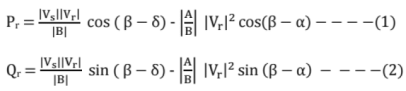
Characteristic Impedance loading: In a lossless transmission line, it is the amount of power delivered to the load through a transmission line in which the load is terminated by impedance which is equal to characteristics impedance of transmission line. The nature of characteristic impedance will be resistive so the nature of the load is resistive. Also called surge impedance loading (SIL)
Ferranti effect: when the transmission line operating at no load (or) light load condition, the receiving end voltage is more than the sending voltage. This phenomenon is called ferranti effect. It is more severe in long transmission line.
The Steady State ABCD Values of Long Lines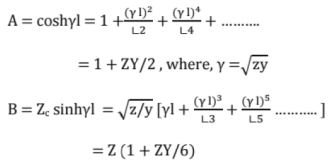
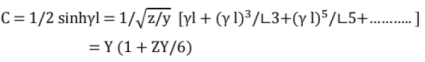
D = 1 + ZY/2
Wave Propagation Line
Terminated by an Impedance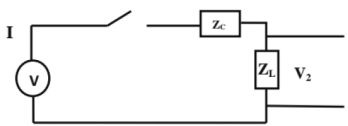 ZC = Characteristic impedance of line
ZC = Characteristic impedance of line
ZL = Load impedance
V = Incident voltage
I = Incident current

V2 = Refracted voltage
I2 = Refracted Current
V1 = Reflected voltage
I1 = Reflected Current
ZL = 0 for short-circuit, and, ZL = ∞, for open-circuit.
Voltage Control
The Methods of Voltage Control
Concepts of Corona
Corona: The ionization of insulating material (air) surrounding the surface of the conductor of a transmission line (or) the disruption of the dielectric strength of air near the conductor of a transmission line.
The voltage at which the self sustained discharge will be initiated is called the critical disruptive voltage.
The corona initiation can be identified as
(a) Hissing noise,
(b) Releasing of ozone gas,
(c) Occurrence of beds and tufts
Critical Disruptive Voltage of a 1-Phase 2-Wire Transmission Line
Critical disruptive voltage Vd = g0 r loge (d/r)
r = radius of conductor in cm
d = distance of separation in m.
g0 = dielectric strength of air
= 30kv/cm (peak)
= 21.1kv/cm (rms) at NTP
Vd = g1 r loge (d/r) kv/rms
g1 = Dielectric strength at any temperature and pressure.
g1 = g0 δ
δ = Air density factor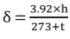
h = Atmospheric pressure in cm of Hg.
t = temperature in C°
The surface of conductor is irregular. So consider the surface irregularity factor (m)
Vd = 21.1 m δ r loge(d/r) kv/rms
Visual Critical Disruptive Voltage
The visual critical disruptive voltage
(Vv) = 21.1 mv δ (1 + 0.3/√r δ) r loge(d/r) kV/rms.
mv = Surface irregularity factor
mv = 1.0 for smooth surface of the conductor
mv = 0.93-0.98 for rough conductor (or) standard conductor
The visual corona will be observed as white bluish slow color.
Critical Disruptive Voltage For 3 Phases System
Vd = 21.1δm GMR; pge (GMD/GMR) kv/rms/phase
GMR = Self distance
GMD = Mutual distance
Corona Loss
f = supply frequency in Hz
δ = air density factor
r = radius of conductor in cm.
d = distance of separation in m.
Vp = operating voltage/phase/rms
Vd = critical disruptive voltage rms/phase
Factors Influencing Corona Loss
(a) Supply frequency increases the corona loss will increase, because P ∝ (f +25)
(b) The corona loss of Ac Transmission line is more than DC transmission line for the same operation voltage.
(c) The corona loss at positive polarity conductor at a DC transmission line is more than negative polarity conductor. When the sinusoidal wave form is having distortion (i/e) consists of harmonics, the corona loss will increase.
(d) Lower the height at the power conductor, higher will be the corona loss.
(e) Temperature& pressure.
(f) Deposition of dust, ice, snow and fog,
(g) Size of conductor.
Disadvantages of Corona
- There is certain real power loss as part from ohmic loss.
- When the corona is initiated on transmission lines, the overall diameter of the conductor will increase so that the capacitance will increase.
Advantages
Corona will act as a safety value against direct lightening, by dissipating the peak magnitude of lightening strokes in the form of corona loss.
Method of Reducing Corona Loss
- Use larger diameter of the conductor
- Use hollow conductor
- Use bundled conductors
Mechanical Design of Transmission Lines
Sag: A perfectly flexible wire of uniform cross section when suspended between two points hangs in the form of a natural cantenary curve. The difference in level between the points of supports & the lowest point is known as sag.
Factors Affecting the Sag are
- Weight of conductor
- Length ofspan
- Working tensile strength
- Temperature.
Sag Tension Calculations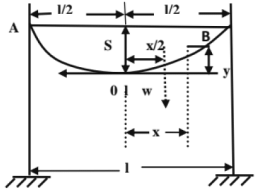
(A) Support at Same Level
I = length of span in meters
T = Tension in Newton
w = Weight in Newton/meter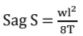
(B) Effect of Wind & Ice Loading
In still air, sag develops due to the weight of conductor only. In actually practice, a conductor's may have ice coating simultaneously subjected to wind pressure. Fig a & b the ice load (wi) acts vertically downwards i.e., in the same direction as the as the weight of conductor whereas the wind load (ww) acts horizontally i.e., perpendicular to the weight of conductor as fig (c).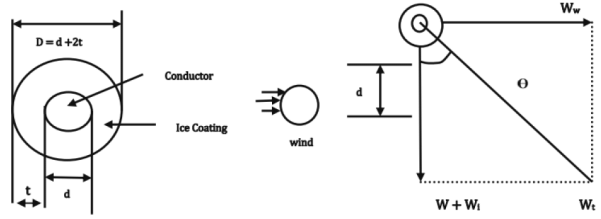
Resultant weight of conductor per unit length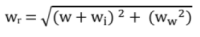
Where w = weight of conductor per unit length
= (Conductor material density) x (Conductor volume per unit length)
Wi = Weight of ice per unit length
= Density of ice x [(d + 2t)2 - d2] x 1
ww = Wind load per unit length
= Wind pressure x (d + 2t) 1
Overhead Insulators
Over Head Insulators: The insulators for over head lines provide insulation to the power conductor from the ground. The insulators are connected to the cross arm of the supporting structure & power conductor passes through the clamp of the insulator. The insulators are to avoid leakage of current through the support of the earth. Thus the insulators play important role in the successful operation of over head lines.

Where 'n' is the no of insulators in the string, sov is spark over voltage.
- String efficiency always less than one or unity.
- The voltage across various units will tend to be equal in case the value of'm' is large.
- In the case of high voltage lines since the clearance between the conductors & the tower structure should be more to avoid flash over under normal operating condition.
- The mutual capacity being fixed the ground capacitance goes on decreasing with large clearance & hence the ratio of the two capacitances goes on increasing.
- The unit nearest the cross-arm should have the minimum capacitance maximum reactance & as we go towards the power conductor the capacitance should be increased by using grading equalizing the potential all units.
String Effeciency Can Be Improved
- By using longer cross - arms.
- By grading the insulators.
- By using a guard ring.
Under Ground Cables
Cable: The combination of conductor & its insulators is called cable.
Underground cables are used in the place of overhead lines to have following advantage & disadvantages
Advantages
- In a densely populated circuits where overhead lines are not possible.
- Underground cables provides better regulation
- The chances of accidents in underground are very low compared to overhead lines
- As the cables are laid underground with better insulation, the chance of failure or fault are less compared to overhead lines.
- It helps in reducing lightening over voltage as its characteristic impedance is very low.
Disadvantages
- Underground cables are very costly as compared to overhead lines.
- Practically, identification of cable faults is difficult than in case of faults in the overhead lines.
- Joining of cables is difficult. Hence tapping for loads & service mains is not convenient in underground system.
- Its surge impedance loading (maximum load that can be transmitted) is very low.
Construction of Cables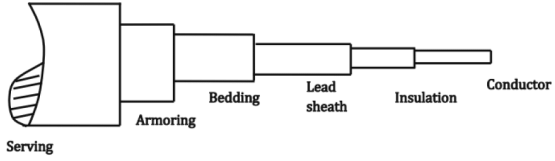 Types of cable:
Types of cable:
- Single core
- Three core
(1) Single core: It consists of stranded copper conductor a belt of insulation of impregnated paper & lead sheaths over it. The sheath is protected by covering it with hessian tapes or jute which is soaked in some preservative compound of bituminous nature.
Insulation Resistance of Single Core Cable
Let 'I' be the length of the cable in meter.
'ρ' be the resistivity of the insulator in Ω - meters
'r' be the radius of single core cable of conductor.
'R' be the internal sheath
Insulation resistance,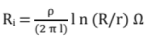
Capacitance of a Single Core Cable
Potential difference between the conductor & sheath.
V = q/(2 π k) In (D/d) volts.
Let 'D' be the initial sheath diameter
'd' be the conductor diameter
K = k0kr, k0 is the primitively of free space = 8.854 x 10-12 F/m
kr is the primitively of the insulation.
'q' be the charge per meter axial length of the cable in coulombs.
Capacity of the cable is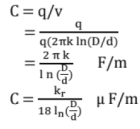
Electric Stress
V = Potential difference between the core & sheath
G = Q/2πkx = V/x In (D/d) kV /cm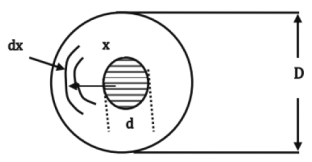
The electrical stress is maximum at the surface of conductor, i.e., when x = r
gmax = 2V/ (d In (D/d)) or V/(r In (D/d))
stress is minimum when x = R.
gmin = V/ R In (D/d)
or
2V/ D In (D/d)
gmax / grain = R/r
gmax becomes least value when
In (D/d) = 1 i.e., D/d = e
Therefore d = D/2.718
It is concluded that is maximum stress at the conductor & minimum at the sheath. By distributing the stress uniformly, the breakdown of insulation can be avoided this may be two methods.
(a) By using metallic inter sheaths
(b) By using insulating materials of different dielectric constants
Inter sheath grading: The inter sheaths made of metallic cylinders one or more are interested in the dielectric between the conductor & lead sheath to fix up the potentials at that distance from the surface of core in the insulation . The inter sheaths do not carry any part of working current, but carries the current which is the difference between the charging currents taken by section on each side.
3 layers. Two inter sheath are inserted between cable throughout length.
g1max = (V - V1) / r ln (di/d)
g2max = (V1 - V2) / r In (d2/d)
g3max = V2 / r2 In (D/d2)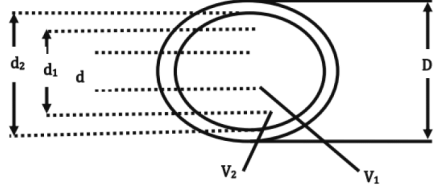
The stress can be made to vary between the same maximum & minimum value by choosing d1 & d2
Such that d1/d = d2/d1 = D/d2 = α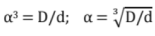
d1 = αd d2 = α2 d
the max stress are required to be made equal, we have g1max = g2max = g3max
V2 = V1 (1 + 1/α) = V(1 + 1/α + 1/α2)
One Inter Sheath
d1/d = D/d1 = α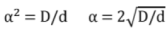
d1 = αd
V2 = (1 + 1/α) V
(1 + 1/α + 1/α2)
gmax with two inter sheath / gmax without inter sheath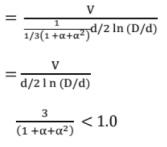
gmax with two inter sheath / gmax without inter sheath = 2/(1 + α)
The new max stress with two inter sheath is only 3/(1 + α + α2) Times that of stress without any inter sheath.
Capacitance Grading
The insulation may be made of dielectric of different permittivity such a cable is known as graded cable & the arrangements results in more uniform stress in the dielectric.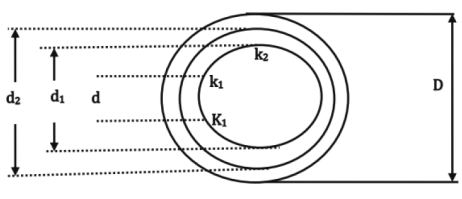
Let d1 be the diameter of the dielectric having permittivity k1 & D the diameter of the dielectric having permittivity k2.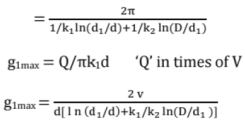
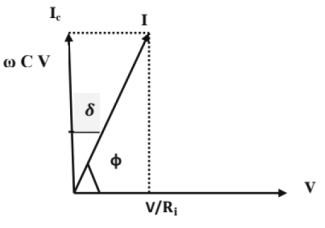
Power Factor in Cables
Capcitance in 3 - Core Cable
The three - core cable has capacitance between the cores and core core capacitance with sheath is shown below fig.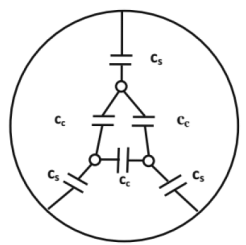
capacitance Cc to the core are in delta & can be replaced by an equivalent star arrangement shown fir.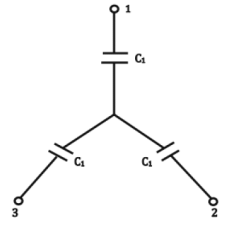
The impedance between core 1 and the star point must be equal to (1/3) times the impedance of each branch of the delta,
this gives 1/ωC1 = 1/3ωC1 (or) C1 = 3Cc.
the star may be assumed to be at zero potential and if sheath is also at zero potential the capacitance of each conductor to neutral is
C0 = C1 + Cs = 3 Cc + Cs
Methods of calculation of Cs & Cc
(a) Let conductors 2 and 3 be connected to the sheath. Capacities Cc between conductors 2 and 3 and Cs of conductor 2 and 3 with respect to the sheath are eliminated.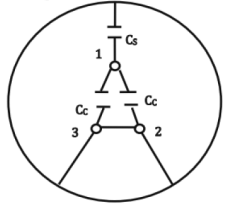
Capacitances Cc and Cs are now in parallel across core one and the sheath and they add up Measure the capacitance between core one and the sheath, is
Ca = Cs + 2Cc ............ (1)
(b) All the conductors are connected together and capacitance Cb is measured between them and sheath.
Cb = 3 Cs or
Cs = 1/3 Cb............. (2)
Since 2Cc = Ca - Cs
Cc = 1/2 (Ca - 1/3 Cb)
= 1/6 (3Ca - Cb)
Cn = C per phase
From 1 and 2 Cn = Cs + 3Cc = 3/2 Ca - Cb/6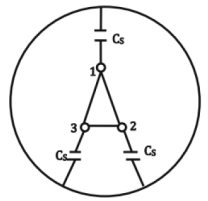
(c) Connect any one conductor to sheath, measure capacitance between remaining two conductors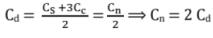
Distribution System
DC Distribution: The electric power is almost exclusively generated, transmitted and distributed as a.c. but for certain applications (e.g for electro-chemical works, for the operation of variable speed machinery d.c. motors etc.) D.C. is absolutely necessary. For this purposes, a.c is converted into d.c. at the sub-station and is then distributed by i) 2 -wire system ii) 3 - wire system.
AC Distribution: The electric power (or energy) is invariably generated, transmitted and distributed in the form of alternating current. The main reason of adopting a.c. system for generation, transmission and distribution of electric power is that the alternating voltage can conveniently be changed to any desired value with the help of a transformer.
Primary Distribution: The system in which electric p ow er is conveyed at 11 k V or 6.6 kV or 3.3 kV to different sub-stations for distribution or to big consumers (e.g industries, factories etc) is called primaiy distribution system.
Secondary Distribution System: The system in which electric power is distributed at 400/230 V to various consumers (e.g residential consumers) is called low voltage or secondary distribution system.
Connection Schemes of Distribution System
Radial system: In radial system, separate feeders radiate from a single substation and feed the distributors at one end only.
Ring Main System: In this system each consumer is supplied via two feeders. The arrangement is similar to two feeders in parallel on different routes.
Inter Connected System: In this system, the feeder ring is energized by two or more than two generating stations or substations.
2. Economics of Power Generation
Load Curve: It is plot of load in kilowatts versus time (usually for a day or a year.) in the order in which they occur, i.e.r chronologically
Load Duration Curve: It is the plot of load in kilowatts versus time duration for which it occurs in the descending order of magnitude, irrespective of the time of occurrence
Load Factor: The ratio of average load to the maximum demand during a given period is known as load factor.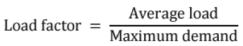
Average Load: The average of loads occurring on the power station in a given period is known as average load or average demand.

Maximum Demand: It is the greatest demand of load on power station during a given period.
Demand Factor: It is the ratio of maximum demand on the power station to its connected load,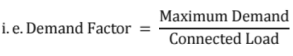
Connected Load: It is the sum of continuous ratings of all the equipments connected to the supply system.
Diversity Factor: The ratio of the sum of individual maximum demands to the simultaneous maximum demand on the power station is known as diversity factor.
Coincidence Factor: It is the recip rocal of diversity factor and is always less than '1'.
Plant Capacity Factor: It is defined as the ratio of average demand on the station to the maximum installed capacity.
Operation Factor: It is given by the ratio of number of hours the plant is in service to the total number of hours in a given period (usually a year)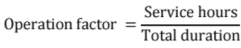
Utilization Factor (Plant Use Factor): It is the ratio of kWh generated to the product of plant capacity and the number of hours for which the plant was in operation.
Firm Power: It is the power intended to be always available.
Cold Reserve: It is that reserve generating capacity which is available for service but is not in operation.
Hot Reserve: It is that reserve generating capacity which is in operation but is not in service.
Spinning Reserve: It is that generating capacity which is connected to bus and is ready to take load.
Methods for Determining Depreciation: There is reduction in cost of equipment and other property of the plant every year due to depreciation. There are three methods for determining the annual depreciation namely:
(a) Straight Line Method
(b) Diminishing Value Method
(c) Sinking Fund Method.
Base Load and Peak Load On Power Station
Base Load: The unvarying load which occurs almost the whole day on the station is known as base load.
Peak Load: The various peak demands of load over and above the base load of the station is known as peak load. Nuclear power stations are used as base load stations operating at high load factors of over 80%. These meet what are called the 'block loads' at the bottom of the load curves.
Load Forecasting: Forecasting of future demand in every utility service is very important and necessary to meet out the consumer demand efficiently. For estimating the future demand of electricity, load forecasting is required. It is broadly classified as
(i) Long Term Load Forecasting (LTLF) and
(ii) Short Term Load forecasting (STLF).
Economics of Power Generation: The art of determining per unit cost of production of electrical energy is known as economics of power generation. Cost of electrical energy can be divided into two parts namely
(i) Fixed cost
(ii) Variable cost
(i) Fixed Cost: It is determined by the capital investment, interest charge, tax paid, salaries and other expenses that continue irrespective of load.
(ii) Variable Cost: It is a function of loading on generating units, losses, daily load requirements etc. Economic operation is concerned about minimizing the variable cost Proper scheduling of power plants (thermal and hydel) is done to obtain economic operation.
Expressions for Cost of Electrical Energy
The overall annual cost of electrical energy generated by a power station can be expressed in two forms viz three part form and two part form.
Economic Load Dispatch
The economic load dispatch involves the solution of two different problems. These are unit commitment (or) preload dispatch and on-line economic load dispatch.
Unit commitment (or) Preload dispatch: Select optimally out of the available generating sources to meet the expected load and provide a specified margin of operating reserve over a specified period of time.
On-line Economic Dispatch: It is required to distribute the load among the generating units actually parallel with the system in such manner as to minimize the total fuel cost minute-to- minute requirements of the system.
The economic load dispatch problem applicable for fuel based units rather than hydro electric stations.
The relation between fuel cost and the power generation in MW of a particular unit (ith) in n units is given as
Fi = 1/2 α Pi2 + βPi + γRs/hr α, β and γ are called the constants.
Incremental Fuel Cost Relation
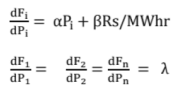
The incremental fuel costs of all the units are same.
λ = cost received in Rs/Mwhr.
Economic Load Dispatch Including Line Losses
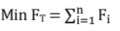
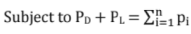
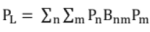
Where Pm = real power generated at the mth plant
Bmn = loss coefficients under certain assumed conditions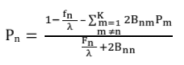
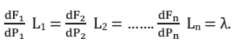
L1 = L2 = Ln = Penalty factor.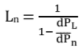
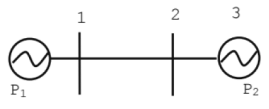
Kor a two Bus system
PL = B11 P12 + P1 + B12 P2 + P2 B21 P1 + B22 P22
B11, B12, B21, B22, = Loss coefficients.
Special Case: When the load is located at Bus (2)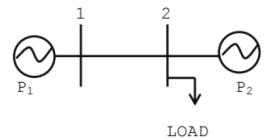
B12 = 0
B21 = 0
B22 = 0
PL = B11P12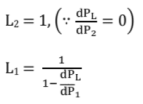
|
1 videos|30 docs|57 tests
|