Algebra Class 6 Notes Maths Chapter 11
| Table of contents |

|
| What is Algebra? |

|
| Matchstick Pattern |

|
| The Idea of a Variable |

|
| More Examples of Variables |

|
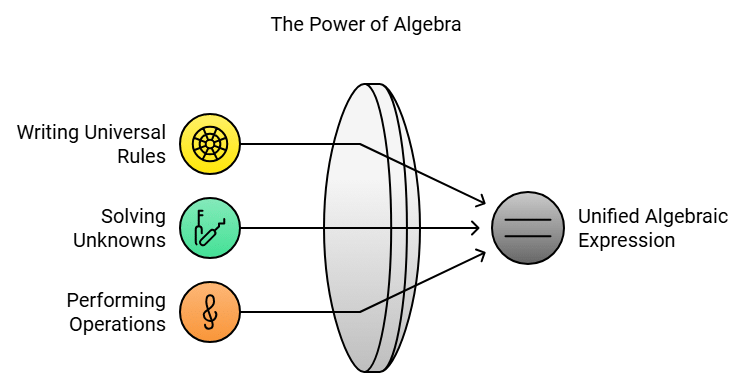
What is Algebra?
Algebra is a part of math that lets us show problems or situations using mathematical expressions.
In algebra, we use letters to represent numbers. This allows us to:
- Write rules that work for any number.
- Solve problems where numbers are unknown.
- Perform operations like addition and multiplication with these letters.

Why Learn Algebra?
Algebra helps us solve real-life problems and puzzles. It's a powerful tool that makes math even more useful and interesting.
Matchstick Pattern
Suppose you're arranging matchsticks to form a pattern. Each time you form one shape, it requires 2 matchsticks.
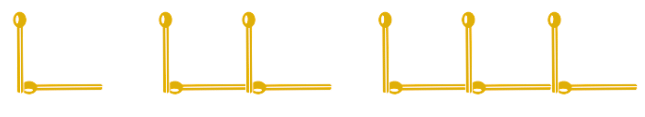
- If you make 1 shape, you need 2 matchsticks.
- If you make 2 shapes, you need 4 matchsticks.
- If you make 3 shapes, you need 6 matchsticks.
Notice the pattern: the number of matchsticks needed is always twice the number of shapes. We can express this with a simple algebraic rule:
- Number of matchsticks needed = 2 × number of shapes
- Or, if we let n represent the number of shapes:
- Number of matchsticks needed = 2 × n
So,
For 3 shapes:
2 × 3 = 6 matchsticks
For 7 shapes:
2 × 7 = 14 matchsticks
This formula, 2n, allows you to quickly calculate the total number of matchsticks needed for any number of shapes, making it easier to solve such problems without drawing or counting every time.
Example: Find the rule which gives the number of matchsticks required to make a pattern of the letter H. Use a variable to write the rule.
Ans: To form the letter H, you need 2 vertical matchsticks and 1 horizontal matchstick. For n letters H:The pattern for n letters H will require 2 vertical sticks for each letter and 1 horizontal stick connecting the letters.
For n letters, the total number of matchsticks is:
Total matchsticks = 3n
This is because each letter H requires 3 matchsticks.

The Idea of a Variable
In the previous example, we found a rule to calculate how many matchsticks are needed to create a pattern of "L" shapes:
Number of matchsticks required = 2n
Here, n represents the number of "L" shapes in the pattern. n can be any number—1, 2, 3, 4, and so on. As n changes, the total number of matchsticks changes too.
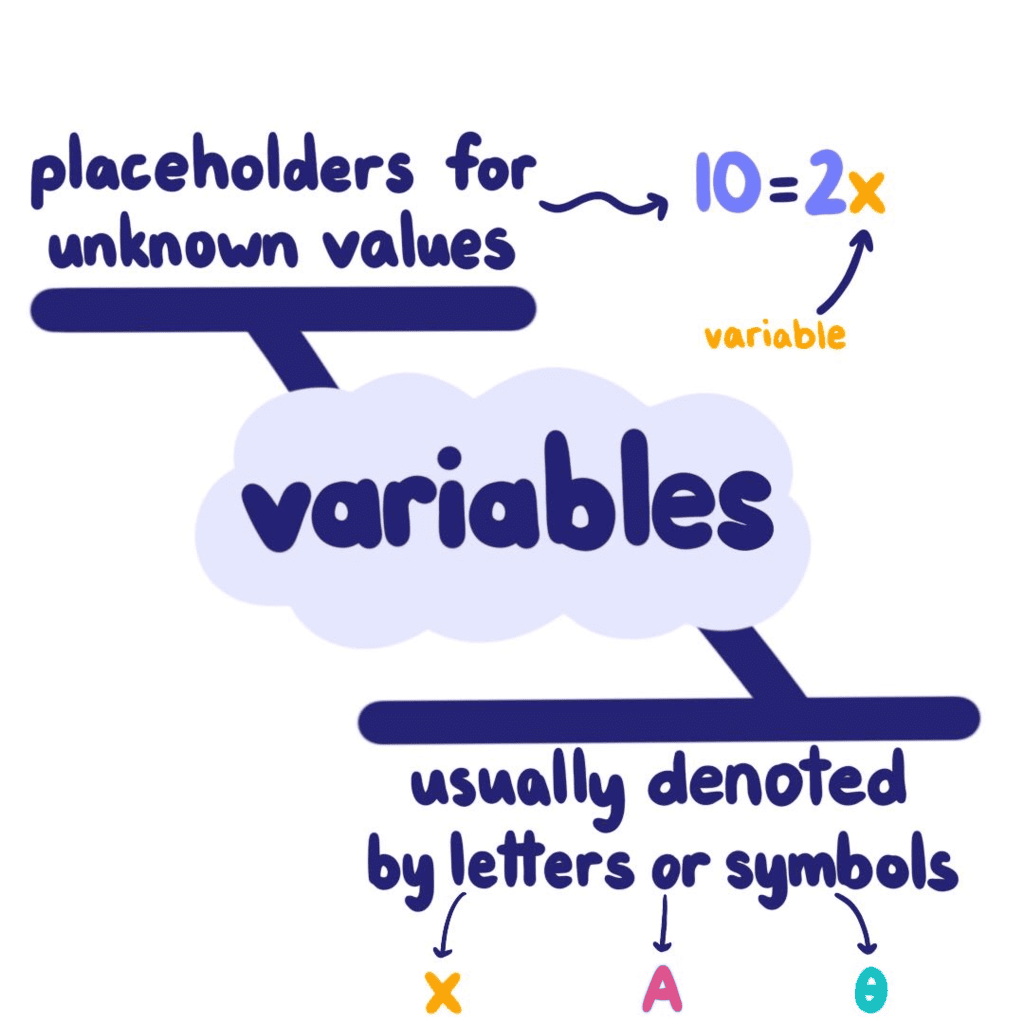
What is a Variable?
In this example, n is called a variable. The word "variable" comes from the idea that its value can vary or change. It's not a fixed number; it can be different each time.

For instance:
If n = 2, then 2n = 4 matchsticks.
If n = 5, then 2n = 10 matchsticks.
A variable, like n, is something in math that can take on different values. It’s useful because it allows us to create rules (like 2n) that work for any number. This makes solving problems quicker and more flexible.
More Examples of Variables
In math, we often use letters like n, m, x, or y to represent a variable. A variable is a number that can change; it doesn’t have a fixed value.
For example:
n can be 1, 2, 3, or any other number.
m could be 5, 10, 15, etc.
There’s nothing special about the letter n—we could use any letter like m, x, or y instead. The important thing is that a variable can take different values.
Fixed Numbers vs. Variables
Fixed Numbers: Numbers like 5, 100, or 3 (the number of angles in a triangle) are not variables because they don’t change. They always have the same value.
Variables: n, on the other hand, can vary. It can be 1, 2, 3, and so on, depending on the situation.
So, in math, variables are letters we use to represent numbers that can change or vary.
Solved Examples
Q1: There are 6 chairs in each row in a hall. What is the rule that gives the total number of chairs, given the number of rows? Use r for the number of rows.
Ans: To find the total number of chairs:
Each row contains 6 chairs.
For r rows, the total number of chairs is:
Total chairs = 6 × r = 6r
Q2: If each box contains 12 books, how will you write the total number of books in terms of the number of boxes? Use b for the number of boxes.
Ans: To find the total number of books:
Each box contains 12 books.For b boxes, the total number of books is:
Total books = 12 × b = 12b
Q3: Ankit is Ravi’s older brother. Ankit is 5 years older than Ravi. If Ravi’s age is y years, how can you write Ankit's age in terms of y?
Ans: To find Ankit's age in terms of Ravi's age:
Ravi’s age is y years.
Since Ankit is 5 years older than Ravi, you add 5 to Ravi’s age to determine Ankit’s age.
Thus, Ankit’s age is:
Ankit's age = y + 5
So, if Ravi is y years old, Ankit will be y + 5 years old.
Q4: A basket contains 8 apples. If you have p baskets, how many apples are there in total? Use p for the number of baskets.
Ans: To find the total number of apples:
Each basket contains 8 apples.
For p baskets, the total number of apples is:
Total apples = 8 × p
Q5: Tina is transferring apples from a big basket into smaller baskets. When Tina finishes with the big basket, it can fill 4 smaller baskets and still has 12 apples left over. If each smaller basket holds z apples, how many apples were in the big basket?
Ans: To determine the number of apples in the big basket:
Each smaller basket holds z apples.
The big basket fills 4 smaller baskets, so the total number of apples that fill these baskets is 4z.
Additionally, there are 12 apples left over after filling the smaller baskets.
Thus, the total number of apples in the big basket is:
Total apples = 4z + 12
So, if each smaller basket contains z apples and there are 12 apples left over, the big basket originally had 4z + 12 apples.
|
92 videos|348 docs|54 tests
|
FAQs on Algebra Class 6 Notes Maths Chapter 11
| 1. What is the definition of algebra? |  |
| 2. How does the matchstick pattern illustrate algebraic concepts? |  |
| 3. What is the role of a variable in algebra? |  |
| 4. Can you provide examples of real-life situations where variables are used? |  |
| 5. How can I improve my understanding of algebra? |  |