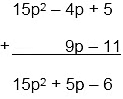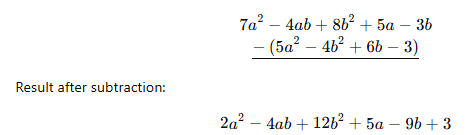Algebraic Expressions and Identities Chapter Notes | Mathematics (Maths) Class 8 PDF Download
What are Algebraic Expressions?
Any mathematical expression that consists of numbers, variables and operations are called Algebraic Expression.
Components of Algebraic Expression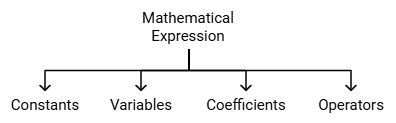
- Constants: Fixed numerical values. For example, in the expression 3 +4, the number 4 is a constant.
- Variables: Symbols that represent unknown values and can change. For example, in 3 +4 is a variable.
- Coefficients: Numbers that are multiplied by the variables. In the expression , 3 is the coefficient of .
- Operators: Symbols that indicate mathematical operations, such as +, , ×, and ÷.
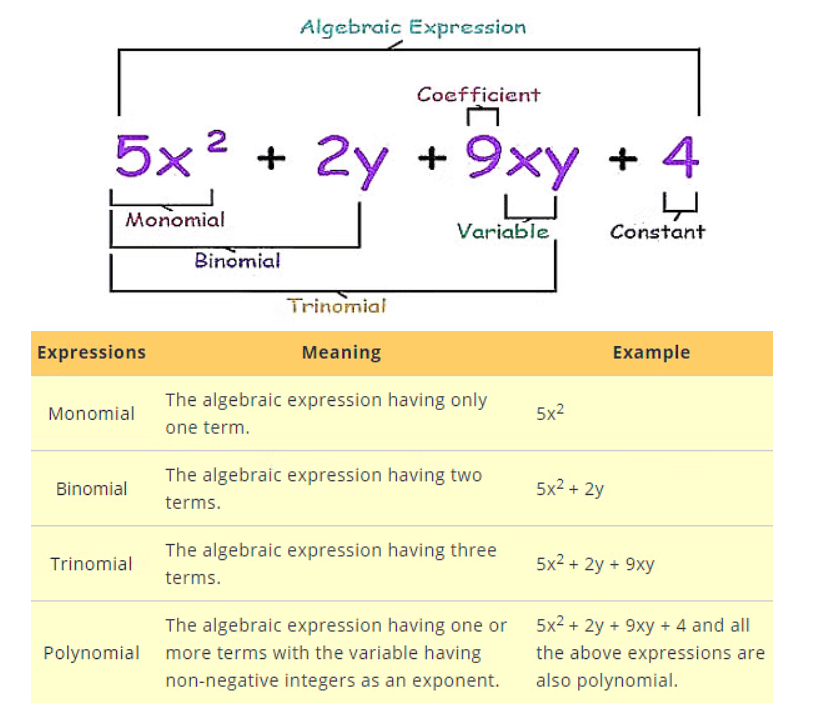
Monomials, Binomials and Polynomials
Addition and Subtraction of Algebraic Expressions
Steps to Add or Subtract Algebraic Expression
- First of all, we have to write the algebraic expressions in different rows in such a way that the like terms come in the same column.
- Add them as we add other numbers.
- If any term of the same variable is not there in another expression then write is as it is in the solution.
Example 1:Add 15p2 – 4p + 5 and 9p – 11
Solution:
Write down the expressions in separate rows with like terms in the same column and add.
Example 2: Subtract 5a2 – 4b2 + 6b – 3 from 7a2 – 4ab + 8b2 + 5a – 3b.
Solution:
For subtraction also write the expressions in different rows. But to subtract we have to change their signs from negative to positive and vice versa.
Multiplication of Algebraic Expression: Introduction
While multiplying, we need to take care of some points about the multiplication of like and unlike terms.
1. Multiplication of Like Terms
The coefficients will get multiplied.The power will not get multiplied but the resultant variable will be the addition of the individual powers.Example3:The product of 4x and 3x will be 12x2.
(4x) x (3x) = 12x2
Example 4:The product of 5x, 3x and 4x will be 60x3.
(5x) x (3x) x (4x) = 60x3
2. Multiplication of Unlike Terms
- The coefficients will get multiplied.
- The power will remain the same if the variable is different.
- If some of the variables are the same then their powers will be added.
Example5:The product of 2p and 3q will be 6pq
(2p) x (3q) = 6pq
Example 6:The product of 2x2y, 3x and 9 will be 54x3y
(2x2y) x (3x) x (9) = 54x3y
Multiplying a Monomial by a Monomial
Multiplying Two Monomials
While multiplying two polynomials the resultant variable will come by
- The coefficient of product = Coefficient of the first monomial × Coefficient of the second monomial
- The algebraic factor of product = Algebraic factor of the first monomial × Algebraic factor of the second monomial.
Example 7:
(3x2) × (4x3) = 12x2+3 = 12x5
Explanation: Multiply the coefficients 3 × 4 = 12 and add the exponents of x (2 + 3 = 5).
Example 8:
(2a3b) × (5ab2) = 10a3+1b1+2 = 10a4b3
Explanation: Multiply the coefficients 2 × 5 = 10, add the exponents of a (3 + 1 = 4), and add the exponents of b (1 + 2 = 3).
Multiplying Three or More Monomials
While multiplying three or more monomial the criterion will remain the same.
Example 9:
4xy × 5x2y2 × 6x3y3 = (4xy × 5x2y2) × 6x3y3
= 20x3y3 × 6x3y3
= 120x3y3 × x3y3
= 120(x3 × x3) × (y3 × y3)
= 120x6y6
= 120x6y6
We can do it in another way also:
4xy × 5x2y2 × 6x3y3
= (4 × 5 × 6) × (x × x2 × x3) × (y × y2 × y3)
= 120x6y6
Example 10: Find the volume of each rectangular box with given length, breadth, and height.
Length Breadth Height 2ax 3by 5cz m2n n2p p2m 2q 4q2 8q3 Volume = length × breadth × height
For (i) volume = (2ax) × (3by) × (5cz)
= 2 × 3 × 5 × (ax) × (by) × (cz)
= 30abcxyzFor (ii) volume = m2n × n2p × p2m
= (m2 × m) × (n × n2) × (p × p2)
= m3n3p3For (iii) volume = 2q × 4q2 × 8q3
= 2 × 4 × 8 × q × q2 × q3
= 64q6
Multiplying a Monomial by a Polynomial
Multiplying a monomial by a binomial
To multiply a monomial with a binomial we have to multiply the monomial with each term of the binomial.
Example 11: Simplify 2x × (3x + 5xy).
Solution: 2x × (3x + 5xy) = (2x × 3x) + (2x × 5xy)
= 6x2 + 10x2y
Example 12: Simplify a2 × (2ab − 5c).
Solution: a2 × (2ab − 5c) = (a2 × 2ab) − (a2 × 5c)
= 2a3b − 5a2c
Multiplying a Monomial by a Trinomial
Consider 4q × (5q2 + 6q + 8).
As in the earlier case, we use the distributive law:
4q × (5q2 + 6q + 8) = (4q × 5q2) + (4q × 6q) + (4q × 8)
= 20q3 + 24q2 + 32q
Multiply each term of the trinomial by the monomial and add products.
Example 13: Simplify the expressions and evaluate them as directed:
(i) x(x − 4) + 3 for x = 2
(ii) 5z(3z − 9) − 4(z − 2) − 45 for z = −1
Solution:
(i) x(x − 4) + 3 = x2 − 4x + 3
For x = 2:
x2 − 4x + 3 = (2)2 − 4(2) + 3
= 4 − 8 + 3 = −1
(ii) 5z(3z − 9) − 4(z − 2) − 45 = 15z2 − 45z − 4z + 8 − 45
= 15z2 − 49z − 37
For z = −1:
15z2 − 49z − 37 = 15(−1)2 − 49(−1) − 37
= 15 + 49 − 37 = 27
Multiplying a Polynomial by a Polynomial
Multiplying a binomial by a binomial
We use the distributive law of multiplication in this case. Multiply each term of a binomial with every term of another binomial. After multiplying the polynomials we have to look for the like terms and combine them.
Example 14: Simplify (3a + 4b) × (2a + 3b)
Solution:
(3a + 4b) × (2a + 3b)
= 3a × (2a + 3b) + 4b × (2a + 3b) [distributive law]
= (3a × 2a) + (3a × 3b) + (4b × 2a) + (4b × 3b)
= 6 a2 + 9ab + 8ba + 12b2
= 6 a2 + 17ab + 12b2 [Since ba = ab]
Multiplying a Binomial by a Trinomial
In this, we also have to multiply each term of the binomial with every term of the trinomial.
Example 15: Simplify (p + q) (2p – 3q + r) – (2p – 3q) r.
Solution:
We have a binomial (p + q) and one trinomial (2p – 3q + r)
(p + q) (2p – 3q + r)
= p × (2p – 3q + r) + q × (2p – 3q + r) [distributive law]
= 2p2 – 3pq + pr + 2pq – 3q2 + qr
= 2p2 – pq – 3q2 + qr + pr (–3pq and 2pq are like terms)
(2p – 3q) r = 2pr – 3qr
Therefore,
(p + q) (2p – 3q + r) – (2p – 3q) r
= 2p2 – pq – 3q2 + qr + pr – (2pr – 3qr)
= 2p2 – pq – 3q2 + qr + pr – 2pr + 3qr
= 2p2 – pq – 3q2 + (qr + 3qr) + (pr – 2pr)
= 2p2 – 3q2 – pq + 4qr – pr
What is Identity?
An identity is an equality that is true for every value of the variable but an equation is true for only some of the values of the variables.
So, an equation is not an identity.
Like, x2 = 1, is valid if x is 1 but is not true if x is 2.so it is an equation but not an identity.
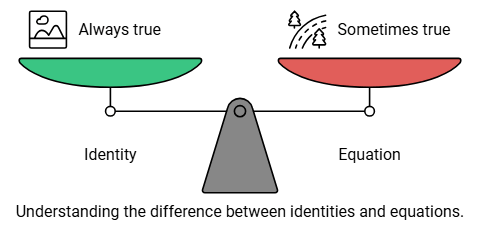
Standard Identities
(a + b)2 = a2 + 2ab + b2
(a - b)2 = a2 – 2ab + b2
a2 – b2 = (a + b) (a - b)
(x + a) (x + b) = x2 + (a + b)x + ab
(a + b + c)2 = a2 + b2 + c2 + 2ab + 2bc + 2ca
These identities are useful in carrying out squares and products of algebraic expressions. They give alternative methods to calculate products of numbers and so on.
|
81 videos|423 docs|31 tests
|
FAQs on Algebraic Expressions and Identities Chapter Notes - Mathematics (Maths) Class 8
| 1. What are algebraic expressions and how are they different from numerical expressions? |  |
| 2. How do you add and subtract algebraic expressions? |  |
| 3. What is the process for multiplying a monomial by a polynomial? |  |
| 4. Can you explain what identities are in algebra? |  |
| 5. What are standard identities in algebra and provide an example? |  |