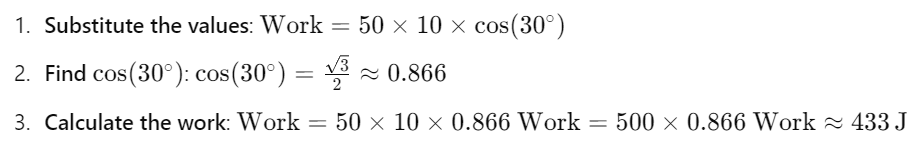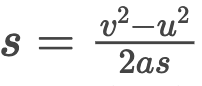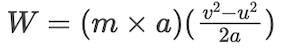Work and Energy Class 9 Notes Science Chapter 10

Work, Power, and Energy

Building on the foundation of the laws of motion, we now explore the interconnected concepts of Work, Power, and Energy—key principles that form the backbone of mechanics and thermodynamics.

Understanding Work, Power, and Energy is crucial because they explain how forces interact with objects, how energy is transferred and transformed, and how systems function efficiently in nature and technology. 

Flowchart

What is Work?

In our daily lives, we often refer to"work" as activities that require physical or mental effort. However, the scientific definition of work might differ from our usual understanding.

For instance, if you push a rock and it doesn’t move, even if you feel tired, scientifically, no work has been done.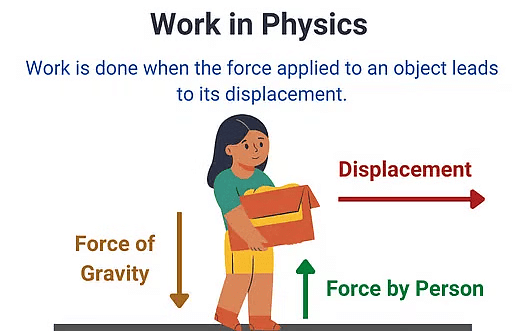 

Scientific Conception of Work

In scientific terms, work is defined as applying force to an object that causes it to move. Work done is calculated by multiplying the force applied by the distance the object moves in the direction of that force. Here are a few examples to clarify this concept:

• Pushing a pebble: When you push a pebble and it moves, you apply force, and the pebble is displaced. Work is done here.
• Pulling a trolley: If a girl pulls a trolley and it moves, the force she applies and the trolley's movement mean work is done.
• Lifting a book: When you lift a book, your force moves it upwards, so work is accomplished.

For work to occur, two key conditions must be satisfied:

• There must be an application of force on the object.
• The object must be displaced in the direction of the force.

It's important to note that if there is no movement of the object, then the work done is zero. Additionally, an object capable of doing work is said to have energy.

Mathematically, work done is calculated as: 

Work done = force x displacement

where:

• F is the constant force applied.
• S is the displacement in the direction of the force.

The SI unit of work is the joule (J or Nm). Work has magnitude but no direction.

In summary, work occurs when a force makes an object move in that direction, and it is measured by the product of force and distance moved.

Work is a fundamental concept linked to energy, which exists in different forms such as kinetic energy and potential energy. Understanding work is essential for learning about energy conservation and transformation.

Clear all your Class 9 doubts with EduRev Join for Free

Join for Free

Work Done By A Constant Force

Work done on an object is defined as the amount of force multiplied by the distance the object moves in the direction of the applied force. 

• Work has only magnitude and no direction. 
• The unit of work is joule: 1 joule = 1 newton × 1 meter. 
• Here, the unit of work is Newton metre (Nm) or joule (J). 
• Thus, 1 J is the amount of work done on an object when a force of 1 N displaces it by 1 m along the direction of the force.

Work done on an object by a force would be zero if the displacement of the object is zero.

Example 1

A force of 10 Newtons is applied to an object, causing it to be displaced by 5 meters. What is the work done on the object?

We can use the formula: W = F x S
Force (F) = 10 Newtons
Displacement (S) = 5 meters
Putting these values into the equation, we have:
W = (10 N) x (5 m) = 50 Joules

Therefore, the work done on the object is 50 Joules.

Example 2

A porter lifts a luggage of 15 kg from the ground and puts it on his head 1.5 m above the ground. Calculate the work done by him on the luggage.

• Mass of luggage, m = 15 kg
• Displacement, s = 1.5 m
• Work done, W = F × s = mg × s = 15 kg × 10 m/s² × 1.5 m = 225 kg m/s² m = 225 Nm = 225 J

Work done is 225 J.

Force at an Angle

When a force is applied at an angle to the direction of displacement, only a part of the force causes motion. The formula to calculate work in such cases is: Work = Force × Distance × cos(θ). 

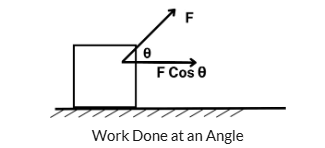
Where:

• Force is the magnitude of the constant force applied.
• Distance is the displacement of the object in the direction of the force.
• θ is the angle between the force and the displacement.

If the force and displacement are in the same direction (θ = 0), the formula simplifies to: Work = Force × Distance. This means that work done by a constant force is equal to the product of the force applied and the distance over which the force acts.

Example 3

A box is pushed with a force of 50 N at an angle of 30° to the horizontal. If the box moves 10 m, calculate the work done.

• Force (F) = 50 N
• Angle (θ) = 30°
• Distance (d) = 10 m

Formula: Work = F × d × cos(θ)

Step-by-step solution:

Answer: The work done is approximately 433 J (Joules).

Positive, Negative & Zero Work Done

• Positive Work: Work is considered positive if the displacement of the object is along the direction of the force applied. 
  Example: Work done by a man is taken positive when he moves from the ground floor to the second floor of his house.
• Negative Work: Work is taken as negative if the displacement of the object is in the direction opposite to the force applied. 
  Example: Work done by the man is negative when he descends from the second floor of the house to the ground floor.

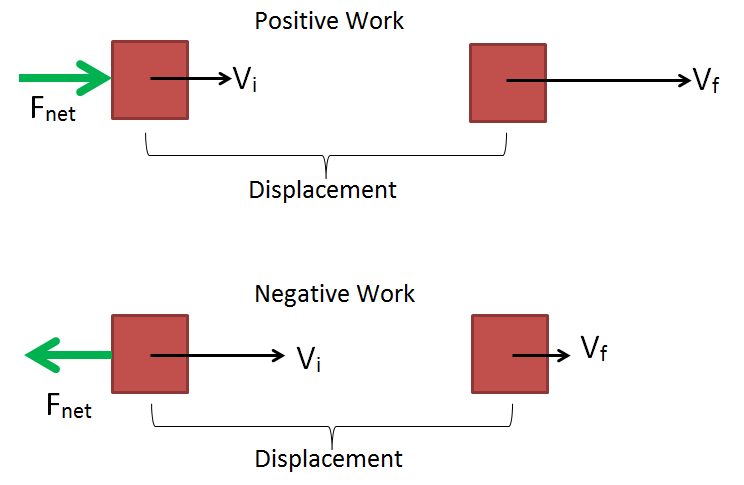Positive and Negative Work Done

• Zero Work Done: If the displacement of an object is in a direction perpendicular to the application of force, work done is zero despite the fact that force is acting and there is some displacement too. 
  Example: Imagine pushing a lawn roller forward. While gravity pulls it downward, the roller moves horizontally. Since gravity acts perpendicular to the roller's movement, it does no work. This is an example of zero work, where a force doesn't contribute to the displacement.

The gravitational potential energy of an object of mass, m raised through a height, h from the earth's surface is given by mgh.

What is Energy?

An object that can perform work is said to have energy. Thus, the energy of an object is its ability to do work. The unit for energy is the same as that for work, making the SI unit for energy the joule (J).

Energy Transformation 

Forms of Energy

Energy exists in various forms in nature, including:

• Mechanical energy
• Heat energy
• Electrical energy
• Light energy
• Chemical energy
• Nuclear energy

Mechanical energy can be divided into two types:

• Kinetic energy
• Potential energy

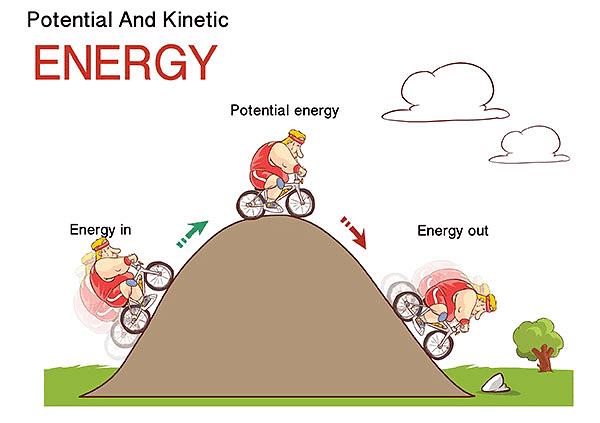Potential and Kinetic Energy

Kinetic Energy

The kinetic energy of an object is the energy possessed by it by virtue of its state of motion. A speeding vehicle, a rolling stone, a flying aircraft, flowing water, blowing wind, and a running athlete possess kinetic energy.

For an object of mass m and having a speed v, the kinetic energy is given by:

F = ma

Also, W=Fs

From the second equation of motion, we know that

v² - u² = 2as

Rearranging the equation, we get

Substituting equation for work done by a moving body,

Taking initial velocity as zero, we get
where:

• E_k is the kinetic energy.
• m is the mass of the object.
• v is the velocity of the object

Note : When two identical bodies are in motion, the body with a higher velocity has more KE.

Potential Energy

An object gains energy when it is lifted to a higher position because work is done against the force of gravity. This energy is known as gravitational potential energy. Gravitational potential energy is defined as the work done to raise an object from the ground to a certain height against gravity.

Let's consider an object with a mass m being lifted to a height h above the ground.

The minimum force required to lift the object is equal to its weight, which is mg(where g is the acceleration due to gravity).

The work done on the object to lift it against gravity is given by the formula:

Work Done (W) = Force × Displacement

W = mg × h = mgh

The energy gained by the object is equal to the work done on it, which is mgh units. 

This energy is the potential energy (E_p) of the object.

E_p = mgh

Note: It's important to note that the work done by gravity depends only on the difference in vertical heights between the initial and final positions of the object, not on the path taken to move the object. For example, if a block is raised from position A to position B by taking two different paths, as long as the vertical height AB is the same (h), the work done on the object is still mgh.

Download the notes Chapter Notes: Work and Energy

Download as PDF

Download as PDF

Potential Energy of an Object at Height

Let's consider an object with a mass m being lifted to a height h above the ground.

The minimum force required to lift the object is equal to its weight, which is mg (where g is the acceleration due to gravity).

The work done on the object to lift it against gravity is given by the formula:

Work Done (W) = Force × Displacement

W = mg × h = mgh

Since work done on the object is equal to mgh, an energy equal to mgh units is gained by the object. This is the potential energy (E_p) of the object.

Potential Energy (E_p) = mgh

Note: The work done by gravity depends only on the difference in vertical heights between the initial and final positions of the object, not on the path taken to move the object. For example, if a block is raised from position A to position B by taking two different paths, as long as the vertical height AB is the same (h), the work done on the object is still mgh.

Example 5

Find the energy possessed by an object of mass 10 kg when it is at a height of 6 m above the ground.
Given g = 9.8 m/s².

• Mass (m) = 10 kg
• Height (h) = 6 m
• Acceleration due to gravity (g) = 9.8 m/s²

Using the formula for potential energy: E_p = mgh

Substituting the values, we have:

Potential Energy = 10 × 9.8 × 6 = 588 J

Therefore, the potential energy of the object is 588 Joules.

Example 6

An object of mass 12 kg is at a certain height above the ground. If the potential energy of the object is 480 J, find the height at which the object is with respect to the ground. Given g = 10 m/s².

Mass of the object, m = 12 kg, potential energy, E = 480 J.

Using the formula E = mgh:

480 J = 12 × 10 × h

Solving for h:

h = 480 J / 120 kg m/s² = 4 m

The object is at a height of 4 m.

Law of Conservation of Energy

According to the law of conservation (transformation) of energy, we can neither create nor destroy energy. Energy can be changed from one form to another, but the total energy remains unchanged. The sum of kinetic energy and potential energy of an object is its total mechanical energy.

• For a freely falling object, the potential energy decreases progressively as it falls, transforming into kinetic energy. This transformation does not violate the law of conservation of energy; rather, it illustrates it, as the total mechanical energy remains constant throughout the fall.
• As the object falls, its potential energy will change into kinetic energy. If v is the velocity of the object at a given instant, the kinetic energy would be 1/2 mv². When the object is about to reach the ground, h = 0 and v will be at its highest. Therefore, the kinetic energy would be the largest and potential energy the least. However, the sum of the potential energy and kinetic energy of the object would be the same at all points. That is,

Potential Energy + Kinetic Energy = Constant

or

mgh + 1/2 mv² = constant

Law of Conservation of Energy

The diagram below shows a pendulum, which consists of a mass (m) connected to a fixed pivot point via a string of length (L).

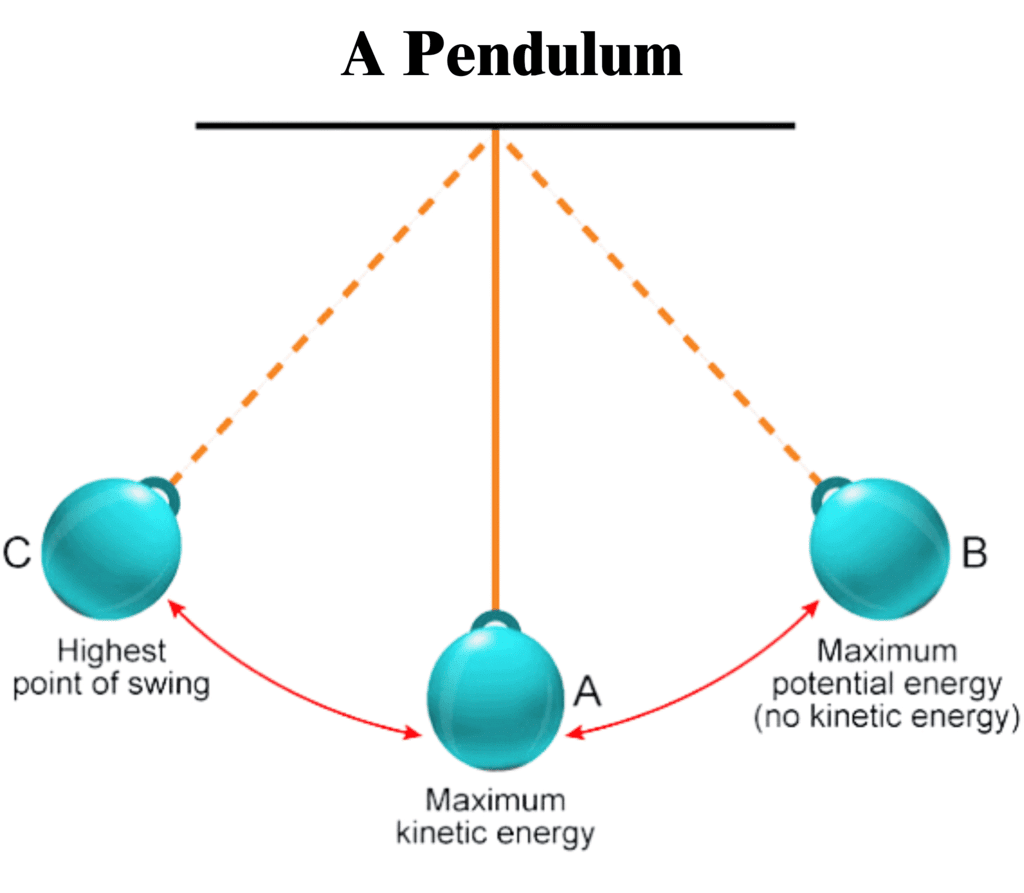
Positions of the Pendulum

• At the highest point (A): Here, the pendulum is briefly at rest and all its energy is potential energy (PE). The height (h) of the mass above the lowest point determines how much potential energy it has. The potential energy can be calculated using the formula: PE = m · g · h, where g is the acceleration due to gravity.
• At the lowest point (B): As the pendulum swings down, its potential energy is changed into kinetic energy (KE). At this point, its height (h) is zero, meaning it has no potential energy. Here, all its energy is kinetic energy, and the pendulum is moving at its highest speed. The kinetic energy can be calculated using the formula: KE = ½ m · v², where v is the velocity of the mass.
• At the highest point on the other side (C): As the pendulum swings upwards, its kinetic energy is transformed back into potential energy.

The total mechanical energy of the pendulum, which is the sum of kinetic energy and potential energy, stays the same throughout its motion. This shows the law of conservation of energy, which states that energy cannot be created or destroyed; it can only be changed from one form to another.

In essence, the energy changes in a pendulum illustrate that the total mechanical energy remains constant, confirming that the total energy before and after the change is unchanged.

Take a Practice Test Test yourself on topics from Class 9 exam

Practice Now

Practice Now

Rate of Doing Work or Power

The rate at which work is done or energy is transferred is known as Power. Power indicates how quickly or slowly work is performed. The formula for calculating power is:

Power = Work done / Time taken

• The SI unit of power is a watt (W). One watt is defined as the power of an agent that does work at the rate of 1 joule per second (1 W = 1 J/s).
• We use larger units for energy transfer, such as kilowatts (kW): 1 kilowatt = 1 kW = 1000 watts.
• Other common units of power include:
  • 1 megawatt (MW) = 10⁶ watts
  • 1 horsepower (hp) = 746 watts

Average Power

Understanding average power is important because sometimes an agent (like a machine or a person) works at different speeds. Average power helps us understand the overall power used. It is calculated by dividing the total energy used by the total time taken. This gives a single number that represents the overall power, even if the rate of work changed.

• Average Power = Total energy consumed (or total work done) / Total time
• The power of an agent may vary over time, meaning they might do work at different rates during different intervals.

According to the law of conservation of energy, energy can only change forms; it cannot be created or destroyed. The total energy before and after any change always remains constant. Energy exists in various forms in nature, such as kinetic energy, potential energy, heat energy, and chemical energy. The total of kinetic and potential energies in an object is referred to as its mechanical energy.

What you have learnt

1. Work:
Work is defined as the force applied to an object multiplied by the distance the object moves in the direction of the force.

• Formula: Work = Force × Distance
• Unit: The unit of work is the joule (J), where 1 joule = 1 newton × 1 metre.
• If an object does not move, the work done is zero.

2. Energy:
Energy is the capacity to do work and is measured in the same unit as work, the joule (J).

• Kinetic Energy (KE):
  An object in motion possesses kinetic energy. For an object of mass $mm$ moving with velocity $\mathrm{<br\; style="word-break:\; break-word;\; line-height:\; 1.8;\; font-family:\; Lato,\; sans-serif;\; font-size:\; 20px;\; letter-spacing:\; inherit;\; color:\; black;">}$$v$v:$KE=\frac{1}{2}mv\mathrm{<sup\; style="word-break:\; break-word;\; line-height:\; 1.8;\; font-family:\; Lato,\; sans-serif;\; font-size:\; 13px\; !important;\; letter-spacing:\; inherit;\; color:\; black">2</sup>}KE\; =\; \backslash frac\{1\}\{2\}\; mv^2$
• Potential Energy (PE):
  Energy due to an object's position or shape is called potential energy.
  For an object of mass $mm$m raised to a height PE= mgh where $\mathrm{<br\; style="word-break:\; break-word;\; line-height:\; 1.8;\; font-family:\; Lato,\; sans-serif;\; font-size:\; 20px;\; letter-spacing:\; inherit;\; color:\; black;">}g$g is the acceleration due to gravity.

3. Law of Conservation of Energy:
Energy cannot be created or destroyed but can transform from one form to another. The total energy in a system remains constant before and after any transformation.

4. Forms of Energy:
Energy exists in various forms, including:

• Kinetic energy
• Potential energy
• Heat energy
• Chemical energy

5. Mechanical Energy:
The sum of an object's kinetic and potential energy is called its mechanical energy.

6. Power:
Power is the rate at which work is done.
Formula: Power = Work ÷ Time

Unit: The SI unit of power is the watt (W), where 1 watt = 1 joule per second (1 W = 1 J/s).