Class 9 Maths Chapter 2 Question Answers - Polynomials
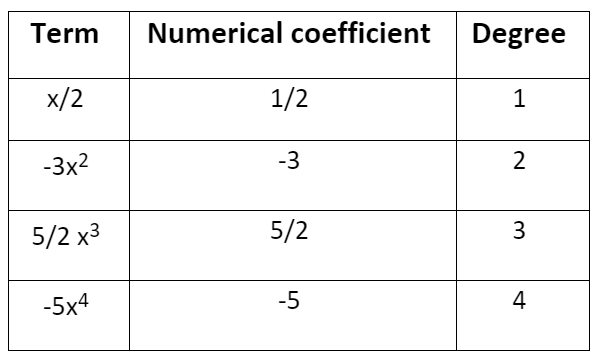
Q1. Write the numerical co-efficient and degree of each term of: x2 − 3x2 + 52 x3 − 5x4
Sol:
Q2. Write the coefficient of x2 in each of the following:
(i) 9 – 12x + x3
(ii) ∏/6 x2 – 3x + 4
(iii) √3x – 7
Sol:
(i) 9 – 12x + x3
Coefficient of x2 =0
(ii) ∏/6 x2 – 3x + 4
Coefficient of x2 = ∏/6
(iii) √3x – 7
Coefficient of x2 = 0
Q3. Factorise z2 – 4z –12
Sol: To factorize this expression, we need to find two numbers α and β such that α + β = -4 and αβ = -12
z2 – 6z + 2z – 12
z(z – 6) + 2(z – 6)
(z + 2)(z – 6)
Q4. (a) For what value of k, the polynomial x2 + (4 – k)x + 2 is divisible by x – 2?
(b) For what value of ‘m’ is x3 – 2mx2 + 16 is divisible by (x + 2)?
Sol: (a) Here p(x) = x2 + 4x – kx + 2
If p(x) is exactly divisible by x – 2, then p(2) = 0
i.e. (2)2 + 4(2) – k(2) + 2 = 0
⇒ 4 + 8 – 2k + 2 = 0
⇒ 14 – 2k = 0
⇒ 2k = 14
⇒ k= 14/2 = 7
Thus, the required value of k is 7.(b) Here, p(x) = x3 – 2mx2 + 16
∴ p(–2) = (–2)3 –2(–2)2m + 16
⇒ –8 –8m + 16
⇒ –8m + 8
Since, p(x) is divisible by x + 2
∴ p(–2) = 0
or –8m + 8 = 0
⇒ m = 1
Q5. Factorize x2 – x – 12.
Sol: We have x2 – x – 12
⇒ x2 – 4x + 3x – 12
⇒ x(x – 4) + 3(x – 4)
⇒ (x – 4)(x + 3)
Thus, x2 – x – 12 = (x – 4)(x + 3)
Q6.Verify whether the indicated numbers are zeros of the polynomials corresponding to them in the following cases:
(i) f(x) = 3x + 1, x = −1/3
(ii) f(x) = x2 – 1, x = 1,−1
Sol: (i) f(x) = 3x + 1, x = −1/3f(x) = 3x + 1
Substitute x = −1/3 in f(x)
f( −1/3) = 3(−1/3) + 1
= -1 + 1
= 0
Since, the result is 0, so x = −1/3 is the root of 3x + 1(ii) f(x) = x2 – 1, x = 1,−1
f(x) = x2 – 1
Given that x = (1 , -1)
Substitute x = 1 in f(x)
f(1) = 12 – 1
= 1 – 1
= 0
Now, substitute x = (-1) in f(x)
f(-1) = (−1)2 – 1
= 1 – 1
= 0
Since , the results when x = 1 and x = -1 are 0, so (1 , -1) are the roots of the polynomial f(x) = x2 – 1
Q7. Check whether (x – 1) is a factor of the polynomial x3 – 27x2 + 8x + 18.
Sol: Here, p(x) = x3 – 27x2 + 8x + 18, (x – 1) will be a factor of p(x) only if (x – 1) divides p(x) leaving a remainder 0.
For x – 1 = 0
⇒ x = 1
∴ p(1) = (1)3 – 27(1)2 + 8(1) + 18
⇒ 1 – 27 + 8 + 18
⇒ 27 – 27
⇒ 0
Since, p(1) = 0
∴ (x – 1) is a factor of p(x).
Thus, (x – 1) is a factor of x3 – 27x2 + 8x + 18.
Q8.Evaluate each of the following using identities:
(i) (2x – 1/x)2
(ii) (2x + y) (2x – y)
Sol:
(i) (2x – 1/x)2
[Use identity: (a – b)2 = a2 + b2 – 2ab ]
(2x – 1/x)2 = (2x)2 + (1/x)2 – 2 (2x)(1/x)
= 4x2 + 1/x2 – 4
(ii) (2x + y) (2x – y)
[Use identity: (a – b)(a + b) = a2 – b2 ]
(2x + y) (2x – y) = (2x )2 – (y)2
= 4x2 – y2
Q9. Find the value of k, if (x – k) is a factor of x6 – kx5 + x4 – kx3 + 3x – k + 4.
Sol: Here, p(x) = x6 – kx5 + x4 – kx3 + 3x – k + 4
If (x – k) is a factor of p(x), then p(k) = 0
i.e (k)6 – k(k5) + k4 – k(k3) + 3k – k + 4 = 0
⇒ k6 – k6 + k4 – k4 + 3k – k + 4 = 0
⇒ 2k + 4 = 0
⇒ 2k = – 4
⇒ k = (-4/2) = –2
Thus, the required value of k is –2.
Q10. Factorize: 9a2 – 9b2 + 6a + 1
Sol: 9a2 – 9b2 + 6a + 1
⇒ [9a2 + 6a + 1] – 9b2
⇒ [(3a)2 + 2(3a)(1) + (1)2] – (3b)2
⇒ (3a + 1)2 – (3b)2
⇒ [(3a + 1) + 3b][(3a + 1) – 3b] {using x2 – y2 = (x – y)(x + y)}
⇒ (3a + 1 + 3b)(3a + 1 – 3b)
|
40 videos|566 docs|57 tests
|
FAQs on Class 9 Maths Chapter 2 Question Answers - Polynomials
| 1. What is a polynomial? |  |
| 2. How do you classify polynomials based on the number of terms? |  |
| 3. What are the degrees of polynomials? |  |
| 4. How can you add or subtract polynomials? |  |
| 5. What is the significance of the roots of a polynomial? |  |