CUET Commerce Exam > CUET Commerce Notes > General Test Preparation for CUET > Concept & Solved Questions: Venn Diagram
Concept & Solved Questions: Venn Diagram | General Test Preparation for CUET - CUET Commerce PDF Download
Learn the methods and shortcuts to deal with the questions on Venn Diagrams.
What is a Venn Diagram?
Venn diagram, also known as Euler-Venn diagram is a simple representation of sets by diagrams. The usual depiction makes use of a rectangle as the universal set and circles for the sets under consideration.
In CAT and other MBA entrance exams, questions asked from this topic involve 2 or 3 variable only. Therefore, in this article we are going to discuss problems related to 2 and 3 variables.
Let's take a look at some basic formulas for Venn diagrams of two and three elements
What is a Venn Diagram?
Venn diagram, also known as Euler-Venn diagram is a simple representation of sets by diagrams. The usual depiction makes use of a rectangle as the universal set and circles for the sets under consideration.
In CAT and other MBA entrance exams, questions asked from this topic involve 2 or 3 variable only. Therefore, in this article we are going to discuss problems related to 2 and 3 variables.
Let's take a look at some basic formulas for Venn diagrams of two and three elements
n ( A ∪ B) = n(A) + n (B) - n (A ∩ B)
n (A ∪ B ∪ C) = n(A) + n (B) + n (C) - n (A ∩ B) - n (B ∩ C) - n (C ∩ A) + n (A ∩ B ∩ C)
And so on, where n(A) = number of elements in set A.
Once you understand the concept of Venn diagram with the help of diagrams, you don’t have to memorize these formulas.
Venn Diagram in case of two elements
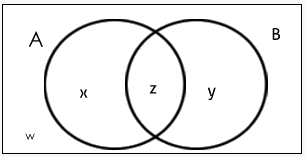
Where;
X = number of elements that belong to set A only
Y = number of elements that belong to set B only
Z = number of elements that belong to set A and B both (AB)
W = number of elements that belong to none of the sets A or B
From the above figure, it is clear that
n(A) = x + z ;
n (B) = y + z ;
n(A ∩ B) = z ;
n (A ∪ B) = x +y+ z.
Total number of elements = x + y + z + w
Venn Diagram in case of three elements
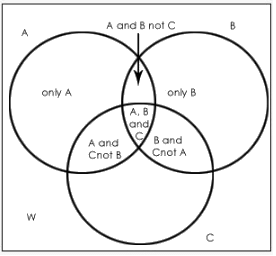
Where,
W = number of elements that belong to none of the sets A, B or C
Tip: Always start filling values in the Venn diagram from the innermost value.
Solved Examples:
Example 1: In a college, 200 students are randomly selected. 140 like tea, 120 like coffee and 80 like both tea and coffee.
• How many students like only tea?
• How many students like only coffee?
• How many students like neither tea nor coffee?
• How many students like only one of tea or coffee?
• How many students like at least one of the beverages?
Solution: The given information may be represented by the following Venn diagram, where T = tea and C = coffee.
• Number of students who like only tea = 60• Number of students who like only coffee = 40
• Number of students who like neither tea nor coffee = 20
• Number of students who like only one of tea or coffee = 60 + 40 = 100
• Number of students who like at least one of tea or coffee = n (only Tea) + n (only coffee) + n (both Tea & coffee) = 60 + 40 + 80 = 180
Example 2: In a survey of 500 students of a college, it was found that 49% liked watching football, 53% liked watching hockey and 62% liked watching basketball. Also, 27% liked watching football and hockey both, 29% liked watching basketball and hockey both and 28% liked watching football and basket ball both. 5% liked watching none of these games.
• How many students like watching all the three games?
• Find the ratio of number of students who like watching only football to those who like watching only hockey.
• Find the number of students who like watching only one of the three given games.
• Find the number of students who like watching at least two of the given games.
Solution:
n(F) = percentage of students who like watching football = 49%
n(H) = percentage of students who like watching hockey = 53%
n(B)= percentage of students who like watching basketball = 62%
Solved Examples:
Example 1: In a college, 200 students are randomly selected. 140 like tea, 120 like coffee and 80 like both tea and coffee.
• How many students like only tea?
• How many students like only coffee?
• How many students like neither tea nor coffee?
• How many students like only one of tea or coffee?
• How many students like at least one of the beverages?
Solution: The given information may be represented by the following Venn diagram, where T = tea and C = coffee.
• Number of students who like only tea = 60• Number of students who like only coffee = 40• Number of students who like neither tea nor coffee = 20
• Number of students who like only one of tea or coffee = 60 + 40 = 100
• Number of students who like at least one of tea or coffee = n (only Tea) + n (only coffee) + n (both Tea & coffee) = 60 + 40 + 80 = 180
Example 2: In a survey of 500 students of a college, it was found that 49% liked watching football, 53% liked watching hockey and 62% liked watching basketball. Also, 27% liked watching football and hockey both, 29% liked watching basketball and hockey both and 28% liked watching football and basket ball both. 5% liked watching none of these games.
• How many students like watching all the three games?
• Find the ratio of number of students who like watching only football to those who like watching only hockey.
• Find the number of students who like watching only one of the three given games.
• Find the number of students who like watching at least two of the given games.
Solution:
n(F) = percentage of students who like watching football = 49%
n(H) = percentage of students who like watching hockey = 53%
n(B)= percentage of students who like watching basketball = 62%
n ( F ∩ H) = 27% ; n (B ∩ H) = 29% ; n(F ∩ B) = 28%
Since 5% like watching none of the given games so, n (F ∪ H ∪ B) = 95%.
Now applying the basic formula,
95% = 49% + 53% + 62% -27% - 29% - 28% + n (F ∩ H ∩ B)
Solving, you get n (F ∩ H ∩ B) = 15%.
Now, make the Venn diagram as per the information given.
Note: All values in the Venn diagram are in percentage.
Number of students who like watching all the three games = 15 % of 500 = 75.
Ratio of the number of students who like only football to those who like only hockey = (9% of 500)/(12% of 500) = 9/12 = 3:4.
The number of students who like watching only one of the three given games = (9% + 12% + 20%) of 500 = 205
The number of students who like watching at least two of the given games=(number of students who like watching only two of the games) +(number of students who like watching all the three games)= (12 + 13 + 14 + 15)% i.e. 54% of 500 = 270.
To know the importance of this topic, check out some previous year CAT questions from this topic:
CAT 2017 Solved Questions:
DIRECTIONS for the question: Read the information given below and answer the question that follows.
Applicants for the doctoral programmes of Ambi Institute of Engineering (AIE) and Bambi Institute of Engineering (BIE) have to appear for a Common Entrance Test (CET). The test has three sections: Physics (P), Chemistry (C), and Maths (M). Among those appearing for CET, those at or above the 80th percentile in at least two sections, and at or above the 90th percentile overall, are selected for Advanced Entrance Test (AET) conducted by AIE. AET is used by AIE for final selection. For the 200 candidates who are at or above the 90th percentile overall based on CET, the following are known about their performance in CET:
1. No one is below the 80th percentile in all 3 sections.
2. 150 are at or above the 80th percentile in exactly two sections.
3. The number of candidates at or above the 80th percentile only in P is the same as the number of candidates at or above the 80th percentile only in C. The same is the number of candidates at or above the 80th percentile only in M.
4. Number of candidates below 80th percentile in P: Number of candidates below 80th percentile in C: Number of candidates below 80th percentile in M = 4:2:1.
BIE uses a different process for selection. If any candidate is appearing in the AET by AIE, BIE considers their AET score for final selection provided the candidate is at or above the 80th percentile in P. Any other candidate at or above the 80th percentile in P in CET, but who is not eligible for the AET, is required to appear in a separate test to be conducted by BIE for being considered for final selection. Altogether, there are 400 candidates this year who are at or above the 80th percentile in P.
Question 1: What best can be concluded about the number of candidates sitting for the separate test for BIE who were at or above the 90th percentile overall in CET?
Answer: The number of candidates sitting for separate test for BIE who were at or above 90th percentile in CET (a) is either 3 or 10.
Question 2: If the number of candidates who are at or above the 90th percentile overall and also at or above the 80th percentile in all three sections in CET is actually a multiple of 5, what is the number of candidates who are at or above the 90th percentile overall and at or above the 80th percentile in both P and M in CET?
Answer: From the given condition, g is a multiple of 5. Hence, g = 20. The number of candidates at or above 90th percentile overall and at or above 80th percentile in both P and M = e + g = 60.
Since 5% like watching none of the given games so, n (F ∪ H ∪ B) = 95%.
Now applying the basic formula,
95% = 49% + 53% + 62% -27% - 29% - 28% + n (F ∩ H ∩ B)
Solving, you get n (F ∩ H ∩ B) = 15%.
Now, make the Venn diagram as per the information given.
Note: All values in the Venn diagram are in percentage.
Number of students who like watching all the three games = 15 % of 500 = 75.
Ratio of the number of students who like only football to those who like only hockey = (9% of 500)/(12% of 500) = 9/12 = 3:4.
The number of students who like watching only one of the three given games = (9% + 12% + 20%) of 500 = 205
The number of students who like watching at least two of the given games=(number of students who like watching only two of the games) +(number of students who like watching all the three games)= (12 + 13 + 14 + 15)% i.e. 54% of 500 = 270.
To know the importance of this topic, check out some previous year CAT questions from this topic:
CAT 2017 Solved Questions:
DIRECTIONS for the question: Read the information given below and answer the question that follows.
Applicants for the doctoral programmes of Ambi Institute of Engineering (AIE) and Bambi Institute of Engineering (BIE) have to appear for a Common Entrance Test (CET). The test has three sections: Physics (P), Chemistry (C), and Maths (M). Among those appearing for CET, those at or above the 80th percentile in at least two sections, and at or above the 90th percentile overall, are selected for Advanced Entrance Test (AET) conducted by AIE. AET is used by AIE for final selection. For the 200 candidates who are at or above the 90th percentile overall based on CET, the following are known about their performance in CET:
1. No one is below the 80th percentile in all 3 sections.
2. 150 are at or above the 80th percentile in exactly two sections.
3. The number of candidates at or above the 80th percentile only in P is the same as the number of candidates at or above the 80th percentile only in C. The same is the number of candidates at or above the 80th percentile only in M.
4. Number of candidates below 80th percentile in P: Number of candidates below 80th percentile in C: Number of candidates below 80th percentile in M = 4:2:1.
BIE uses a different process for selection. If any candidate is appearing in the AET by AIE, BIE considers their AET score for final selection provided the candidate is at or above the 80th percentile in P. Any other candidate at or above the 80th percentile in P in CET, but who is not eligible for the AET, is required to appear in a separate test to be conducted by BIE for being considered for final selection. Altogether, there are 400 candidates this year who are at or above the 80th percentile in P.
Question 1: What best can be concluded about the number of candidates sitting for the separate test for BIE who were at or above the 90th percentile overall in CET?
Answer: The number of candidates sitting for separate test for BIE who were at or above 90th percentile in CET (a) is either 3 or 10.
Question 2: If the number of candidates who are at or above the 90th percentile overall and also at or above the 80th percentile in all three sections in CET is actually a multiple of 5, what is the number of candidates who are at or above the 90th percentile overall and at or above the 80th percentile in both P and M in CET?
Answer: From the given condition, g is a multiple of 5. Hence, g = 20. The number of candidates at or above 90th percentile overall and at or above 80th percentile in both P and M = e + g = 60.
Question 3: If the number of candidates who are at or above the 90th percentile overall and also at or above the 80th percentile in all three sections in CET is actually a multiple of 5, then how many candidates were shortlisted for the AET for AIE?
Answer: In this case, g = 20. Number of candidates shortlisted for AET = d + e + f + g = 10 + 40 + 100 + 20 = 170
Question 4: If the number of candidates who are at or above the 90th percentile overall and also are at or above the 80th percentile in P in CET, is more than 100, how many candidates had to sit for the separate test for BIE?
Answer: From the given condition, the number of candidates at or above 90th percentile overall and at or above 80th percentile in P in CET = 104. The number of candidates who have to sit for separate test = 296 + 3 = 299.
Another type of questions asked from this topic is based on maxima and minima. We have discussed this type in the other article.
It is given that 200 candidates scored above 90th percentile overall in CET. Let the following Venn diagram represent the number of persons who scored above 80 percentile in CET in each of the three sections:
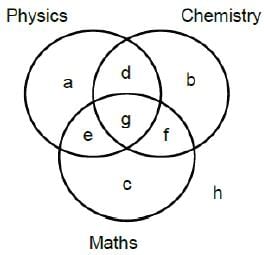
From 1, h = 0.
From 2, d + e + f = 150
From 3, a = b = c
Since there are a total of 200 candidates, 3a + g = 200 – 150 = 50
From 4, (2a + f) : (2a + e) : (2a + d) = 4 : 2 : 1
Therefore, 6a + (d + e + f) is divisible by 4 + 2 + 1 = 7.
Since d + e + f = 150, 6a + 150 is divisible by 7, i.e., 6a + 3 is divisible by 7. Hence, a = 3, 10, 17, . . .
Further, since 3a + g = 5 0, a must be less than 17. Therefore, only two cases are possible for the value of a, i.e., 3 or 10.
We can calculate the values of the other variables for the two cases.
a = 3 or 10
d = 18 or 10
e = 42 or 40
f = 90 or 100
g = 41 or 20
Among the candidates who are at or above 90th percentile, the candidates who are at or above 80th percentile in at least two sections are selected for AET. Hence, the candidates represented by d, e, f and g are selected for AET. BIE will consider the candidates who are appearing for AET and are at or above 80th percentile in P. Hence, BIE will consider the candidates represented by d, e and g, which can be 104 or 80. BIE will conduct a separate test for the other students who are at or above 80th percentile in P. Given that there are a total of 400 candidates at or above 80th percentile in P, and since there are 104 or 80 candidates at or above 80th percentile in P and are at or above 90th percentile overall, there must be 296 or 320 candidates at or above 80th percentile in P who scored less than 90th percentile overall.
Key Learning:
• It is important to carefully list the conditions given in the question in the form of a Venn diagram.
• While solving such questions, avoid taking many variables.
• Try solving the questions using the Venn diagram approach and not with the help of formulae.
The document Concept & Solved Questions: Venn Diagram | General Test Preparation for CUET - CUET Commerce is a part of the CUET Commerce Course General Test Preparation for CUET.
All you need of CUET Commerce at this link: CUET Commerce
|
154 videos|396 docs|723 tests
|
FAQs on Concept & Solved Questions: Venn Diagram - General Test Preparation for CUET - CUET Commerce
| 1. What is a Venn diagram? |  |
Ans. A Venn diagram is a graphical representation of sets, showing their relationships through overlapping circles or other shapes. It is used to visually illustrate the similarities and differences between different sets or groups of objects or concepts.
| 2. How do you create a Venn diagram? | |
Ans. To create a Venn diagram, you start by drawing overlapping circles or other shapes that represent the sets you want to compare. Then, you place the elements or concepts that belong to each set inside the corresponding circle or shape. The overlapping region represents the elements or concepts that are common to both sets.
| 3. What is the purpose of a Venn diagram? | |
Ans. The purpose of a Venn diagram is to help visualize and analyze the relationships between different sets or groups. It allows you to identify the similarities and differences between sets, understand the overlap between them, and determine the elements or concepts that belong exclusively to each set or the intersection of multiple sets.
| 4. Can Venn diagrams be used for complex data analysis? | |
Ans. Yes, Venn diagrams can be used for complex data analysis. They are particularly useful when dealing with multiple sets or groups and can provide insights into the relationships and interactions between them. By using advanced techniques and incorporating more sets or layers, Venn diagrams can handle intricate data analysis and visualization tasks.
| 5. How can Venn diagrams aid in problem-solving? | |
Ans. Venn diagrams aid in problem-solving by helping to organize and analyze information. They can be used to identify commonalities, differences, and relationships between sets, which can guide decision-making and problem-solving processes. By visually representing the data, Venn diagrams provide a visual tool that assists in understanding the problem and finding solutions.
Related Exams
About this Document
|
4.4K Views |
|
4.74/5 Rating |
|
Nov 21, 2024 Last updated |
Document Description: Concept & Solved Questions: Venn Diagram for CUET Commerce 2024 is part of General Test Preparation for CUET preparation.
The notes and questions for Concept & Solved Questions: Venn Diagram have been prepared according to the CUET Commerce exam syllabus. Information about Concept & Solved Questions: Venn Diagram covers topics
like and Concept & Solved Questions: Venn Diagram Example, for CUET Commerce 2024 Exam. Find important definitions, questions, notes, meanings, examples, exercises and tests below for Concept & Solved Questions: Venn Diagram.
Introduction of Concept & Solved Questions: Venn Diagram in English is available as part of our General Test Preparation for CUET
for CUET Commerce & Concept & Solved Questions: Venn Diagram in Hindi for General Test Preparation for CUET course.
Download more important topics related with notes, lectures and mock test series for CUET Commerce
Exam by signing up for free. CUET Commerce: Concept & Solved Questions: Venn Diagram | General Test Preparation for CUET - CUET Commerce
Description
Full syllabus notes, lecture & questions for Concept & Solved Questions: Venn Diagram | General Test Preparation for CUET - CUET Commerce - CUET Commerce | Plus excerises question with solution to help you revise complete syllabus for General Test Preparation for CUET | Best notes, free PDF download
Information about Concept & Solved Questions: Venn Diagram
In this doc you can find the meaning of Concept & Solved Questions: Venn Diagram defined & explained in the simplest way possible. Besides explaining types of
Concept & Solved Questions: Venn Diagram theory, EduRev gives you an ample number of questions to practice Concept & Solved Questions: Venn Diagram tests, examples and also practice CUET Commerce
tests
|
154 videos|396 docs|723 tests
|
Download as PDF
|
Explore Courses for CUET Commerce exam
|
|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
Related Searches
Concept & Solved Questions: Venn Diagram | General Test Preparation for CUET - CUET Commerce
,Free
,Important questions
,shortcuts and tricks
,video lectures
,Extra Questions
,Sample Paper
,Concept & Solved Questions: Venn Diagram | General Test Preparation for CUET - CUET Commerce
,mock tests for examination
,ppt
,past year papers
,Concept & Solved Questions: Venn Diagram | General Test Preparation for CUET - CUET Commerce
,Semester Notes
,Previous Year Questions with Solutions
,Viva Questions
,study material
,Summary
,Objective type Questions
,practice quizzes
,MCQs
,Exam
;
Additional Information about Concept & Solved Questions: Venn Diagram for CUET Commerce Preparation
Concept & Solved Questions: Venn Diagram Free PDF Download
The Concept & Solved Questions: Venn Diagram is an invaluable resource that delves deep into the core of the CUET Commerce exam.
These study notes are curated by experts and cover all the essential topics and concepts, making your preparation more efficient and effective.
With the help of these notes, you can grasp complex subjects quickly, revise important points easily,
and reinforce your understanding of key concepts. The study notes are presented in a concise and easy-to-understand manner,
allowing you to optimize your learning process. Whether you're looking for best-recommended books, sample papers, study material,
or toppers' notes, this PDF has got you covered. Download the Concept & Solved Questions: Venn Diagram now and kickstart your journey towards success in the CUET Commerce exam.
Importance of Concept & Solved Questions: Venn Diagram
The importance of Concept & Solved Questions: Venn Diagram cannot be overstated, especially for CUET Commerce aspirants.
This document holds the key to success in the CUET Commerce exam.
It offers a detailed understanding of the concept, providing invaluable insights into the topic.
By knowing the concepts well in advance, students can plan their preparation effectively.
Utilize this indispensable guide for a well-rounded preparation and achieve your desired results.
Concept & Solved Questions: Venn Diagram Notes
Concept & Solved Questions: Venn Diagram Notes offer in-depth insights into the specific topic to help you master it with ease.
This comprehensive document covers all aspects related to Concept & Solved Questions: Venn Diagram.
It includes detailed information about the exam syllabus, recommended books, and study materials for a well-rounded preparation.
Practice papers and question papers enable you to assess your progress effectively.
Additionally, the paper analysis provides valuable tips for tackling the exam strategically.
Access to Toppers' notes gives you an edge in understanding complex concepts.
Whether you're a beginner or aiming for advanced proficiency, Concept & Solved Questions: Venn Diagram Notes on EduRev are your ultimate resource for success.
Concept & Solved Questions: Venn Diagram CUET Commerce
The "Concept & Solved Questions: Venn Diagram CUET Commerce Questions" guide is a valuable resource for all aspiring students preparing for the
CUET Commerce exam. It focuses on providing a wide range of practice questions to help students gauge
their understanding of the exam topics. These questions cover the entire syllabus, ensuring comprehensive preparation.
The guide includes previous years' question papers for students to familiarize themselves with the exam's format and difficulty level.
Additionally, it offers subject-specific question banks, allowing students to focus on weak areas and improve their performance.
Study Concept & Solved Questions: Venn Diagram on the App
Students of CUET Commerce can study Concept & Solved Questions: Venn Diagram alongwith tests & analysis from the EduRev app,
which will help them while preparing for their exam. Apart from the Concept & Solved Questions: Venn Diagram,
students can also utilize the EduRev App for other study materials such as previous year question papers, syllabus, important questions, etc.
The EduRev App will make your learning easier as you can access it from anywhere you want.
The content of Concept & Solved Questions: Venn Diagram is prepared as per the latest CUET Commerce syllabus.
|
© EduRev
|
Education Revolution
|
Follow Us
|
Signup on EduRev and stay on top of your study goals
10M+ students crushing their study goals daily