CAT Exam > CAT Notes > Quantitative Aptitude (Quant) > Concepts with Solved Examples: Trigonometry
Concepts with Solved Examples: Trigonometry | Quantitative Aptitude (Quant) - CAT PDF Download
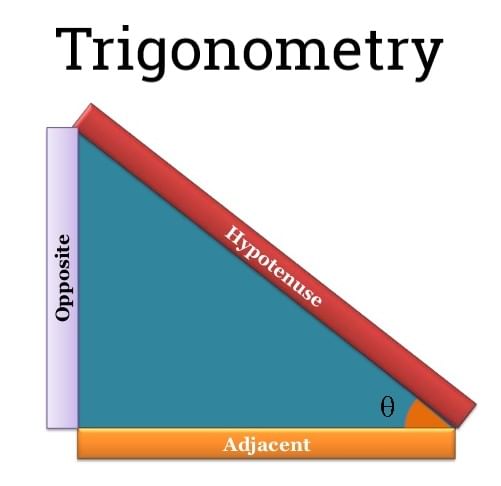
Angles and their Relationship
- Angles are measured in many units’ viz. degrees, minutes, seconds, radians, gradients.
- Where 1 degree = 60 minutes, 1 minute = 60 seconds,
π radians = 180° = 200g
⇒ 1 radian = 180°/π and 1 degree = π/ 180 radians.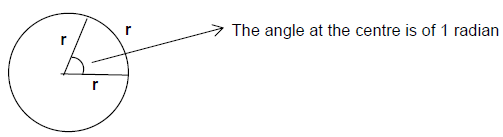
Basic Trigonometric Ratios
- In a right triangle ABC, if θ be the angle between AC & BC.
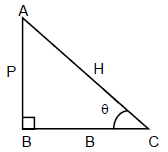
- If θ is one of the angle other then right angle, then the side opposite to the angle is perpendicular (P) and the sides containing the angle are taken as Base ( B) and the hypotenuse (H). In this type of triangles, we can have six types of ratios. These ratios are called trigonometric ratios.
Important Formulae

Range of Values of Ratios
If 0 ≤ θ ≤ 360o, then the values for different trigonometric ratios will be as follows:
- - 1 ≤ Sin θ ≤ 1
- - 1 ≤ cos θ ≤ 1
- - ∞ ≤ tan θ ≤ ∞
- - ∞ ≤ cot θ ≤ ∞
- - ∞ ≤ Sec θ ≤ -1 & 1 ≤ Sec θ ≤ ∞
- - ∞ ≤ Cosec θ ≤ - 1 & 1 ≤ Cosec θ ≤ ∞
Sign of Trigonometric Ratios
We divide the angle at a point (i.e. 360°) into 4 parts called quadrants. In the first quadrant all the trigonometric ratios are positive.
Important Results
Example.1 Simplify.
Sol.
Example.2 If cot A , find the value of 3 cos A + 4 sin A, where A is in the first quadrant.
Sol.
Example.3 Find the value of
Sol.
= 1 + 1 - 1 = 1
Table: Values of trigonometric Ratio for some special angles.
Properties of Triangle
➢ Sine Rule
- In any triangle ABC if AB, BC, AC be represented by c, a, b respectively
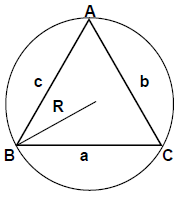
➢ Cosine Rule
- In a triangle ABC of having sides of any size, we have the following rule:
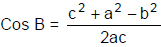
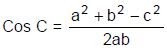
➢ Area of Triangle
The document Concepts with Solved Examples: Trigonometry | Quantitative Aptitude (Quant) - CAT is a part of the CAT Course Quantitative Aptitude (Quant).
All you need of CAT at this link: CAT
|
167 videos|229 docs|95 tests
|
FAQs on Concepts with Solved Examples: Trigonometry - Quantitative Aptitude (Quant) - CAT
| 1. What are the basic trigonometric ratios? |  |
Ans. The basic trigonometric ratios are sine, cosine, and tangent. They are defined as follows:
- Sine (sin): The ratio of the length of the side opposite the angle to the length of the hypotenuse.
- Cosine (cos): The ratio of the length of the side adjacent to the angle to the length of the hypotenuse.
- Tangent (tan): The ratio of the length of the side opposite the angle to the length of the side adjacent to the angle.
| 2. How are angles and their relationship important in trigonometry? |  |
Ans. In trigonometry, angles are fundamental as they determine the values of trigonometric ratios. The relationship between angles and their trigonometric ratios allows us to solve various problems involving triangles and other geometric shapes. By understanding the properties and relationships of angles, we can calculate lengths, areas, and other measurements in real-world situations.
| 3. What are the properties of triangles in trigonometry? |  |
Ans. In trigonometry, some important properties of triangles include:
- The sum of the interior angles of a triangle is always 180 degrees.
- The length of one side of a triangle is always less than the sum of the lengths of the other two sides.
- The longest side of a triangle is opposite the largest angle, and the shortest side is opposite the smallest angle.
- The ratio of the lengths of the sides of a right triangle follows the Pythagorean theorem.
| 4. Can you provide an example of a trigonometry problem involving angles and their relationship? |  |
Ans. Sure! Here's an example:
In a right triangle, the length of one side is 5 cm, and the length of the hypotenuse is 13 cm. Find the measure of the acute angle opposite the side with length 5 cm.
Solution:
Using the cosine ratio, we have cos(angle) = adjacent/hypotenuse.
cos(angle) = 5/13
angle = arccos(5/13)
Using a calculator, we find the angle to be approximately 67.38 degrees.
| 5. How do trigonometric ratios help in real-world applications? |  |
Ans. Trigonometric ratios have numerous real-world applications, such as in engineering, physics, architecture, and navigation. They are used to solve problems involving heights and distances, angles of elevation and depression, projectile motion, electrical circuits, and more. For example, trigonometry helps engineers design bridges and buildings, astronomers measure distances between celestial objects, and pilots navigate aircraft using angles and distances.
Related Searches






















