Definite Integral As Limit Of A Sum And Estimate Of Definite Integrals | Mathematics (Maths) for JEE Main & Advanced PDF Download
Definite Integral As Limit Of A Sum
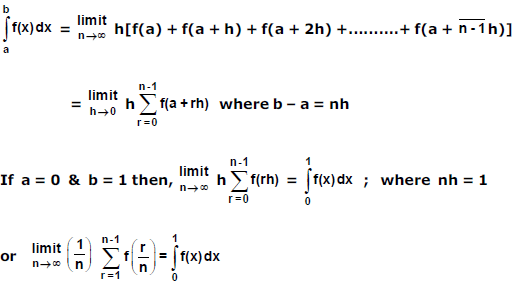
Remark :
The symbol
was introduced by Leibnitz and is called integral sign. It is an elongated S and was chosen because an integral is a limit of sums. In the notation
is called the integrand and a and b are called the limits of integration; a is the lower limit and b is the upper limit. The symbol dx has no official meaning by itself;
is all one symbol. The procedure of calculating an integral is called integration.
Estimate Of Definite Integration & General Inequality
STATEMENT : If f is continuous on the interval [a, b], there is atleast one number c between a and b such that
Proof : Suppose M and m are the largest and smallest values of f, respectively, on [a, b]. This means m ≤ f(x) ≤ M when a ≤ x ≤ b
Because f is continuous on the closed interval [a, b] and because the number I = lies between m and M, the intermediate value theorem syas there exists a number c between a and b for which f(c) = I ; that is,
The mean value theorem for integrals does not specify how to determine c. It simply guarantees the existence of atleast one number c in the interval.
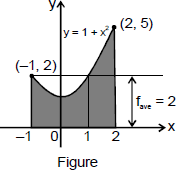
Since f(x) = 1 + x2 is continuous on the interval [–1, 2], the Mean Value Theorem for Integrals says there is a number c in [–1, 2] such that
In this particular case we can find c explicitly. From previous Example we know that fave = 2, so the value of c satisfies f(c) = = 2
Thus, in this case there happen to be two numbers c = ± 1 in the interval [–1, 2] that work in the mean value theorem for Integrals.
Walli's Formula & Reduction Formula
Solved Examples
Ex.1 Evaluate using limit of sum.
Sol.
This integral can't be interpreated as an area because f takes on both positive and negative values. But is can be interpreated as the difference of areas A1 – A2, where A1 and A2 are shown in Figure
Ex.2 Prove that, Hence or otherwise evaluate
Sol.
Consider sin nθ + sin (n - 2) θ = 2 sin (n - 1) θ cos θ ⇒ sin nθ sec θ = 2 sin (n-1) θ - sin (n - 2) θ sec θ
Hence
,
Ex.3 Prove that
Sol.
L.H.S. =
(From Walli's formula)
Ex.4 If un = then show that u1, u2, u3 ,...... constitute an arithmetic progression. Hence or otherwise find the value of un.
Sol.
un + 1 – 2un + un – 1 = (un + 1 – un) – (un – un – 1)
∴ un – 1 + un + 1 = 2un i.e., un – 1, un, un + 1 form an A.P.
⇒ u1, u2, u3,.........constitute an A.P.
Ex.5 Evaluate
Sol.
Integrating by parts taking unity as the second function, we have
Ex.6 Show that Hence or otherwise evaluate
Sol.
adding (1) and (2) then
Put a tan x = t ⇒ a sec2x dx = dt when x = 0 ⇒ t = 0 ; x = p/2 ⇒ t = ∝
Differentiating both side w.r.t. 'a', we get
again differentiating both sides w.r.t. 'a' we get
Put a = √5 on both sides, we get
Ex.7 Let f be an injective functions such that f(x) f(y) + 2 = f(x) + f(y) + f(xy) for all non negative real x and y with f(0) = 1 and f '(1) = 2 find f(x) and show that f(x) dx – x (f(x) + 2) is a constant.
Sol. We have f(x) f(y) + 2 = f(x) + f(y) + f(xy) ......(1)
Putting x = 1 and y = 1 then f(1) f(1) + 2 = 3f(1)
we get f(1) = 1, 2 & f(1) 1 (
f(0) = 1 & function is injective) then f(1) = 2
Replacing y by 1/x in (1) then f(x) f(1/x) + 2 = f(x) + f(1/x) + f(1) ⇒ f(x) f(1/x) = f(x) + f(1/x) [ f(1) = 2)
Hence f(x) is of the type f(x) = 1 ± xn ⇒ f(1) = 1 ± 1 = 2 (given)
∴ f(x) = 1 + xn and f '(x) = nxn – 1 ⇒ f '(1) = n = 2 ∴ f(x) = 1 + x2
∴
Ex.8 Evaluate
Sol.
Let I = Make |sin x| – |cos x| = 0 ∴ |tan x| = 1
∴ tan x = ± 1 and both these values lie in the interval [0, π].
We find for 0 < x < π/4, |sin x| – |cos x| < 0
|sin x| – |cos x| > 0
Ex.9 Evaluate , (where [ * ] is the greatest integer function)
Sol. Let I =
Let f(x) = x2 + x + 1 ⇒ f '(x) = 2x - 1 for x > 1/2, f '(x) > 0 and x < 12, f '(x) , 0
Values of f(x) at x = 1/2 and 2 are 3/4 and 3 integers between them an 1, 2 then x2 - x + 1 = 1, 2
we get x = 1, and values of f(x) at x = 0 and 1/2 are 1 and 3/4 no integer between them
Alternative Method : It is clear from the figure
Ex.10 If
Sol.
Integrating by parts taking x2 as 1 st function, we we get =
[By Prop.]
Adding (1) and (2) we get
Ex.11 Prove that
Sol.
Intergrating by parts taking x as a first function, we have
Ex.12 Evaluate
Sol.
Let g(t) = |t – 1| – |t| + |t + 1| =
=
Ex.13 For all positive integer k, prove that
Hence prove that
Sol. We have 2 sinx [cos x + cos 3x + ...+ cos (2k–1)x ]
= 2 sinx cosx + 2 sinx cos 3x + ....+ 2 sinx cos(2k – 1)x
= sin 2x + sin 4x – sin 2x + sin 6x – sin 4x + ....... + sin 2kx – sin (2k – 2) x
= sin 2kx
⇒ 2[cos x + cos 3x + ........ + cos (2k – 1) x ] = sin2kx/sinx
[2 cos2x + 2 cos 3x cos x + ....... + 2 cos (2k – 1 ) x cosx ] dx
[cosx + cos 3x + ....... + cos (2k – 1 ) x ]cosx dx
=
Ex.14 Let f(x) is periodic function such that Find the function f(x) if (1) = 1 .
Sol.
from (2) and (3)
Differentiating bot sides w.r.t.x, we get
then f(x) = 1/x3 But given f(x) is a periodic function Hence f(x) = 1
Ex.15 Assume then prove that
Sol.
Ex.16 Use induction to prove that ,
Sol.
Now sinkx = sin[(k + 1) x – x ] = sin(k + 1) x cos – cos(k + 1)x sin x
Hence sin (k + 1 ) x cosx = sinkx + cos(k + 1) x sin x
Subistuting P(k + 1) =
Now I. B. P. to get the result
|
172 videos|501 docs|154 tests
|
FAQs on Definite Integral As Limit Of A Sum And Estimate Of Definite Integrals - Mathematics (Maths) for JEE Main & Advanced
| 1. What is the concept of definite integral as a limit of a sum? |  |
| 2. How can we estimate definite integrals? |  |
| 3. Can definite integrals be used to find the value of a function at a specific point? |  |
| 4. What is a general inequality related to definite integrals? |  |
| 5. How are definite integrals used in real-world applications? |  |






















