Detailed Notes: Angle Modulation | Communication System - Electronics and Communication Engineering (ECE) PDF Download
Introduction
Angle modulation is the combination of frequency and phase modulation. We can also say that the frequency and phase combine to form an angle. Angle modulation is defined as the modulation where the frequency or phase of the carrier varies with the amplitude of the message signal. When the amplitude of the carrier varies with the message signal, it is known as amplitude modulation.
The spectral components of the modulated signal depend on the frequency and amplitude of the components in the baseband signal. Angle modulation is non-linear, while amplitude modulation is a linear process. The superposition principle does not apply in angle modulation.
The signal has the form of
V(t) = A cos [ωct + ϕ (t)]
Where,
ωc is the carrier frequency constant
A is the amplitude constant
ϕ (t) is the phase angle, which is not constant. It is a function of the baseband signal.
Types of Angle Modulation
Angle modulation is the combination of phase and frequency modulation. It is the modulation where both the frequency and phase of the carrier vary with the amplitude of the message signal, as discussed above.
Angle Modulation is categorized as frequency modulation and phase modulation.
- Frequency Modulation: If the frequency of the carrier varies with the amplitude of the message signal, it is known as frequency modulation. The amplitude of the modulated signal depends on the frequency difference between the carrier frequency and the center frequency. FM is a type of both analog and digital modulation. The applications of analog frequency modulation are telecommunications, computing, radio broadcasting, video broadcasting, and two-way radio systems. In digital modulation, the process used to transmit digital data using FM is known as Frequency-Shift Keying.
- Phase Modulation: If the carrier phase varies with the amplitude of the message signal, it is known as phase modulation. In angle modulation, it is together used with frequency modulation. It is also an integral part of both analog and digital communication. In analog communication, phase modulation is used for transmitting radio waves and other technologies, such as Wi-Fi and satellite television.
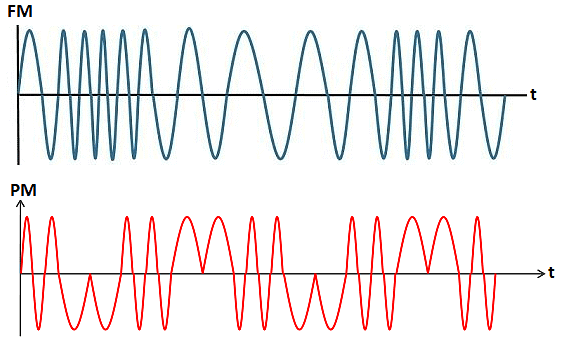
The waveforms of frequency modulation and phase modulation are shown below:
Relationship between Frequency and Phase Modulation
The block diagram of frequency modulation consists of an integrator and a phase modulator, as shown below: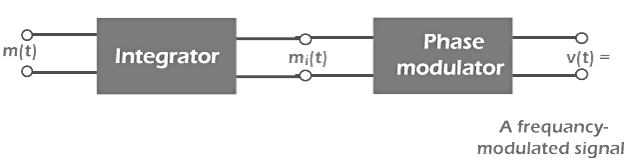
The modulating signal is applied to the integrator, which is further sent to the phase modulator. The output of the combination of the integrator and the phase modulator is the frequency modulated signal.
Let the message signal and the frequency constant be m(t) and KF.
Where,
KF = KKP
The output of the phase modulator is given by: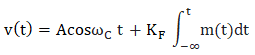 The block diagram of the phase modulation consists of a differentiator and a frequency modulator, as shown below:
The block diagram of the phase modulation consists of a differentiator and a frequency modulator, as shown below: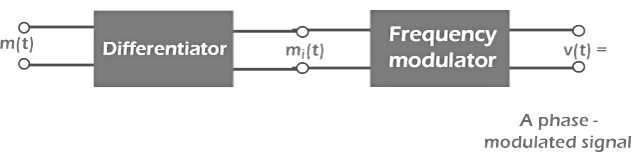 The modulating signal is applied to the differentiator, which is further sent to the frequency modulator. The output of the combination of the differentiator and frequency modulator is the phase modulated signal.
The modulating signal is applied to the differentiator, which is further sent to the frequency modulator. The output of the combination of the differentiator and frequency modulator is the phase modulated signal.
Let the phase constant be KP.
The output can be represented as:
V(t) = A cos [ωct + KP m(t)]
Where,
ωc is the carrier frequency constant
A is the amplitude constant
m(t) is the instantaneous value of the message signal
The instantaneous angular frequency is the differentiation of the output of the frequency modulator. It is given by: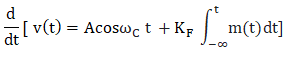 ω = ωc + K m(t)
ω = ωc + K m(t)
The maximum frequency component of the message signal and instantaneous value of the message signal is FM. The integral part of the frequency modulation is a linear operation and does not change the number of frequency components.
Modulation Index
The deviation of the total angle from the carrier angle is defined as phase deviation. The deviation of the total angle from the carrier angle is defined as phase deviation. The instantaneous frequency deviation from the carrier frequency is known as frequency deviation.
The frequency of the message signal is represented as ωm
ωm = 2πFm
The angle modulated signal is given by:
V(t) = A cos [ωct + Bsin ωmt] … (1)
Where,
ωc is the carrier frequency constant
A is the amplitude constant
ωc is the message frequency constant
B is the peak amplitude of the phase constant ϕ (t)
The instantaneous frequency is represented as ω.
ω = d/dt [ϕ]
ω = d/dt [[ωct + Bcosωmt]
ω = ωc + Bωm cos ωmt
We know,
ω = 2πF
Substituting the value of ω = 2πF, we get:
2πF = ωc + Bωm sin ωmt
F = ωc/2π + Bωm sin ωmt /2π
F = FC + BFm sin ωmt
Where,
FC = ωc/2π
Fm = ωm/2π
The maximum frequency deviation is represented as Δf.
Δf = BFm
Thus, equation (1) can be represented as:
V(t) = A cos [ωct + Δf / Fm sin ωmt]
In the next section, we will discuss FM (Frequency Modulation) and Phase Modulation (PM) in detail.
Numerical Examples
Let's discuss some numerical examples based on the angle modulation.
Example 1: Consider an angle modulated signal P(t) = 5cos [2π 106t + 3sin (2π 104t)]. Find its instantaneous value at t = 0.8 ms?
Given: Phase ϕ (t)= 2π 106t + 3sin (2π 104t)
Instantaneous frequency = dϕ (t)/dt
= d/dt [2π 106t + 3sin (2π 104t)]
= 2π 106 + 2π 104 3cos (2π 104t)
At, t = 0.8ms
t = 0.8 x 10-3 seconds
Substituting the value of t, we get:
= 2π 106 + 2π 104 3cos (2π 104 × 0.8 x 10-3)
= 2π 106 + 2π 104 3cos (16 π)
= 2π 106 + 2π 104 3 (1)
(cos16 π = 1)
= 2π 106 + 6π 104
= 2π 104 (102 + 3)
= 2π 104 (103)
= 6.47 ×106 Hz
= 6.47M Hz
Thus, the instantaneous value is 6.47M Hz.
Example 2: Consider an angle modulated signal P(t) = 3cos [2π 108t + 5sin (2π 105t)]. Find its instantaneous value at t = 0.5 ms?
Given: Phase ϕ (t)= 2π 108t + 5sin (2π 105t)
Instantaneous frequency = dϕ (t)/dt
= d/dt [2π 108t + 5sin (2π 105t)]
= 2π 108 + 2π 105 5cos (2π 105t)
At, t = 0.5ms
t = 0.5 x 10-3 seconds
Substituting the value of t, we get:
= 2π 108 + 2π 105 5cos (2π 104 × 0.5 x 10-3)
= 2π 108 + 2π 105 5cos (10 π)
= 2π 108 + 2π 105 5 (1)
(cos 10 π = 1)
= 2π 108 + 10π 105
= 2π 105 (103 + 5)
= 2π 104 (1005)
= 6.314 ×108 Hz
Thus, the instantaneous value is 6.314 ×108 Hz.
|
14 videos|38 docs|30 tests
|