Detailed Notes: Proportion | Mathematics & Pedagogy Paper 2 for CTET & TET Exams - CTET & State TET PDF Download
Introduction
- When two ratios are equal, the four quantities composing them are said to be proportional.
- Thus if a/b = c/d, then a, b, c, d are proportional. This is expressed by saying that a is to b as c is to d, and the proportion is written as a:b::c:d or a:b = c:d.

- The terms a and d are called the extremes while the terms b and c are called the means.
Thus, we can say:
Product of Extremes = Product of Means
Example: What is the value of x in the following expression?
5/8 = x/12
Solution:
⇒ 5/8 = x/12
⇒ x = 60/8 = 7.5
It can be calculated with the help of percentages also. In this question, the percentage increase in the denominator is 50%, so the numerator will also increase by 50%.
Operations in Proportions
If four quantities a, b, c and d form a proportion, many other proportions may be deduced by the properties of fractions. The results of these operations are very useful. These operations are:
- Invertendo: If a/b = c/d then b/a = d/c
- Alternando: If a/b = c/d, then a/c = b/d
- Componendo: If a/b=c/d, then (a+b)/b=(c+d)/d
- Dividendo: If a/b=c/d, then (a-b)/b=(c-d)/d
Componendo and Dividendo: If a/b=c/d then (a + b)/(a – b) = (c + d)/(c – d)
Variation
Two quantities A and B are said to vary with each other if there is some relationship between A and B such that the change in A and B is uniform and guided by some rule.
➢ Some Typical Examples of Variation
- Area (A) of a circle = π.R2, where R is the radius of the circle. Area of a circle depends upon the value of the radius of a circle, or, in other words, we can say that the area of a circle varies as the square of the radius of a circle.
- At a constant temperature, the pressure is inversely proportional to the volume.
- If the speed of any vehicle is constant, then the distance traversed is proportional to the time taken to cover the distance.
Essentially there are two kinds of proportions that two variables can be related by: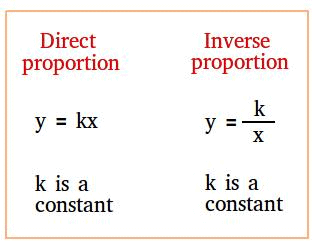
1. Direct Proportion
When it is said that A varies directly as B, you should understand the following implications:
(a) Logical Implication: When A increases B increases.
(b) Calculation Implication: If A increases by 10%, B will also increase by 10.
(c) Graphical Implications: The following graph is representative of this situation.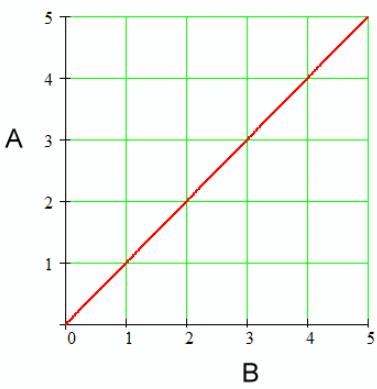
2. Inverse Proportion
When A varies inversely as B, the following implication arises.
(a) Logical Implication: When A increases B decreases.
(b) Calculation Implication: If A decreases by 9.09%, B will increase by 10%.
(c) Graphical Implications: The following graph is representative of this situation.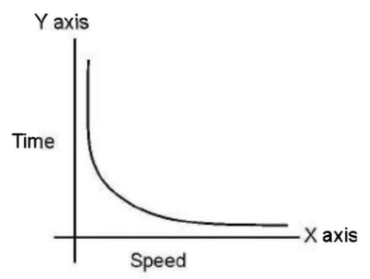
(d) Equation Implication: The product A X B is constant.
Example 1: The height of a tree varies as the square root of its age (between 5 and 17 years). When the age of a tree is 9 years, its height is 4 feet. What will be the height of the tree at the age of 16?
Solution: Let us assume the height of the tree is H and its age is A years.
► So, H ∝ √A, or, H = K x √A Now, 4 = K x √9 K = 4/3
► So, height at the age of 16 years = H = K x √A = 4/3 x 4 = 16/3 = 5 feet 4 inches.
Note: For problems based on this chapter we are always confronted with ratios and proportions between different number of variables. For the above problem we had three variables which were in the ratio of 4 : 6 : 9. When we have such a situation we normally assume the values in the same proportion, using one unknown ‘x’ only (in this example we could take the three values as 4x, 6x and 9x, respectively).Then, the total value is represented by the addition of the three giving rise to a linear equation, which on a solution, will result in the answer to the value of the unknown ‘x’.
- However, the student should realise that this unknown ‘x’ is not needed to solve the problem most of the time.
This is illustrated through the following alternative approach to solving the above problem:
► Assume the three values as 4, 6 and 9. Then we have:
► (4 + 28) + (6 + 37) + (9 + 18) = 5783
⇒ 19 = 5783–83 = 5700
⇒ 1 = 300
► Hence, 4 + 28 = 1228.
While adopting this approach, the student should be careful in being able to distinguish the numbers in bold as pointing out the unknown variable.
This document is the final document of EduRev’s notes of Ratios & proportions chapter. In the next document we have tried to solve the different CAT previous year questions & curated some of the best tests for you to practice - as a CAT aspirant you should be well aware of types of questions and should have a good practice of questions. Hence we suggests you to practice all the questions in the upcoming documents & Tests.
|
82 videos|300 docs|69 tests
|
FAQs on Detailed Notes: Proportion - Mathematics & Pedagogy Paper 2 for CTET & TET Exams - CTET & State TET
| 1. What are operations in proportions? |  |
| 2. How are proportions useful in real-life situations? |  |
| 3. What is variation in proportions? |  |
| 4. How can we solve problems involving proportions? |  |
| 5. Can proportions be used in scaling or resizing objects? |  |

















