Entropy as an exact differential | Additional Documents & Tests for IIT JAM PDF Download
This is one of four Maxwell relations (the others will follow shortly). They are all extremely useful in that the quantity on the right-hand side is virtually impossible to measure directly, while the quantity on the left-hand side is easily measured in the laboratory. For the present case one simply measures the adiabatic variation of temperature with volume in an insulated cylinder so that there is no heat flow (constant S).
The other three Maxwell relations follow by similarly considering the differential expressions for the thermodynamic potentials F(T, V), H(S, P), and G(T, P), with independent variables as indicated. The results are
Because the quantity dS = d′Qmax/T is an exact differential, many other important relationships connecting the thermodynamic properties of substances can be derived. For example, with the substitutions d′Q = T dSand d′W = P dV, the differential form (dU = d′Q − d′W) of the first law of thermodynamics becomes (for a single pure substance)
dU = T dS − P dV.
The advantage gained by the above formula is that dU is now expressed entirely in terms of state functions in place of the path-dependent quantities d′Q and d′W. This change has the very important mathematical implication that the appropriate independent variables are S and V in place of T and V, respectively, for internal energy.
This replacement of T by S as the most appropriate independent variable for the internal energy of substances is the single most valuable insight provided by the combined first and second laws of thermodynamics. With U regarded as a function U(S, V), its differential dU is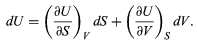
A comparison with the preceding equation shows immediately that the partial derivatives are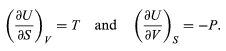
Furthermore, the cross partial derivatives,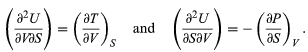
must be equal because the order of differentiation in calculating the second derivatives of U does not matter. Equating the right-hand sides of the above pair of equations then yields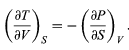
This is one of four Maxwell relations (the others will follow shortly). They are all extremely useful in that the quantity on the right-hand side is virtually impossible to measure directly, while the quantity on the left-hand side is easily measured in the laboratory. For the present case one simply measures the adiabatic variation of temperature with volume in an insulated cylinder so that there is no heat flow (constant S).
The other three Maxwell relations follow by similarly considering the differential expressions for the thermodynamic potentials F(T, V), H(S, P), and G(T, P), with independent variables as indicated. The results are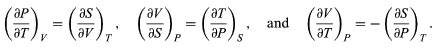
As an example of the use of these equations, equation (35) for CP − CVcontains the partial derivative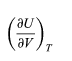
Which vanishes for an ideal gas and is difficult to evaluate directly from experimental data for real substances. The general properties of partial derivatives can first be used to write it in the form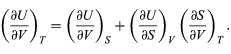
Combining this with equation for the partial derivatives together with the first of the Maxwell equations from equation then yields the desired result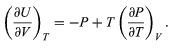
The quantity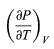
comes directly from differentiating the equation of state. For an ideal gas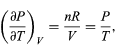
and so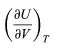
is zero as expected. The departure of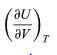
from zero reveals directly the effects of internal forces between the molecules of the substance and the work that must be done against them as the substance expands at constant temperature.

|
Explore Courses for IIT JAM exam
|

|

















